Question Number 119893 by peter frank last updated on 27/Oct/20

Answered by Ar Brandon last updated on 27/Oct/20
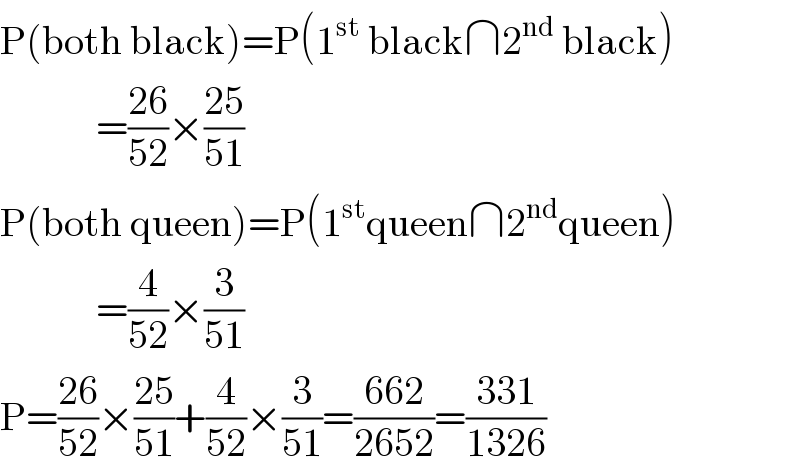
$$\mathrm{P}\left(\mathrm{both}\:\mathrm{black}\right)=\mathrm{P}\left(\mathrm{1}^{\mathrm{st}} \:\mathrm{black}\cap\mathrm{2}^{\mathrm{nd}} \:\mathrm{black}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{26}}{\mathrm{52}}×\frac{\mathrm{25}}{\mathrm{51}} \\ $$$$\mathrm{P}\left(\mathrm{both}\:\mathrm{queen}\right)=\mathrm{P}\left(\mathrm{1}^{\mathrm{st}} \mathrm{queen}\cap\mathrm{2}^{\mathrm{nd}} \mathrm{queen}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{4}}{\mathrm{52}}×\frac{\mathrm{3}}{\mathrm{51}} \\ $$$$\mathrm{P}=\frac{\mathrm{26}}{\mathrm{52}}×\frac{\mathrm{25}}{\mathrm{51}}+\frac{\mathrm{4}}{\mathrm{52}}×\frac{\mathrm{3}}{\mathrm{51}}=\frac{\mathrm{662}}{\mathrm{2652}}=\frac{\mathrm{331}}{\mathrm{1326}} \\ $$
Answered by Ar Brandon last updated on 27/Oct/20

$$\frac{\overset{\mathrm{26}} {\:}\mathrm{C}_{\mathrm{2}} }{\overset{\mathrm{52}} {\:}\mathrm{C}_{\mathrm{2}} }+\frac{\overset{\mathrm{4}} {\:}\mathrm{C}_{\mathrm{2}} }{\overset{\mathrm{52}} {\:}\mathrm{C}_{\mathrm{2}} }=\frac{\mathrm{331}}{\mathrm{1326}} \\ $$
Commented by peter frank last updated on 28/Oct/20

$$\mathrm{thank}\:\mathrm{you} \\ $$
