Question-120295

Question Number 120295 by A8;15: last updated on 30/Oct/20

Commented by TANMAY PANACEA last updated on 30/Oct/20

Answered by Dwaipayan Shikari last updated on 30/Oct/20

Σ_(n=1) ^n n^7 =(1/(24))n^2 (n+1)^2 (3n^4 +6n^3 −n^2 −4n+2) =(1/(24))(3n^8 +12n^7 +14n^6 −7n^4 +2n^2 ) Σ_(n=1) ^∞ (n^8 /(8n!))+Σ_(n=1) ^∞ (n^7 /(2n!))+Σ_(n=1) ^∞ ((7n^6 )/(12n!))−(7/(24))Σ_(n=1) ^∞ (n^4 /(n!))+Σ_(n=1) ^∞ (n^2 /(12n!)) =((4140)/8)e+((877)/2)e+((1421)/(12))e−((105)/(24))e+(1/6)e

$$\underset{{n}=\mathrm{1}} {\overset{{n}} {\sum}}{n}^{\mathrm{7}} =\frac{\mathrm{1}}{\mathrm{24}}{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{3}{n}^{\mathrm{4}} +\mathrm{6}{n}^{\mathrm{3}} −{n}^{\mathrm{2}} −\mathrm{4}{n}+\mathrm{2}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{24}}\left(\mathrm{3}{n}^{\mathrm{8}} +\mathrm{12}{n}^{\mathrm{7}} +\mathrm{14}{n}^{\mathrm{6}} −\mathrm{7}{n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{2}} \right) \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{8}} }{\mathrm{8}{n}!}+\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{7}} }{\mathrm{2}{n}!}+\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{7}{n}^{\mathrm{6}} }{\mathrm{12}{n}!}−\frac{\mathrm{7}}{\mathrm{24}}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{4}} }{{n}!}+\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{2}} }{\mathrm{12}{n}!} \\ $$$$=\frac{\mathrm{4140}}{\mathrm{8}}{e}+\frac{\mathrm{877}}{\mathrm{2}}{e}+\frac{\mathrm{1421}}{\mathrm{12}}{e}−\frac{\mathrm{105}}{\mathrm{24}}{e}+\frac{\mathrm{1}}{\mathrm{6}}{e} \\ $$

Commented by A8;15: last updated on 30/Oct/20

what about factorials, (n+1)⁷/n! ?

Commented by A8;15: last updated on 30/Oct/20

Sorry 1⁷/1! or (1⁷+2⁷)/2! or (1⁷+2⁷+3⁷)/3! ?

Commented by Dwaipayan Shikari last updated on 30/Oct/20

T_n =((Σ_(n=1) ^n n^7 )/(n!)) And Sum is Σ_(n=1) ^∞ (((3n^8 +12n^7 +14n^6 −7n^4 +2n^2 ))/(24n!)) Which is the above result

$${T}_{{n}} =\frac{\underset{{n}=\mathrm{1}} {\overset{{n}} {\sum}}{n}^{\mathrm{7}} }{{n}!} \\ $$$${And}\:{Sum}\:{is} \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{3}{n}^{\mathrm{8}} +\mathrm{12}{n}^{\mathrm{7}} +\mathrm{14}{n}^{\mathrm{6}} −\mathrm{7}{n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{2}} \right)}{\mathrm{24}{n}!}\:{Which}\:{is}\:{the}\:{above}\:{result} \\ $$

Commented by Dwaipayan Shikari last updated on 30/Oct/20

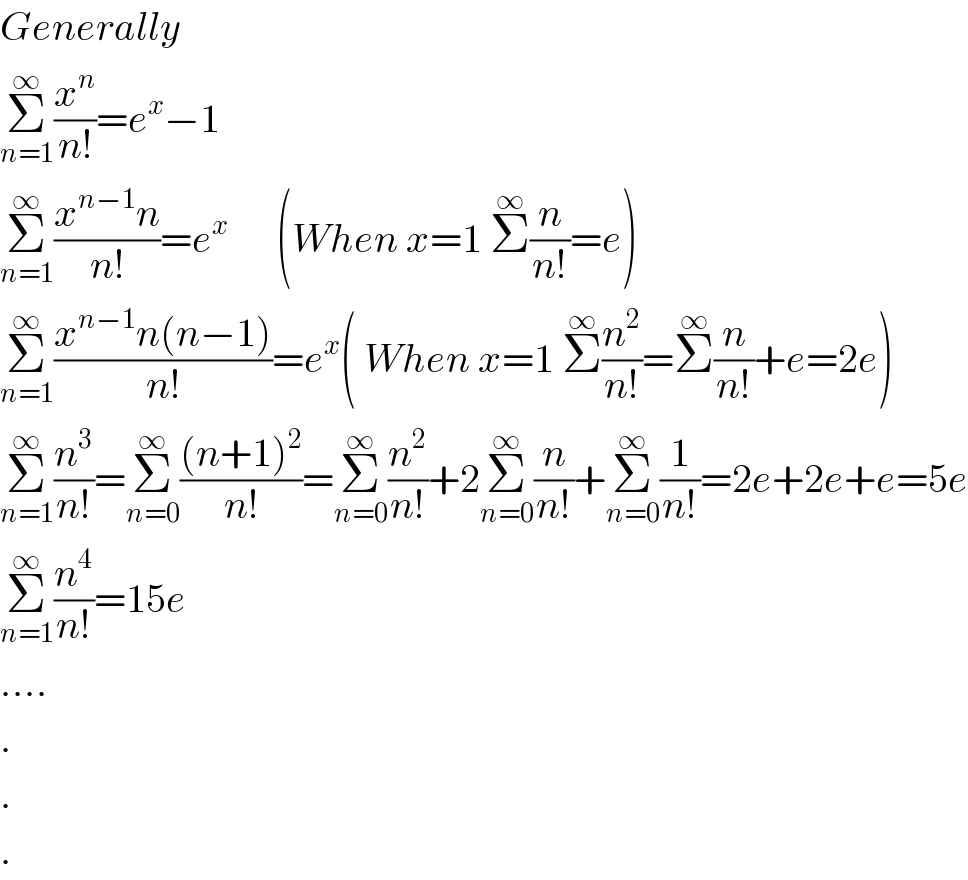
Generally Σ_(n=1) ^∞ (x^n /(n!))=e^x −1 Σ_(n=1) ^∞ ((x^(n−1) n)/(n!))=e^(x ) (When x=1 Σ^∞ (n/(n!))=e) Σ_(n=1) ^∞ ((x^(n−1) n(n−1))/(n!))=e^x ( When x=1 Σ^∞ (n^2 /(n!))=Σ^∞ (n/(n!))+e=2e) Σ_(n=1) ^∞ (n^3 /(n!))=Σ_(n=0) ^∞ (((n+1)^2 )/(n!))=Σ_(n=0) ^∞ (n^2 /(n!))+2Σ_(n=0) ^∞ (n/(n!))+Σ_(n=0) ^∞ (1/(n!))=2e+2e+e=5e Σ_(n=1) ^∞ (n^4 /(n!))=15e .... . . .

$${Generally} \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{x}^{{n}} }{{n}!}={e}^{{x}} −\mathrm{1} \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{x}^{{n}−\mathrm{1}} {n}}{{n}!}={e}^{{x}\:\:} \:\:\:\:\:\left({When}\:{x}=\mathrm{1}\:\overset{\infty} {\sum}\frac{{n}}{{n}!}={e}\right) \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{x}^{{n}−\mathrm{1}} {n}\left({n}−\mathrm{1}\right)}{{n}!}={e}^{{x}} \left(\:{When}\:{x}=\mathrm{1}\:\overset{\infty} {\sum}\frac{{n}^{\mathrm{2}} }{{n}!}=\overset{\infty} {\sum}\frac{{n}}{{n}!}+{e}=\mathrm{2}{e}\right) \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{3}} }{{n}!}=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }{{n}!}=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{2}} }{{n}!}+\mathrm{2}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{n}}{{n}!}+\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}!}=\mathrm{2}{e}+\mathrm{2}{e}+{e}=\mathrm{5}{e} \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{4}} }{{n}!}=\mathrm{15}{e} \\ $$$$…. \\ $$$$. \\ $$$$. \\ $$$$. \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply