Menu Close

Question-120549

Tinku Tara 0 Comments
Pin




Question Number 120549 by bobhans last updated on 01/Nov/20
Answered by TANMAY PANACEA last updated on 01/Nov/20
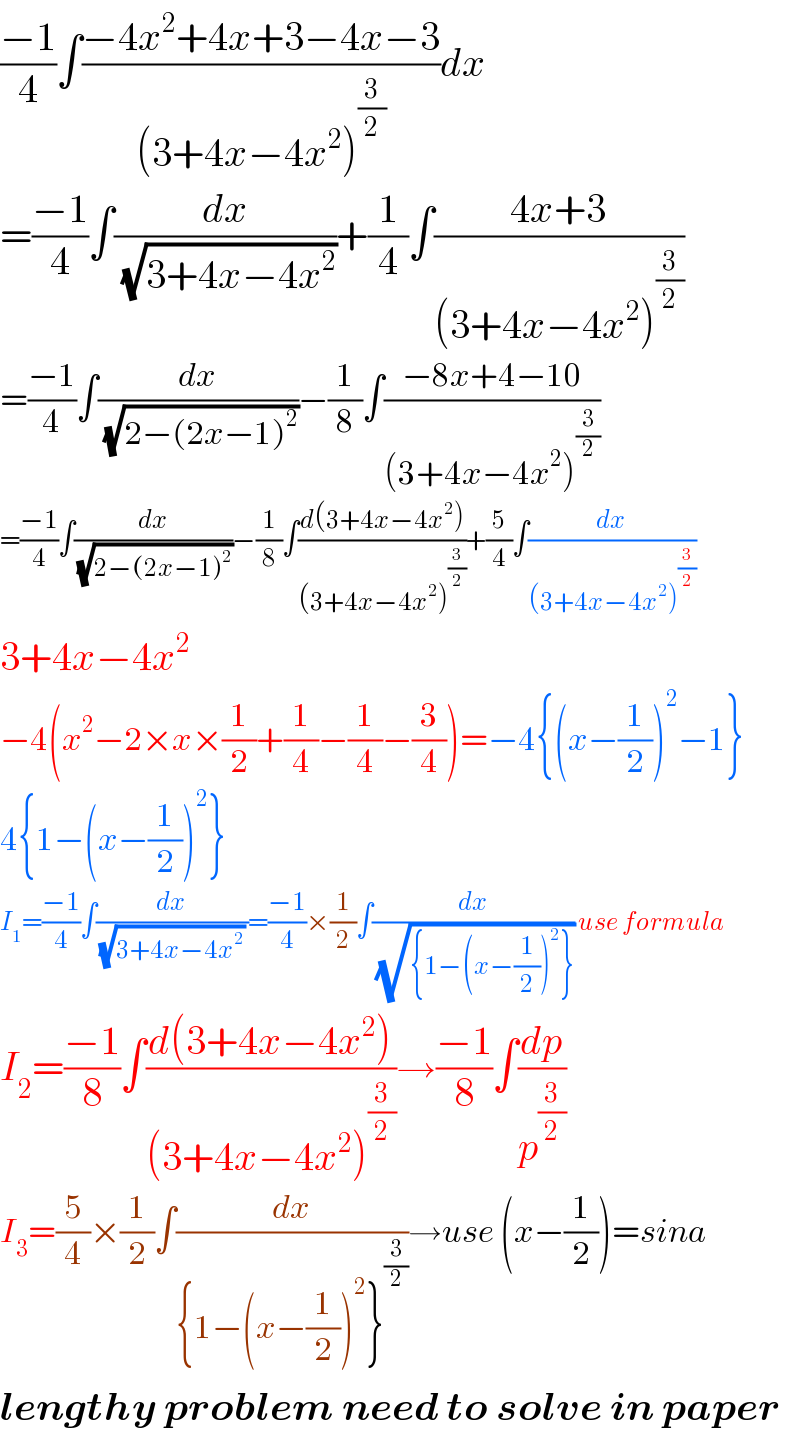
((−1)/4)∫((−4x^2 +4x+3−4x−3)/((3+4x−4x^2 )^(3/2) ))dx =((−1)/4)∫(dx/( (√(3+4x−4x^2 ))))+(1/4)∫((4x+3)/((3+4x−4x^2 )^(3/2) )) =((−1)/4)∫(dx/( (√(2−(2x−1)^2 ))))−(1/8)∫((−8x+4−10)/((3+4x−4x^2 )^(3/2) )) =((−1)/4)∫(dx/( (√(2−(2x−1)^2 ))))−(1/8)∫((d(3+4x−4x^2 ))/((3+4x−4x^2 )^(3/2) ))+(5/4)∫(dx/((3+4x−4x^2 )^(3/2) )) 3+4x−4x^2 −4(x^2 −2×x×(1/2)+(1/4)−(1/4)−(3/4))=−4{(x−(1/2))^2 −1} 4{1−(x−(1/2))^2 } I_1 =((−1)/4)∫(dx/( (√(3+4x−4x^2 )) ))=((−1)/4)×(1/2)∫(dx/( (√({1−(x−(1/2))^2 })))) use formula I_2 =((−1)/8)∫((d(3+4x−4x^2 ))/((3+4x−4x^2 )^(3/2) ))→((−1)/8)∫(dp/p^(3/2) ) I_3 =(5/4)×(1/2)∫(dx/({1−(x−(1/2))^2 }^(3/2) ))→use (x−(1/2))=sina lengthy problem need to solve in paper
$$\frac{−\mathrm{1}}{\mathrm{4}}\int\frac{−\mathrm{4}{x}^{\mathrm{2}} +\mathrm{4}{x}+\mathrm{3}−\mathrm{4}{x}−\mathrm{3}}{\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }{dx} \\ $$$$=\frac{−\mathrm{1}}{\mathrm{4}}\int\frac{{dx}}{\:\sqrt{\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} }}+\frac{\mathrm{1}}{\mathrm{4}}\int\frac{\mathrm{4}{x}+\mathrm{3}}{\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} } \\ $$$$=\frac{−\mathrm{1}}{\mathrm{4}}\int\frac{{dx}}{\:\sqrt{\mathrm{2}−\left(\mathrm{2}{x}−\mathrm{1}\right)^{\mathrm{2}} }}−\frac{\mathrm{1}}{\mathrm{8}}\int\frac{−\mathrm{8}{x}+\mathrm{4}−\mathrm{10}}{\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} } \\ $$$$=\frac{−\mathrm{1}}{\mathrm{4}}\int\frac{{dx}}{\:\sqrt{\mathrm{2}−\left(\mathrm{2}{x}−\mathrm{1}\right)^{\mathrm{2}} }}−\frac{\mathrm{1}}{\mathrm{8}}\int\frac{{d}\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)}{\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }+\frac{\mathrm{5}}{\mathrm{4}}\int\frac{{dx}}{\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} } \\ $$$$\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \\ $$$$−\mathrm{4}\left({x}^{\mathrm{2}} −\mathrm{2}×{x}×\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{3}}{\mathrm{4}}\right)=−\mathrm{4}\left\{\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} −\mathrm{1}\right\} \\ $$$$\mathrm{4}\left\{\mathrm{1}−\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \right\} \\ $$$${I}_{\mathrm{1}} =\frac{−\mathrm{1}}{\mathrm{4}}\int\frac{{dx}}{\:\sqrt{\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} }\:}=\frac{−\mathrm{1}}{\mathrm{4}}×\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dx}}{\:\sqrt{\left\{\mathrm{1}−\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \right\}}}\:{use}\:{formula} \\ $$$${I}_{\mathrm{2}} =\frac{−\mathrm{1}}{\mathrm{8}}\int\frac{{d}\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)}{\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\rightarrow\frac{−\mathrm{1}}{\mathrm{8}}\int\frac{{dp}}{{p}^{\frac{\mathrm{3}}{\mathrm{2}}} } \\ $$$${I}_{\mathrm{3}} =\frac{\mathrm{5}}{\mathrm{4}}×\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dx}}{\left\{\mathrm{1}−\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \right\}^{\frac{\mathrm{3}}{\mathrm{2}}} }\rightarrow{use}\:\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)={sina} \\ $$$$\boldsymbol{{lengthy}}\:\boldsymbol{{problem}}\:\boldsymbol{{need}}\:\boldsymbol{{to}}\:\boldsymbol{{solve}}\:\boldsymbol{{in}}\:\boldsymbol{{paper}} \\ $$
Answered by john santu last updated on 01/Nov/20
∫ ((x^2 dx)/((4−(1−2x)^2 )^(3/2) )) ; [ set 1−2x = 2 sin t or sin t = ((1−2x)/2) ] ∫ (((((1−2sin t)/2))^2 (−cos t dt ))/((4−4sin^2 t)^(3/2) )) = −(1/(32))∫ (((1−2sin t)^2 cos t dt)/(cos^3 t)) = −(1/(32)) ∫ ((1−4sin t+4sin^2 t)/(cos^2 t)) dt = −(1/(32)) ∫ (sec^2 t−4((sin t)/(cos^2 t))+4 tan^2 t) dt = −(1/(32)) [ ∫(5sec^2 t−4)dt+4∫ ((d(cos t))/(cos^2 t)) ]= −(1/(32)) [ 5tan t−4t−(4/(cos t)) ] + c = −((5(1−2x))/(32(√(3+4x−4x^2 ))))+(1/8)arc sin (((1−2x)/2))+(1/( 16(√(3+4x−4x^2 )))) + c [✓]
$$\int\:\frac{{x}^{\mathrm{2}} \:{dx}}{\left(\mathrm{4}−\left(\mathrm{1}−\mathrm{2}{x}\right)^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\:;\:\left[\:{set}\:\mathrm{1}−\mathrm{2}{x}\:=\:\mathrm{2}\:\mathrm{sin}\:{t}\:{or}\:\mathrm{sin}\:{t}\:=\:\frac{\mathrm{1}−\mathrm{2}{x}}{\mathrm{2}}\:\right] \\ $$$$\int\:\frac{\left(\frac{\mathrm{1}−\mathrm{2sin}\:{t}}{\mathrm{2}}\right)^{\mathrm{2}} \left(−\mathrm{cos}\:{t}\:{dt}\:\right)}{\left(\mathrm{4}−\mathrm{4sin}\:^{\mathrm{2}} {t}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\:= \\ $$$$−\frac{\mathrm{1}}{\mathrm{32}}\int\:\frac{\left(\mathrm{1}−\mathrm{2sin}\:{t}\right)^{\mathrm{2}} \:\mathrm{cos}\:{t}\:{dt}}{\mathrm{cos}\:^{\mathrm{3}} {t}}\:=\: \\ $$$$−\frac{\mathrm{1}}{\mathrm{32}}\:\int\:\frac{\mathrm{1}−\mathrm{4sin}\:{t}+\mathrm{4sin}\:^{\mathrm{2}} {t}}{\mathrm{cos}\:^{\mathrm{2}} {t}}\:{dt}\:= \\ $$$$−\frac{\mathrm{1}}{\mathrm{32}}\:\int\:\left(\mathrm{sec}\:^{\mathrm{2}} {t}−\mathrm{4}\frac{\mathrm{sin}\:{t}}{\mathrm{cos}\:^{\mathrm{2}} {t}}+\mathrm{4}\:\mathrm{tan}\:^{\mathrm{2}} {t}\right)\:{dt}\:= \\ $$$$−\frac{\mathrm{1}}{\mathrm{32}}\:\left[\:\int\left(\mathrm{5sec}\:^{\mathrm{2}} {t}−\mathrm{4}\right){dt}+\mathrm{4}\int\:\frac{{d}\left(\mathrm{cos}\:{t}\right)}{\mathrm{cos}\:^{\mathrm{2}} {t}}\:\right]= \\ $$$$−\frac{\mathrm{1}}{\mathrm{32}}\:\left[\:\mathrm{5tan}\:{t}−\mathrm{4}{t}−\frac{\mathrm{4}}{\mathrm{cos}\:{t}}\:\right]\:+\:{c}\:= \\ $$$$−\frac{\mathrm{5}\left(\mathrm{1}−\mathrm{2}{x}\right)}{\mathrm{32}\sqrt{\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} }}+\frac{\mathrm{1}}{\mathrm{8}}\mathrm{arc}\:\mathrm{sin}\:\left(\frac{\mathrm{1}−\mathrm{2}{x}}{\mathrm{2}}\right)+\frac{\mathrm{1}}{\:\mathrm{16}\sqrt{\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} }}\:+\:{c}\:\left[\checkmark\right] \\ $$
Answered by MJS_new last updated on 01/Nov/20
∫(x^2 /((3+4x−4x^2 )^(3/2) ))dx= [t=x−(1/2) → dx=dt] =(1/(32))∫(((2t+1)^2 )/( (1−t^2 )^(3/2) ))dt= [u=arcsin t → dt=(√(1−t^2 ))du] =(1/(32))∫(((1+2sin u)^2 )/(cos^2 u))du= [v=tan u → du=cos^2 u dv] =(1/(32))∫(((2v+(√(v^2 +1)))^2 )/(v^2 +1))dv= =(5/(32))∫dv−(1/8)∫(dv/(v^2 +1))+(1/8)∫(v/( (√(v^2 +1))))dv= =(5/(32))v−(1/8)arctan v +(1/8)(√(v^2 +1))=... [v=tan (u/2) → du=(2/(v^2 +1))dv] =(1/(16))∫(((v^2 +4v+1)^2 )/((v−1)^2 (v+1)^2 (v^2 +1)))dv= =(9/(32))∫(dv/((v−1)^2 ))+(1/(32))∫(dv/((v+1)^2 ))−(1/4)∫(dv/((v^2 +1)))= =−(9/(32(v−1)))−(1/(32(v+1)))−(1/4)arctan v =...
$$\int\frac{{x}^{\mathrm{2}} }{\left(\mathrm{3}+\mathrm{4}{x}−\mathrm{4}{x}^{\mathrm{2}} \right)^{\mathrm{3}/\mathrm{2}} }{dx}= \\ $$$$\:\:\:\:\:\left[{t}={x}−\frac{\mathrm{1}}{\mathrm{2}}\:\rightarrow\:{dx}={dt}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{32}}\int\frac{\left(\mathrm{2}{t}+\mathrm{1}\right)^{\mathrm{2}} }{\:\left(\mathrm{1}−{t}^{\mathrm{2}} \right)^{\mathrm{3}/\mathrm{2}} }{dt}= \\ $$$$\:\:\:\:\:\left[{u}=\mathrm{arcsin}\:{t}\:\rightarrow\:{dt}=\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }{du}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{32}}\int\frac{\left(\mathrm{1}+\mathrm{2sin}\:{u}\right)^{\mathrm{2}} }{\mathrm{cos}^{\mathrm{2}} \:{u}}{du}= \\ $$$$\:\:\:\:\:\left[{v}=\mathrm{tan}\:{u}\:\rightarrow\:{du}=\mathrm{cos}^{\mathrm{2}} \:{u}\:{dv}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{32}}\int\frac{\left(\mathrm{2}{v}+\sqrt{{v}^{\mathrm{2}} +\mathrm{1}}\right)^{\mathrm{2}} }{{v}^{\mathrm{2}} +\mathrm{1}}{dv}= \\ $$$$=\frac{\mathrm{5}}{\mathrm{32}}\int{dv}−\frac{\mathrm{1}}{\mathrm{8}}\int\frac{{dv}}{{v}^{\mathrm{2}} +\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{8}}\int\frac{{v}}{\:\sqrt{{v}^{\mathrm{2}} +\mathrm{1}}}{dv}= \\ $$$$=\frac{\mathrm{5}}{\mathrm{32}}{v}−\frac{\mathrm{1}}{\mathrm{8}}\mathrm{arctan}\:{v}\:+\frac{\mathrm{1}}{\mathrm{8}}\sqrt{{v}^{\mathrm{2}} +\mathrm{1}}=… \\ $$$$\:\:\:\:\:\left[{v}=\mathrm{tan}\:\frac{{u}}{\mathrm{2}}\:\rightarrow\:{du}=\frac{\mathrm{2}}{{v}^{\mathrm{2}} +\mathrm{1}}{dv}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{16}}\int\frac{\left({v}^{\mathrm{2}} +\mathrm{4}{v}+\mathrm{1}\right)^{\mathrm{2}} }{\left({v}−\mathrm{1}\right)^{\mathrm{2}} \left({v}+\mathrm{1}\right)^{\mathrm{2}} \left({v}^{\mathrm{2}} +\mathrm{1}\right)}{dv}= \\ $$$$=\frac{\mathrm{9}}{\mathrm{32}}\int\frac{{dv}}{\left({v}−\mathrm{1}\right)^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{32}}\int\frac{{dv}}{\left({v}+\mathrm{1}\right)^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{4}}\int\frac{{dv}}{\left({v}^{\mathrm{2}} +\mathrm{1}\right)}= \\ $$$$=−\frac{\mathrm{9}}{\mathrm{32}\left({v}−\mathrm{1}\right)}−\frac{\mathrm{1}}{\mathrm{32}\left({v}+\mathrm{1}\right)}−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{arctan}\:{v}\:=… \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *