Question Number 120558 by peter frank last updated on 01/Nov/20
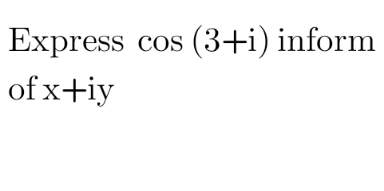
Answered by mr W last updated on 01/Nov/20
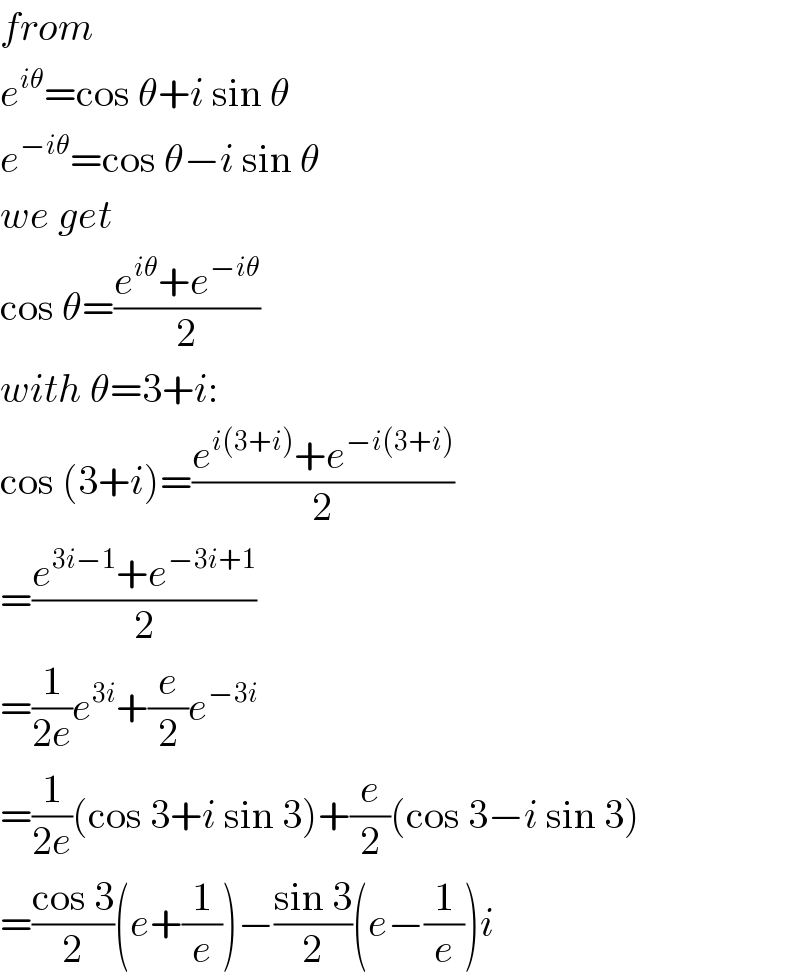
$${from} \\ $$$${e}^{{i}\theta} =\mathrm{cos}\:\theta+{i}\:\mathrm{sin}\:\theta \\ $$$${e}^{−{i}\theta} =\mathrm{cos}\:\theta−{i}\:\mathrm{sin}\:\theta \\ $$$${we}\:{get} \\ $$$$\mathrm{cos}\:\theta=\frac{{e}^{{i}\theta} +{e}^{−{i}\theta} }{\mathrm{2}} \\ $$$${with}\:\theta=\mathrm{3}+{i}: \\ $$$$\mathrm{cos}\:\left(\mathrm{3}+{i}\right)=\frac{{e}^{{i}\left(\mathrm{3}+{i}\right)} +{e}^{−{i}\left(\mathrm{3}+{i}\right)} }{\mathrm{2}} \\ $$$$=\frac{{e}^{\mathrm{3}{i}−\mathrm{1}} +{e}^{−\mathrm{3}{i}+\mathrm{1}} }{\mathrm{2}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}{e}}{e}^{\mathrm{3}{i}} +\frac{{e}}{\mathrm{2}}{e}^{−\mathrm{3}{i}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}{e}}\left(\mathrm{cos}\:\mathrm{3}+{i}\:\mathrm{sin}\:\mathrm{3}\right)+\frac{{e}}{\mathrm{2}}\left(\mathrm{cos}\:\mathrm{3}−{i}\:\mathrm{sin}\:\mathrm{3}\right) \\ $$$$=\frac{\mathrm{cos}\:\mathrm{3}}{\mathrm{2}}\left({e}+\frac{\mathrm{1}}{{e}}\right)−\frac{\mathrm{sin}\:\mathrm{3}}{\mathrm{2}}\left({e}−\frac{\mathrm{1}}{{e}}\right){i} \\ $$
Commented by peter frank last updated on 01/Nov/20

$$\mathrm{thank}\:\mathrm{you} \\ $$
Answered by TANMAY PANACEA last updated on 01/Nov/20

$${cos}\mathrm{3}{cosi}−{sin}\mathrm{3}{sini} \\ $$$${cos}\mathrm{3}{cosh}−{sin}\mathrm{3}×{isinh} \\ $$
Answered by mathmax by abdo last updated on 01/Nov/20

$$\mathrm{cos}\left(\mathrm{3}+\mathrm{i}\right)\:=\frac{\mathrm{e}^{\mathrm{i}\left(\mathrm{3}+\mathrm{i}\right)} +\mathrm{e}^{−\mathrm{i}\left(\mathrm{3}+\mathrm{i}\right)} }{\mathrm{2}}\:=\frac{\mathrm{e}^{\mathrm{3i}−\mathrm{1}} \:+\mathrm{e}^{−\mathrm{3i}+\mathrm{1}} }{\mathrm{2}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left\{\mathrm{e}^{−\mathrm{1}} \left(\mathrm{cos}\left(\mathrm{3}\right)+\mathrm{isin3}\right)+\mathrm{e}\left(\mathrm{cos3}−\mathrm{isin3}\right)\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left\{\left(\mathrm{e}+\mathrm{e}^{−\mathrm{1}} \right)\mathrm{cos3}\:+\mathrm{isin3}\left(\mathrm{e}^{−\mathrm{1}} −\mathrm{e}\right)\right\} \\ $$$$=\frac{\mathrm{e}+\mathrm{e}^{−\mathrm{1}} }{\mathrm{2}}\mathrm{cos}\left(\mathrm{3}\right)−\mathrm{isin}\left(\mathrm{3}\right)\left(\frac{\mathrm{e}−\mathrm{e}^{−\mathrm{1}} }{\mathrm{2}}\right)\:=\mathrm{ch}\left(\mathrm{1}\right)\mathrm{cos}\left(\mathrm{3}\right)−\mathrm{ish}\left(\mathrm{1}\right)\mathrm{sin3} \\ $$
Commented by peter frank last updated on 01/Nov/20

$$\mathrm{thank}\:\:\mathrm{you} \\ $$
Commented by mathmax by abdo last updated on 08/Nov/20

$$\mathrm{you}\:\mathrm{are}\:\mathrm{welcome} \\ $$
