Question Number 120560 by peter frank last updated on 01/Nov/20

Answered by mathmax by abdo last updated on 01/Nov/20
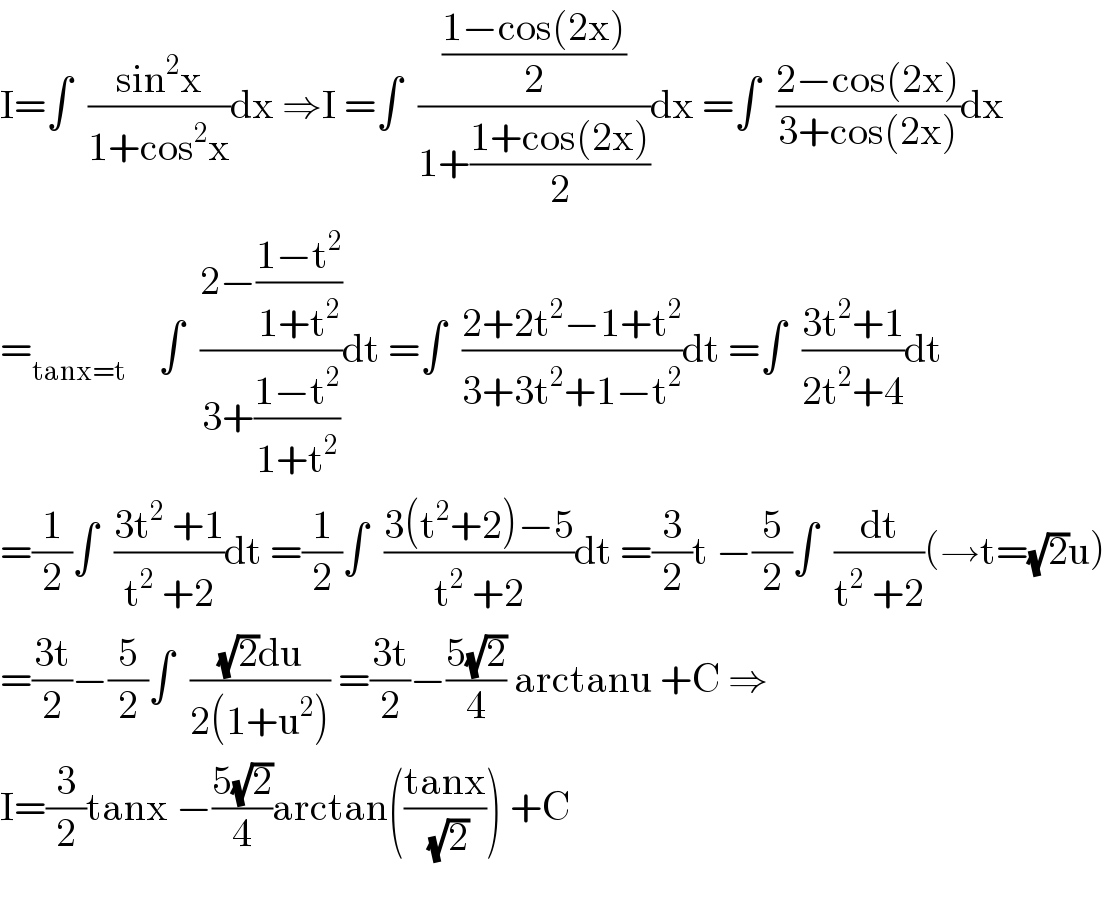
$$\mathrm{I}=\int\:\:\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx}\:\Rightarrow\mathrm{I}\:=\int\:\:\frac{\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{2}}}{\mathrm{1}+\frac{\mathrm{1}+\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{2}}}\mathrm{dx}\:=\int\:\:\frac{\mathrm{2}−\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{3}+\mathrm{cos}\left(\mathrm{2x}\right)}\mathrm{dx} \\ $$$$=_{\mathrm{tanx}=\mathrm{t}} \:\:\:\:\int\:\:\frac{\mathrm{2}−\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }}{\mathrm{3}+\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }}\mathrm{dt}\:=\int\:\:\frac{\mathrm{2}+\mathrm{2t}^{\mathrm{2}} −\mathrm{1}+\mathrm{t}^{\mathrm{2}} }{\mathrm{3}+\mathrm{3t}^{\mathrm{2}} +\mathrm{1}−\mathrm{t}^{\mathrm{2}} }\mathrm{dt}\:=\int\:\:\frac{\mathrm{3t}^{\mathrm{2}} +\mathrm{1}}{\mathrm{2t}^{\mathrm{2}} +\mathrm{4}}\mathrm{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\:\:\frac{\mathrm{3t}^{\mathrm{2}} \:+\mathrm{1}}{\mathrm{t}^{\mathrm{2}} \:+\mathrm{2}}\mathrm{dt}\:=\frac{\mathrm{1}}{\mathrm{2}}\int\:\:\frac{\mathrm{3}\left(\mathrm{t}^{\mathrm{2}} +\mathrm{2}\right)−\mathrm{5}}{\mathrm{t}^{\mathrm{2}} \:+\mathrm{2}}\mathrm{dt}\:=\frac{\mathrm{3}}{\mathrm{2}}\mathrm{t}\:−\frac{\mathrm{5}}{\mathrm{2}}\int\:\:\frac{\mathrm{dt}}{\mathrm{t}^{\mathrm{2}} \:+\mathrm{2}}\left(\rightarrow\mathrm{t}=\sqrt{\mathrm{2}}\mathrm{u}\right) \\ $$$$=\frac{\mathrm{3t}}{\mathrm{2}}−\frac{\mathrm{5}}{\mathrm{2}}\int\:\:\frac{\sqrt{\mathrm{2}}\mathrm{du}}{\mathrm{2}\left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)}\:=\frac{\mathrm{3t}}{\mathrm{2}}−\frac{\mathrm{5}\sqrt{\mathrm{2}}}{\mathrm{4}}\:\mathrm{arctanu}\:+\mathrm{C}\:\Rightarrow \\ $$$$\mathrm{I}=\frac{\mathrm{3}}{\mathrm{2}}\mathrm{tanx}\:−\frac{\mathrm{5}\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{arctan}\left(\frac{\mathrm{tanx}}{\:\sqrt{\mathrm{2}}}\right)\:+\mathrm{C} \\ $$$$ \\ $$
Commented by peter frank last updated on 01/Nov/20

$$\mathrm{thank}\:\mathrm{you} \\ $$
Answered by MJS_new last updated on 01/Nov/20
![∫((sin^2 x)/(1+cos^2 x))dx=∫((2/(1+cos^2 x))−1)dx 2∫(dx/(1+cos^2 x))= [t=tan x → dx=cos^2 x dx] =2∫(dt/(t^2 +2))=(√2)arctan (((√2)t)/2) = =(√2)arctan (((√2)tan x)/2) ∫((sin^2 x)/(1+cos^2 x))dx=−x+(√2)arctan (((√2)tan x)/2) +C](https://www.tinkutara.com/question/Q120606.png)
$$\int\frac{\mathrm{sin}^{\mathrm{2}} \:{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \:{x}}{dx}=\int\left(\frac{\mathrm{2}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \:{x}}−\mathrm{1}\right){dx} \\ $$$$\mathrm{2}\int\frac{{dx}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \:{x}}= \\ $$$$\:\:\:\:\:\left[{t}=\mathrm{tan}\:{x}\:\rightarrow\:{dx}=\mathrm{cos}^{\mathrm{2}} \:{x}\:{dx}\right] \\ $$$$=\mathrm{2}\int\frac{{dt}}{{t}^{\mathrm{2}} +\mathrm{2}}=\sqrt{\mathrm{2}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{2}}{t}}{\mathrm{2}}\:= \\ $$$$=\sqrt{\mathrm{2}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{2}}\mathrm{tan}\:{x}}{\mathrm{2}} \\ $$$$\int\frac{\mathrm{sin}^{\mathrm{2}} \:{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \:{x}}{dx}=−{x}+\sqrt{\mathrm{2}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{2}}\mathrm{tan}\:{x}}{\mathrm{2}}\:+{C} \\ $$
Commented by peter frank last updated on 01/Nov/20

$$\mathrm{thank}\:\mathrm{you} \\ $$
