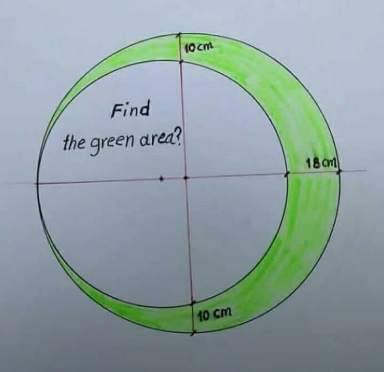
Question Number 120790 by peter frank last updated on 02/Nov/20
Commented by TANMAY PANACEA last updated on 02/Nov/20
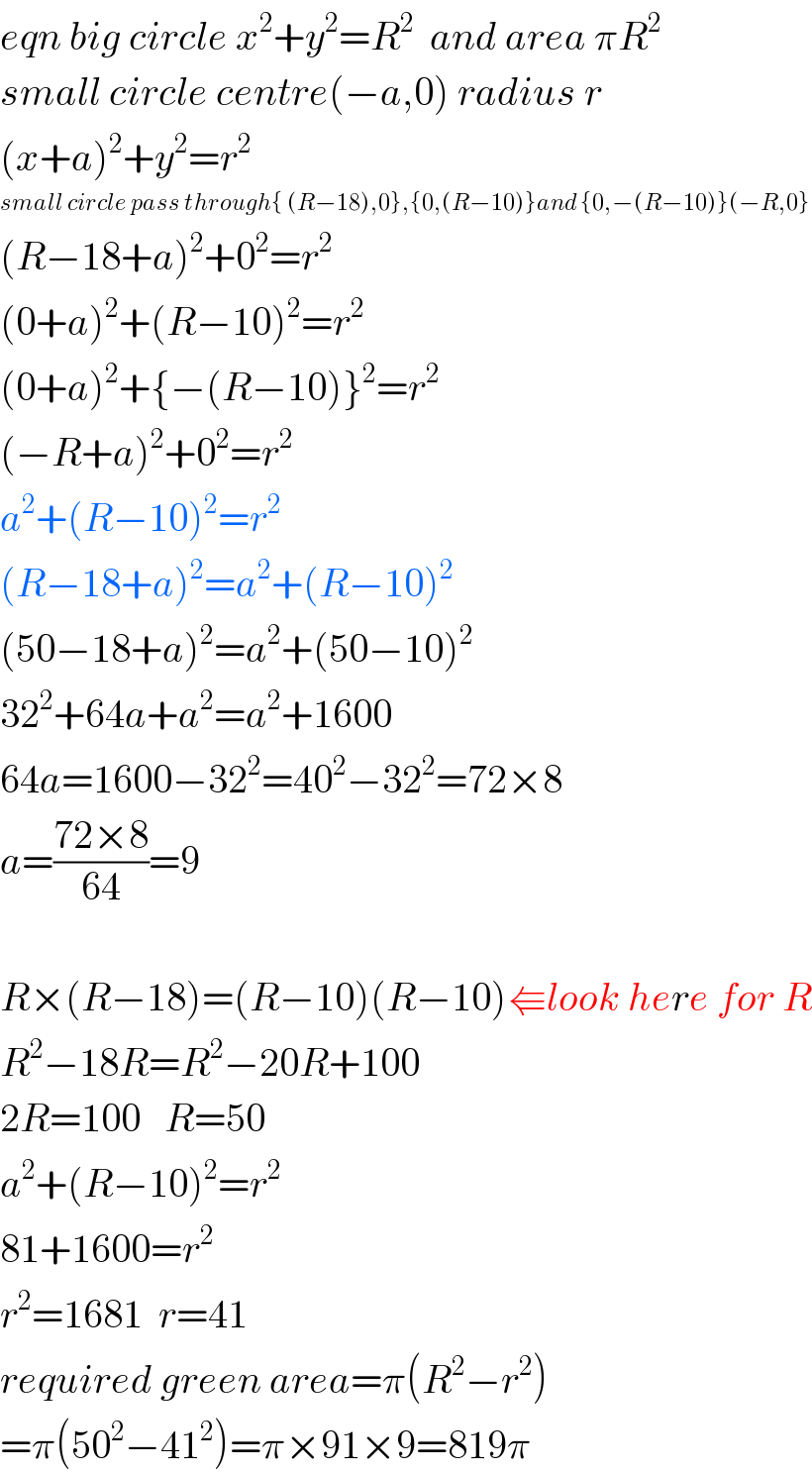
$${eqn}\:{big}\:{circle}\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={R}^{\mathrm{2}} \:\:{and}\:{area}\:\pi{R}^{\mathrm{2}} \\ $$$${small}\:{circle}\:{centre}\left(−{a},\mathrm{0}\right)\:{radius}\:{r} \\ $$$$\left({x}+{a}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$${small}\:{circle}\:{pass}\:{through}\left\{\:\left({R}−\mathrm{18}\right),\mathrm{0}\right\},\left\{\mathrm{0},\left({R}−\mathrm{10}\right)\right\}{and}\:\left\{\mathrm{0},−\left({R}−\mathrm{10}\right)\right\}\left(−{R},\mathrm{0}\right\} \\ $$$$\left({R}−\mathrm{18}+{a}\right)^{\mathrm{2}} +\mathrm{0}^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\left(\mathrm{0}+{a}\right)^{\mathrm{2}} +\left({R}−\mathrm{10}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\left(\mathrm{0}+{a}\right)^{\mathrm{2}} +\left\{−\left({R}−\mathrm{10}\right)\right\}^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\left(−{R}+{a}\right)^{\mathrm{2}} +\mathrm{0}^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$${a}^{\mathrm{2}} +\left({R}−\mathrm{10}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\left({R}−\mathrm{18}+{a}\right)^{\mathrm{2}} ={a}^{\mathrm{2}} +\left({R}−\mathrm{10}\right)^{\mathrm{2}} \\ $$$$\left(\mathrm{50}−\mathrm{18}+{a}\right)^{\mathrm{2}} ={a}^{\mathrm{2}} +\left(\mathrm{50}−\mathrm{10}\right)^{\mathrm{2}} \\ $$$$\mathrm{32}^{\mathrm{2}} +\mathrm{64}{a}+{a}^{\mathrm{2}} ={a}^{\mathrm{2}} +\mathrm{1600} \\ $$$$\mathrm{64}{a}=\mathrm{1600}−\mathrm{32}^{\mathrm{2}} =\mathrm{40}^{\mathrm{2}} −\mathrm{32}^{\mathrm{2}} =\mathrm{72}×\mathrm{8} \\ $$$${a}=\frac{\mathrm{72}×\mathrm{8}}{\mathrm{64}}=\mathrm{9} \\ $$$$ \\ $$$${R}×\left({R}−\mathrm{18}\right)=\left({R}−\mathrm{10}\right)\left({R}−\mathrm{10}\right)\Lleftarrow{look}\:{here}\:{for}\:{R} \\ $$$${R}^{\mathrm{2}} −\mathrm{18}{R}={R}^{\mathrm{2}} −\mathrm{20}{R}+\mathrm{100} \\ $$$$\mathrm{2}{R}=\mathrm{100}\:\:\:{R}=\mathrm{50} \\ $$$${a}^{\mathrm{2}} +\left({R}−\mathrm{10}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\mathrm{81}+\mathrm{1600}={r}^{\mathrm{2}} \\ $$$${r}^{\mathrm{2}} =\mathrm{1681}\:\:{r}=\mathrm{41} \\ $$$${required}\:{green}\:{area}=\pi\left({R}^{\mathrm{2}} −{r}^{\mathrm{2}} \right) \\ $$$$=\pi\left(\mathrm{50}^{\mathrm{2}} −\mathrm{41}^{\mathrm{2}} \right)=\pi×\mathrm{91}×\mathrm{9}=\mathrm{819}\pi \\ $$
