Question-121330

Question Number 121330 by ajfour last updated on 06/Nov/20

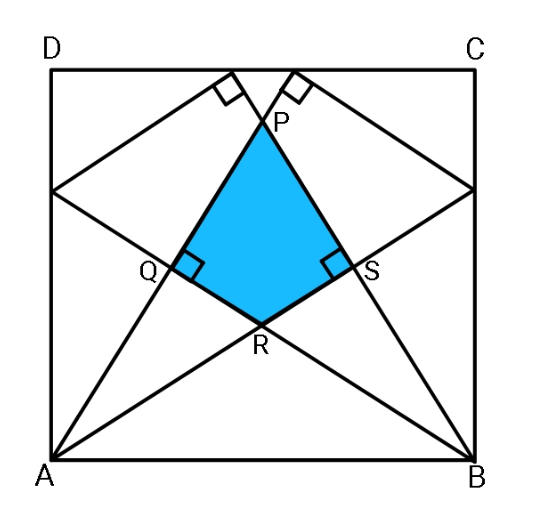
Commented by ajfour last updated on 06/Nov/20

F i n d r a t i o o f c o l o u r e d a r e a t o

o u t e r s q u a r e a r e a .

Commented by MJS_new last updated on 06/Nov/20

I think this is not possible in a square ? ? ?

but I^{'} m tired and maybe I^{'} m wrong \dots

Answered by mr W last updated on 07/Nov/20

Commented by mr W last updated on 07/Nov/20

\tan α = \frac{1 - x}{1} = 1 - x

\tan α = \frac{1 - y}{1} = 1 - y

\Rightarrow x = y

\Rightarrow A E = A F

\Rightarrow β = π - 2 \times \frac{π}{2} = 0

\Rightarrow i m p o s s i b l e

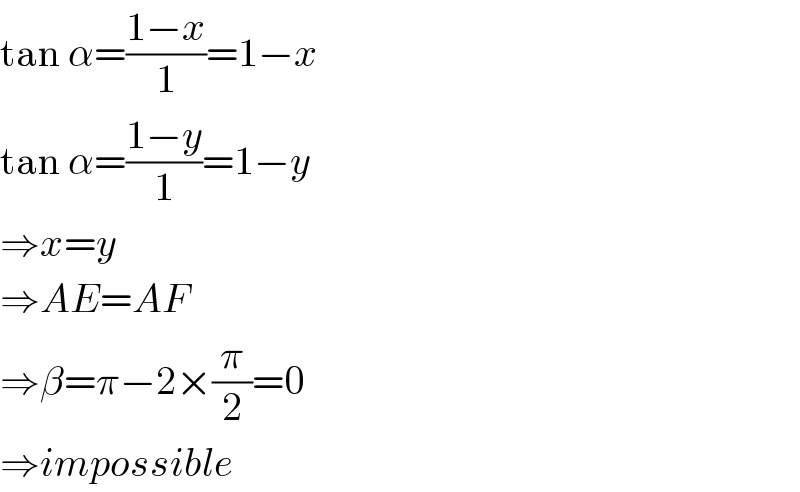
Commented by ajfour last updated on 07/Nov/20

t h a n k s f o r a t t e m p t i n g S i r .

Facebook Tweet Pin