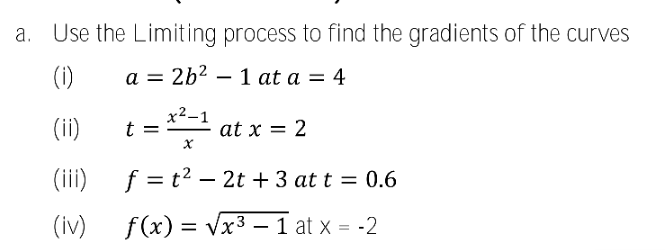
Question Number 121727 by oustmuchiya@gmail.com last updated on 11/Nov/20
Answered by TANMAY PANACEA last updated on 11/Nov/20
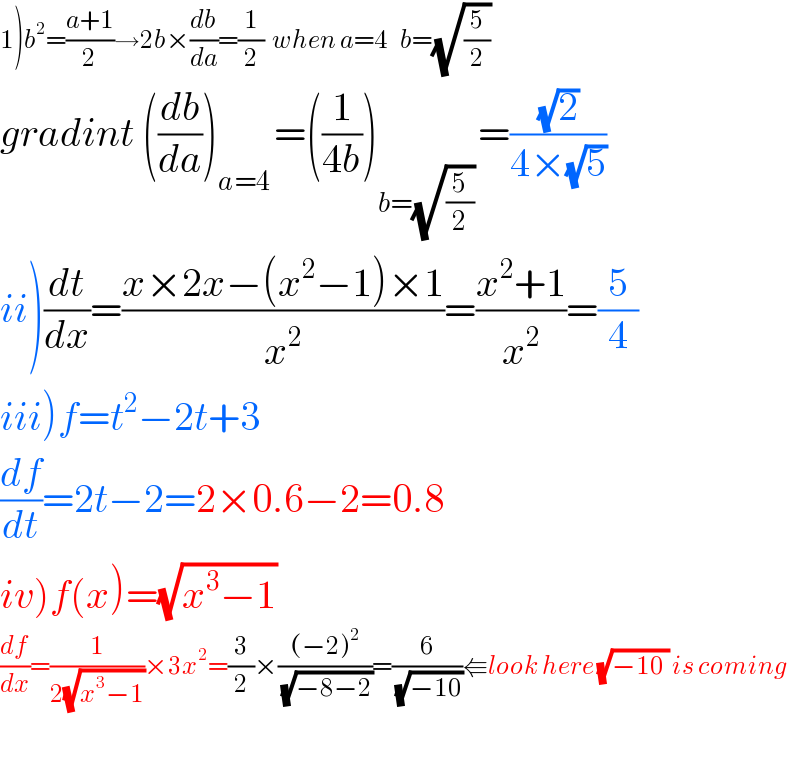
$$\left.\mathrm{1}\right){b}^{\mathrm{2}} =\frac{{a}+\mathrm{1}}{\mathrm{2}}\rightarrow\mathrm{2}{b}×\frac{{db}}{{da}}=\frac{\mathrm{1}}{\mathrm{2}}\:\:{when}\:{a}=\mathrm{4}\:\:\:{b}=\sqrt{\frac{\mathrm{5}}{\mathrm{2}}}\: \\ $$$${gradint}\:\left(\frac{{db}}{{da}}\right)_{{a}=\mathrm{4}\:} =\left(\frac{\mathrm{1}}{\mathrm{4}{b}}\right)_{{b}=\sqrt{\frac{\mathrm{5}}{\mathrm{2}}}\:} =\frac{\sqrt{\mathrm{2}}}{\mathrm{4}×\sqrt{\mathrm{5}}} \\ $$$$\left.{ii}\right)\frac{{dt}}{{dx}}=\frac{{x}×\mathrm{2}{x}−\left({x}^{\mathrm{2}} −\mathrm{1}\right)×\mathrm{1}}{{x}^{\mathrm{2}} }=\frac{{x}^{\mathrm{2}} +\mathrm{1}}{{x}^{\mathrm{2}} }=\frac{\mathrm{5}}{\mathrm{4}} \\ $$$$\left.{iii}\right){f}={t}^{\mathrm{2}} −\mathrm{2}{t}+\mathrm{3} \\ $$$$\frac{{df}}{{dt}}=\mathrm{2}{t}−\mathrm{2}=\mathrm{2}×\mathrm{0}.\mathrm{6}−\mathrm{2}=\mathrm{0}.\mathrm{8} \\ $$$$\left.{iv}\right){f}\left({x}\right)=\sqrt{{x}^{\mathrm{3}} −\mathrm{1}}\: \\ $$$$\frac{{df}}{{dx}}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{{x}^{\mathrm{3}} −\mathrm{1}}}×\mathrm{3}{x}^{\mathrm{2}} =\frac{\mathrm{3}}{\mathrm{2}}×\frac{\left(−\mathrm{2}\right)^{\mathrm{2}} }{\:\sqrt{−\mathrm{8}−\mathrm{2}}}=\frac{\mathrm{6}}{\:\sqrt{−\mathrm{10}}}\Lleftarrow{look}\:{here}\:\sqrt{−\mathrm{10}\:}\:{is}\:{coming} \\ $$$$ \\ $$
