Question Number 123601 by aurpeyz last updated on 26/Nov/20

Commented by aurpeyz last updated on 26/Nov/20

$${pls}\:{explain}\:{to}\:{me}\:{if}\:{i}\:{still}\:{need}\:{to} \\ $$$${find}\:{the}\:{class}\:{boundary}\:{since}\:{the}\: \\ $$$${class}\:{interval}\:{is}\:{already}\:{continous}. \\ $$$${thank}\:{you} \\ $$
Commented by Dwaipayan Shikari last updated on 26/Nov/20
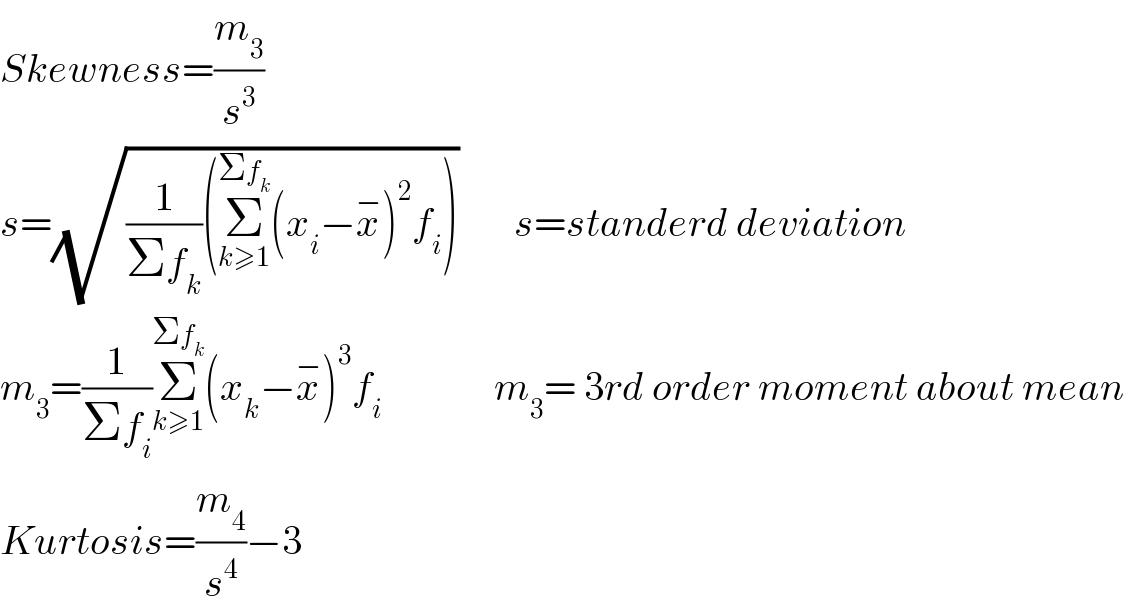
$${Skewness}=\frac{{m}_{\mathrm{3}} }{{s}^{\mathrm{3}} }\:\:\:\:\:\: \\ $$$${s}=\sqrt{\frac{\mathrm{1}}{\Sigma{f}_{{k}} }\left(\underset{{k}\geqslant\mathrm{1}} {\overset{\Sigma{f}_{{k}} } {\sum}}\left({x}_{{i}} −\overset{−} {{x}}\right)^{\mathrm{2}} {f}_{{i}} \right)}\:\:\:\:\:\:\:{s}={standerd}\:{deviation} \\ $$$${m}_{\mathrm{3}} =\frac{\mathrm{1}}{\Sigma{f}_{{i}} }\underset{{k}\geqslant\mathrm{1}} {\overset{\Sigma{f}_{{k}} } {\sum}}\left({x}_{{k}} −\overset{−} {{x}}\right)^{\mathrm{3}} {f}_{{i}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:{m}_{\mathrm{3}} =\:\mathrm{3}{rd}\:{order}\:{moment}\:{about}\:{mean} \\ $$$${Kurtosis}=\frac{{m}_{\mathrm{4}} }{{s}^{\mathrm{4}} }−\mathrm{3} \\ $$
