Question Number 125654 by ajfour last updated on 12/Dec/20
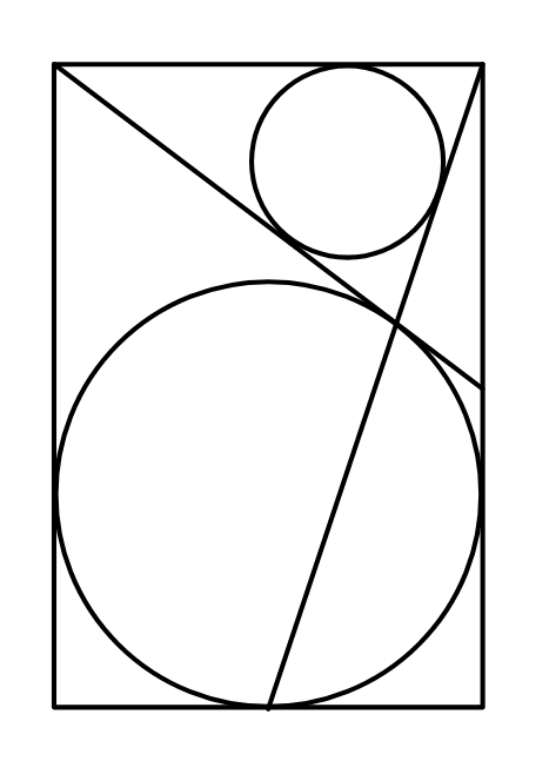
Commented by ajfour last updated on 12/Dec/20

$${Find}\:{R}/{r}. \\ $$
Answered by mr W last updated on 13/Dec/20
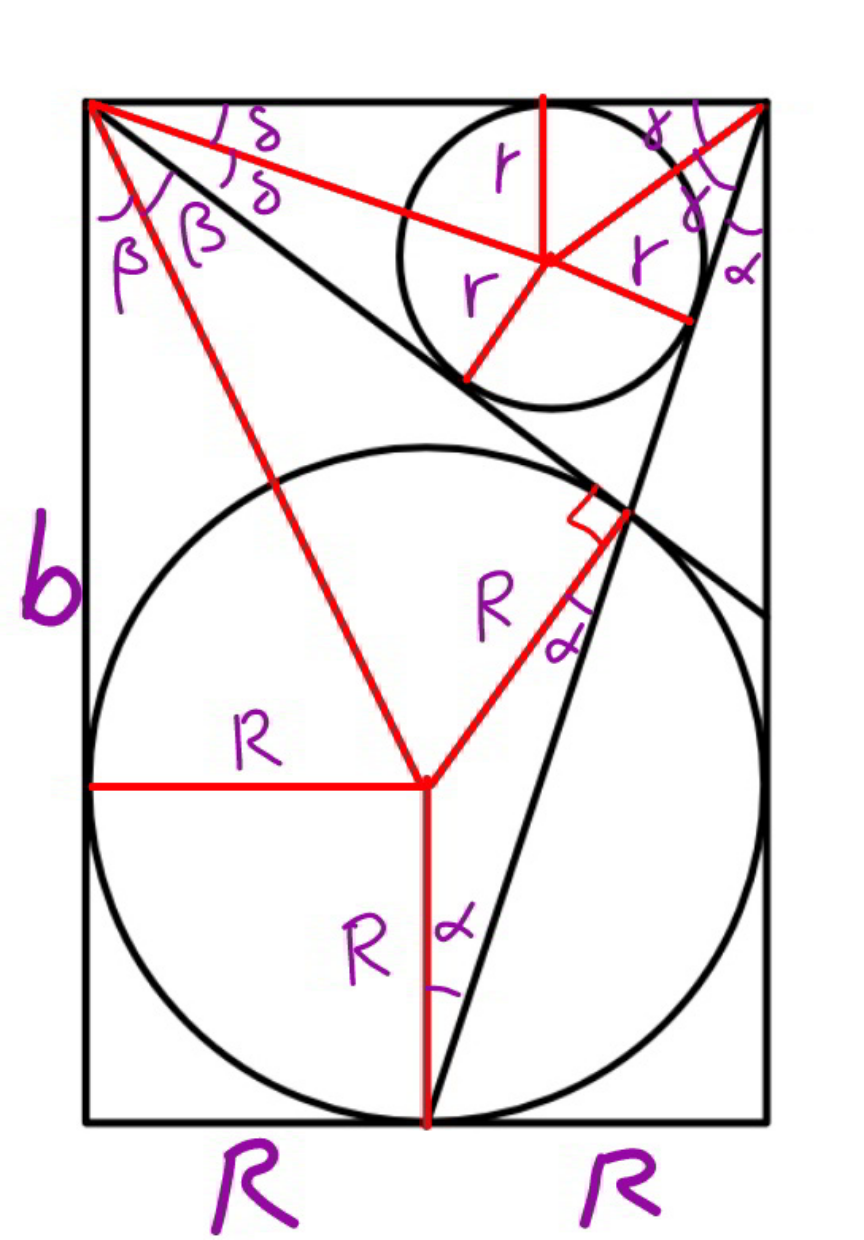
Commented by mr W last updated on 13/Dec/20
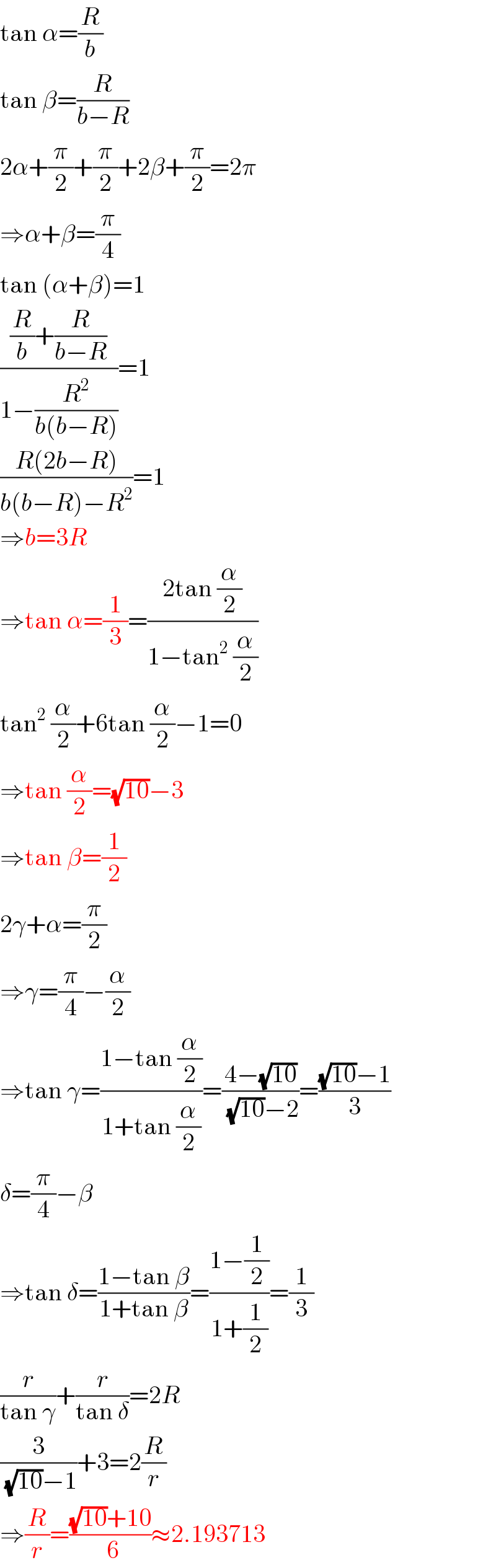
$$\mathrm{tan}\:\alpha=\frac{{R}}{{b}} \\ $$$$\mathrm{tan}\:\beta=\frac{{R}}{{b}−{R}} \\ $$$$\mathrm{2}\alpha+\frac{\pi}{\mathrm{2}}+\frac{\pi}{\mathrm{2}}+\mathrm{2}\beta+\frac{\pi}{\mathrm{2}}=\mathrm{2}\pi \\ $$$$\Rightarrow\alpha+\beta=\frac{\pi}{\mathrm{4}} \\ $$$$\mathrm{tan}\:\left(\alpha+\beta\right)=\mathrm{1} \\ $$$$\frac{\frac{{R}}{{b}}+\frac{{R}}{{b}−{R}}}{\mathrm{1}−\frac{{R}^{\mathrm{2}} }{{b}\left({b}−{R}\right)}}=\mathrm{1} \\ $$$$\frac{{R}\left(\mathrm{2}{b}−{R}\right)}{{b}\left({b}−{R}\right)−{R}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\Rightarrow{b}=\mathrm{3}{R} \\ $$$$\Rightarrow\mathrm{tan}\:\alpha=\frac{\mathrm{1}}{\mathrm{3}}=\frac{\mathrm{2tan}\:\frac{\alpha}{\mathrm{2}}}{\mathrm{1}−\mathrm{tan}^{\mathrm{2}} \:\frac{\alpha}{\mathrm{2}}} \\ $$$$\mathrm{tan}^{\mathrm{2}} \:\frac{\alpha}{\mathrm{2}}+\mathrm{6tan}\:\frac{\alpha}{\mathrm{2}}−\mathrm{1}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{tan}\:\frac{\alpha}{\mathrm{2}}=\sqrt{\mathrm{10}}−\mathrm{3} \\ $$$$\Rightarrow\mathrm{tan}\:\beta=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{2}\gamma+\alpha=\frac{\pi}{\mathrm{2}} \\ $$$$\Rightarrow\gamma=\frac{\pi}{\mathrm{4}}−\frac{\alpha}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{tan}\:\gamma=\frac{\mathrm{1}−\mathrm{tan}\:\frac{\alpha}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}\:\frac{\alpha}{\mathrm{2}}}=\frac{\mathrm{4}−\sqrt{\mathrm{10}}}{\:\sqrt{\mathrm{10}}−\mathrm{2}}=\frac{\sqrt{\mathrm{10}}−\mathrm{1}}{\mathrm{3}} \\ $$$$\delta=\frac{\pi}{\mathrm{4}}−\beta \\ $$$$\Rightarrow\mathrm{tan}\:\delta=\frac{\mathrm{1}−\mathrm{tan}\:\beta}{\mathrm{1}+\mathrm{tan}\:\beta}=\frac{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}}{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\frac{{r}}{\mathrm{tan}\:\gamma}+\frac{{r}}{\mathrm{tan}\:\delta}=\mathrm{2}{R} \\ $$$$\frac{\mathrm{3}}{\:\sqrt{\mathrm{10}}−\mathrm{1}}+\mathrm{3}=\mathrm{2}\frac{{R}}{{r}} \\ $$$$\Rightarrow\frac{{R}}{{r}}=\frac{\sqrt{\mathrm{10}}+\mathrm{10}}{\:\mathrm{6}}\approx\mathrm{2}.\mathrm{193713} \\ $$
Commented by ajfour last updated on 13/Dec/20
$${Beautifully}\:{solved},\:{Sir}!\:{Too}\:{good}. \\ $$
