Question-128764

Question Number 128764 by behi83417@gmail.com last updated on 10/Jan/21

Commented by behi83417@gmail.com last updated on 10/Jan/21

$$\measuredangle\mathrm{DAB}=\measuredangle\mathrm{CBA}=\mathrm{30}^{\bullet} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{AB}}=? \\ $$

Answered by Olaf last updated on 10/Jan/21

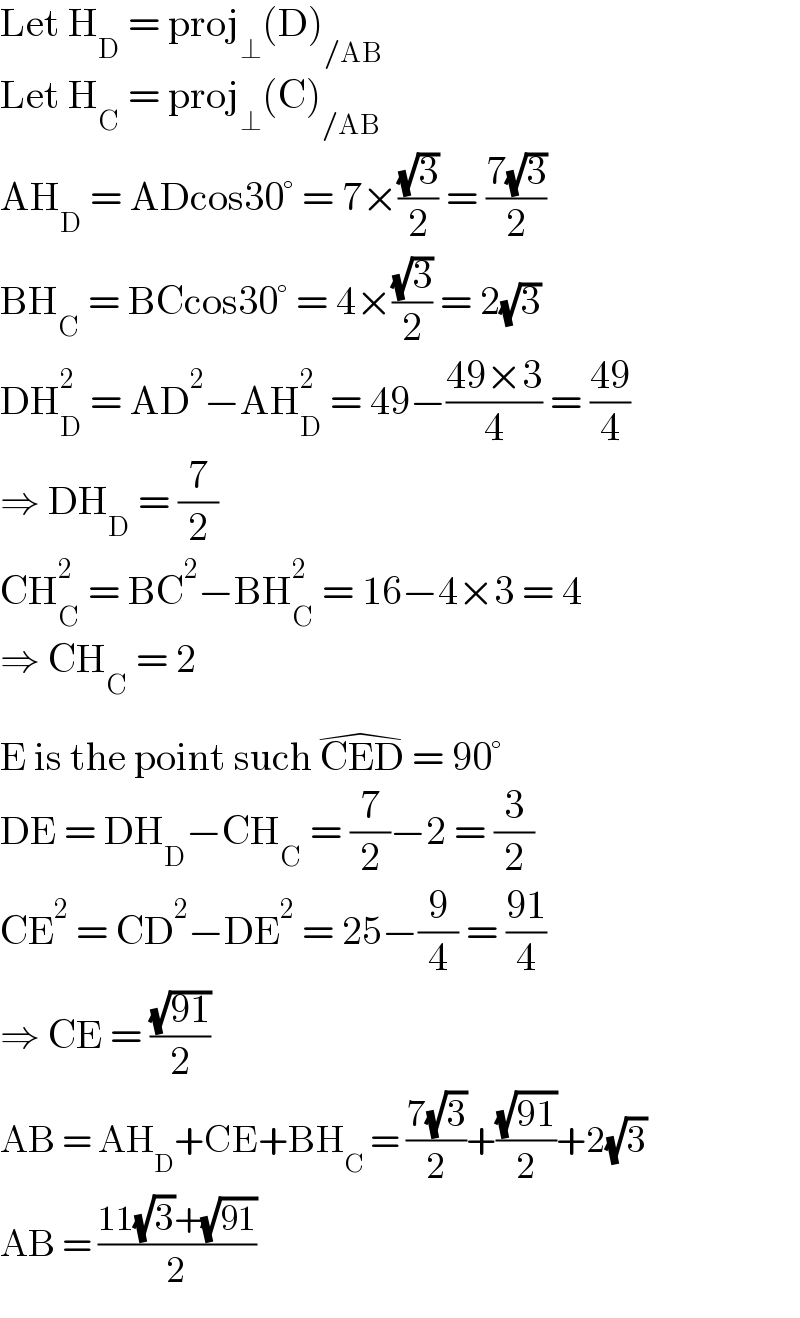
Let H_D = proj_⊥ (D)_(/AB) Let H_C = proj_⊥ (C)_(/AB) AH_D = ADcos30° = 7×((√3)/2) = ((7(√3))/2) BH_C = BCcos30° = 4×((√3)/2) = 2(√3) DH_D ^2 = AD^2 −AH_D ^2 = 49−((49×3)/4) = ((49)/4) ⇒ DH_D = (7/2) CH_C ^2 = BC^2 −BH_C ^2 = 16−4×3 = 4 ⇒ CH_C = 2 E is the point such CED^(�) = 90° DE = DH_D −CH_C = (7/2)−2 = (3/2) CE^2 = CD^2 −DE^2 = 25−(9/4) = ((91)/4) ⇒ CE = ((√(91))/2) AB = AH_D +CE+BH_C = ((7(√3))/2)+((√(91))/2)+2(√3) AB = ((11(√3)+(√(91)))/2)

$$\mathrm{Let}\:\mathrm{H}_{\mathrm{D}} \:=\:\mathrm{proj}_{\bot} \left(\mathrm{D}\right)_{/\mathrm{AB}} \\ $$$$\mathrm{Let}\:\mathrm{H}_{\mathrm{C}} \:=\:\mathrm{proj}_{\bot} \left(\mathrm{C}\right)_{/\mathrm{AB}} \\ $$$$\mathrm{AH}_{\mathrm{D}} \:=\:\mathrm{ADcos30}°\:=\:\mathrm{7}×\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:=\:\frac{\mathrm{7}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\mathrm{BH}_{\mathrm{C}} \:=\:\mathrm{BCcos30}°\:=\:\mathrm{4}×\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:=\:\mathrm{2}\sqrt{\mathrm{3}} \\ $$$$\mathrm{DH}_{\mathrm{D}} ^{\mathrm{2}} \:=\:\mathrm{AD}^{\mathrm{2}} −\mathrm{AH}_{\mathrm{D}} ^{\mathrm{2}} \:=\:\mathrm{49}−\frac{\mathrm{49}×\mathrm{3}}{\mathrm{4}}\:=\:\frac{\mathrm{49}}{\mathrm{4}} \\ $$$$\Rightarrow\:\mathrm{DH}_{\mathrm{D}} \:=\:\frac{\mathrm{7}}{\mathrm{2}} \\ $$$$\mathrm{CH}_{\mathrm{C}} ^{\mathrm{2}} \:=\:\mathrm{BC}^{\mathrm{2}} −\mathrm{BH}_{\mathrm{C}} ^{\mathrm{2}} \:=\:\mathrm{16}−\mathrm{4}×\mathrm{3}\:=\:\mathrm{4} \\ $$$$\Rightarrow\:\mathrm{CH}_{\mathrm{C}} \:=\:\mathrm{2} \\ $$$$\mathrm{E}\:\mathrm{is}\:\mathrm{the}\:\mathrm{point}\:\mathrm{such}\:\widehat {\mathrm{CED}}\:=\:\mathrm{90}° \\ $$$$\mathrm{DE}\:=\:\mathrm{DH}_{\mathrm{D}} −\mathrm{CH}_{\mathrm{C}} \:=\:\frac{\mathrm{7}}{\mathrm{2}}−\mathrm{2}\:=\:\frac{\mathrm{3}}{\mathrm{2}} \\ $$$$\mathrm{CE}^{\mathrm{2}} \:=\:\mathrm{CD}^{\mathrm{2}} −\mathrm{DE}^{\mathrm{2}} \:=\:\mathrm{25}−\frac{\mathrm{9}}{\mathrm{4}}\:=\:\frac{\mathrm{91}}{\mathrm{4}} \\ $$$$\Rightarrow\:\mathrm{CE}\:=\:\frac{\sqrt{\mathrm{91}}}{\mathrm{2}} \\ $$$$\mathrm{AB}\:=\:\mathrm{AH}_{\mathrm{D}} +\mathrm{CE}+\mathrm{BH}_{\mathrm{C}} \:=\:\frac{\mathrm{7}\sqrt{\mathrm{3}}}{\mathrm{2}}+\frac{\sqrt{\mathrm{91}}}{\mathrm{2}}+\mathrm{2}\sqrt{\mathrm{3}} \\ $$$$\mathrm{AB}\:=\:\frac{\mathrm{11}\sqrt{\mathrm{3}}+\sqrt{\mathrm{91}}}{\mathrm{2}} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply