Question Number 13835 by Tinkutara last updated on 24/May/17

Commented by Tinkutara last updated on 24/May/17
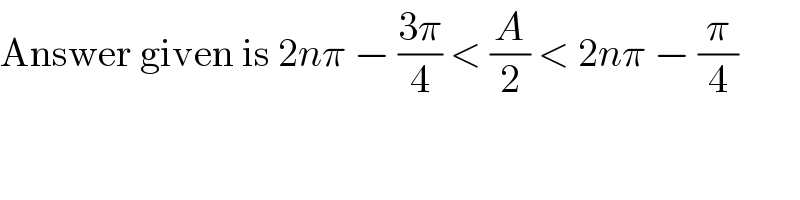
$$\mathrm{Answer}\:\mathrm{given}\:\mathrm{is}\:\mathrm{2}{n}\pi\:−\:\frac{\mathrm{3}\pi}{\mathrm{4}}\:<\:\frac{{A}}{\mathrm{2}}\:<\:\mathrm{2}{n}\pi\:−\:\frac{\pi}{\mathrm{4}} \\ $$
Answered by ajfour last updated on 24/May/17

$${if}\:{A}=\frac{\pi}{\mathrm{2}}−\mathrm{2}{B},\:{then}\:\:\:\frac{{A}}{\mathrm{2}}=\frac{\pi}{\mathrm{4}}−{B} \\ $$$$\mathrm{2sin}\:\left(\frac{\pi}{\mathrm{4}}−{B}\right)=−\sqrt{\mathrm{1}+\mathrm{cos}\:\mathrm{2}{B}}−\sqrt{\mathrm{1}−\mathrm{cos}\:\mathrm{2}{B}} \\ $$$$\mathrm{2sin}\:\left(\frac{\pi}{\mathrm{4}}−{B}\right)=−\sqrt{\mathrm{2}}\mid\mathrm{cos}\:{B}\mid−\sqrt{\mathrm{2}}\mid\mathrm{sin}\:{B}\mid\mid \\ $$$$\mathrm{cos}\:{B}−\mathrm{sin}\:{B}+\mid\mathrm{cos}\:{B}\mid+\mid\mathrm{sin}\:{B}\mid=\mathrm{0} \\ $$$$\Rightarrow\:\mathrm{sin}\:{B}\geqslant\mathrm{0}\:,\:\mathrm{cos}\:{B}\leqslant\mathrm{0} \\ $$$$−\left(\mathrm{2}{n}−\mathrm{1}\right)\pi−\frac{\pi}{\mathrm{2}}\leqslant\:{B}\:\leqslant−\left(\mathrm{2}{n}−\mathrm{1}\right)\pi \\ $$$$−\left(\mathrm{2}{n}−\mathrm{1}\right)\pi−\frac{\pi}{\mathrm{2}}\leqslant\:\frac{\pi}{\mathrm{4}}−\frac{{A}}{\mathrm{2}}\:\leqslant−\left(\mathrm{2}{n}−\mathrm{1}\right)\pi \\ $$$$\Rightarrow\:\frac{{A}}{\mathrm{2}}\leqslant\mathrm{2}{n}\pi−\pi+\frac{\pi}{\mathrm{4}}+\frac{\pi}{\mathrm{2}} \\ $$$${and}\:\:\frac{{A}}{\mathrm{2}}\geqslant\mathrm{2}{n}\pi−\pi+\frac{\pi}{\mathrm{4}} \\ $$$$\mathrm{2}\boldsymbol{{n}\pi}−\frac{\mathrm{3}\boldsymbol{\pi}}{\mathrm{4}}\leqslant\:\frac{{A}}{\mathrm{2}}\:\leqslant\:\mathrm{2}\boldsymbol{{n}\pi}−\frac{\boldsymbol{\pi}}{\mathrm{4}}\:. \\ $$
