Question Number 145379 by ajfour last updated on 04/Jul/21

Commented by ajfour last updated on 04/Jul/21
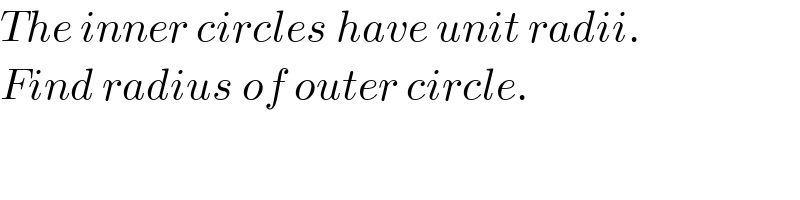
$${The}\:{inner}\:{circles}\:{have}\:{unit}\:{radii}. \\ $$$${Find}\:{radius}\:{of}\:{outer}\:{circle}. \\ $$
Answered by mr W last updated on 04/Jul/21

Commented by mr W last updated on 04/Jul/21
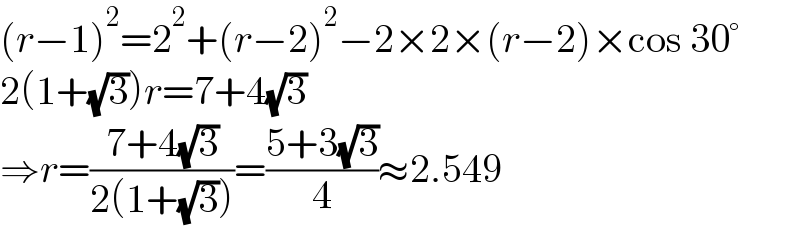
$$\left({r}−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{2}^{\mathrm{2}} +\left({r}−\mathrm{2}\right)^{\mathrm{2}} −\mathrm{2}×\mathrm{2}×\left({r}−\mathrm{2}\right)×\mathrm{cos}\:\mathrm{30}° \\ $$$$\mathrm{2}\left(\mathrm{1}+\sqrt{\mathrm{3}}\right){r}=\mathrm{7}+\mathrm{4}\sqrt{\mathrm{3}} \\ $$$$\Rightarrow{r}=\frac{\mathrm{7}+\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{2}\left(\mathrm{1}+\sqrt{\mathrm{3}}\right)}=\frac{\mathrm{5}+\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{4}}\approx\mathrm{2}.\mathrm{549} \\ $$
Commented by ajfour last updated on 04/Jul/21
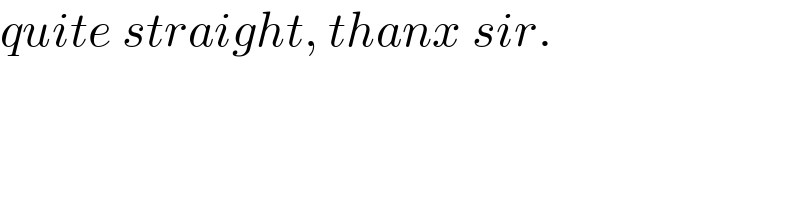
$${quite}\:{straight},\:{thanx}\:{sir}. \\ $$
