Question Number 145652 by imjagoll last updated on 07/Jul/21
Answered by john_santu last updated on 07/Jul/21

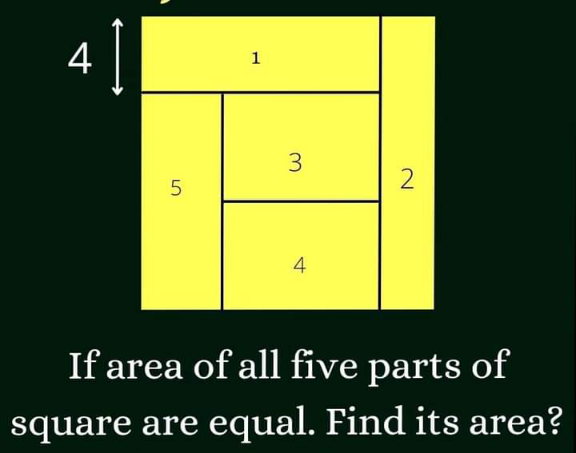
$${area}\:{of}\:{square}\:=\:\left(\mathrm{4}+{y}\right)^{\mathrm{2}} \\ $$$${let}\:{length}\:{side}\:{of}\:{area}\:{I}\:=\:{x} \\ $$$${then}\:{area}\:{of}\:{I}=\:\mathrm{4}{x}\: \\ $$$${the}\:{sum}\:{area}\:{of}\:\mathrm{3},\mathrm{4},\mathrm{5}\:=\:\mathrm{12}{x}\:=\:{xy} \\ $$$${then}\:{we}\:{get}\:{y}=\mathrm{12}\:{and}\:{area}\:{of}\:{square} \\ $$$$=\:\left(\mathrm{4}+\mathrm{12}\right)^{\mathrm{2}} \:=\:\mathrm{16}^{\mathrm{2}} =\:\mathrm{256} \\ $$$$ \\ $$
Answered by mr W last updated on 07/Jul/21
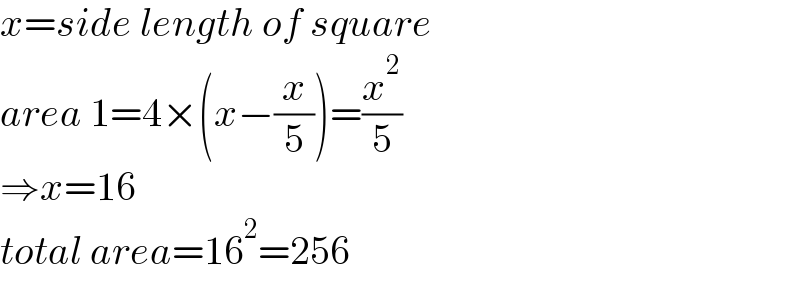
$${x}={side}\:{length}\:{of}\:{square} \\ $$$${area}\:\mathrm{1}=\mathrm{4}×\left({x}−\frac{{x}}{\mathrm{5}}\right)=\frac{{x}^{\mathrm{2}} }{\mathrm{5}} \\ $$$$\Rightarrow{x}=\mathrm{16} \\ $$$${total}\:{area}=\mathrm{16}^{\mathrm{2}} =\mathrm{256} \\ $$
