Question Number 146849 by Apor_mu_calculus last updated on 16/Jul/21
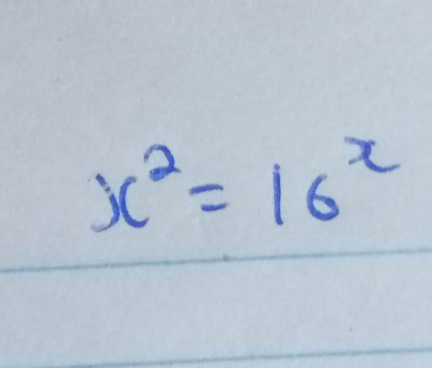
Commented by Cyriille last updated on 16/Jul/21

$$\mathrm{To}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{equation}\:\mathrm{you}\:\mathrm{have}\: \\ $$$$\mathrm{to}\:\mathrm{plot}\:\mathrm{2}\:\mathrm{graphs}\:; \\ $$$${y}={x}^{\mathrm{2}} \:\:\:{and} \\ $$$${y}=\mathrm{16}^{{x}} \\ $$$$\mathrm{your}\:\mathrm{solution}\:\mathrm{will}\:\mathrm{be}\:\mathrm{the}\:\mathrm{point}\:\mathrm{of}\: \\ $$$$\mathrm{intersection} \\ $$
Answered by ajfour last updated on 16/Jul/21

$$\mathrm{2ln}\:{x}=\mathrm{2}{x}\mathrm{ln}\:\mathrm{4} \\ $$$$\mathrm{ln}\:{x}={x}\mathrm{ln}\:\mathrm{4} \\ $$$$\frac{\mathrm{ln}\:{x}}{\mathrm{ln}\:\mathrm{4}}={x} \\ $$$${seems}\:{like}\:{there}\:{got}\:{to}\:{be} \\ $$$${some}\:{simpler}\:{solution}\:{to} \\ $$$${this},\:{but}\:{i}\:{dunnow}. \\ $$
