Question Number 147007 by Ar Brandon last updated on 17/Jul/21

Answered by Olaf_Thorendsen last updated on 17/Jul/21
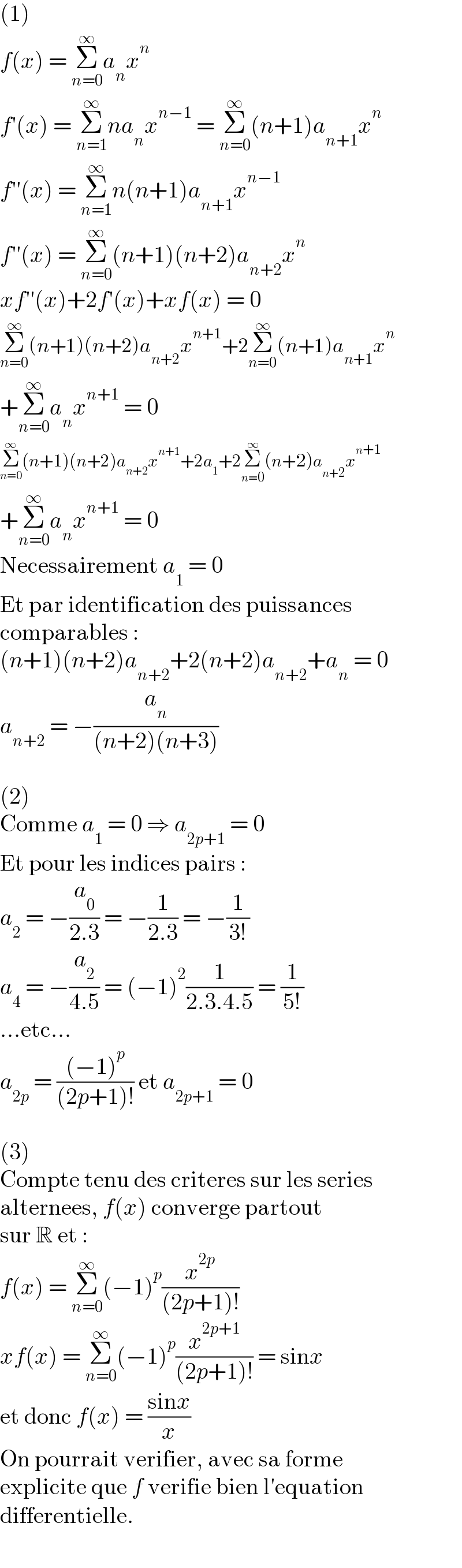
$$\left(\mathrm{1}\right) \\ $$$${f}\left({x}\right)\:=\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}{a}_{{n}} {x}^{{n}} \\ $$$${f}'\left({x}\right)\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{na}_{{n}} {x}^{{n}−\mathrm{1}} \:=\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right){a}_{{n}+\mathrm{1}} {x}^{{n}} \\ $$$${f}''\left({x}\right)\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{n}\left({n}+\mathrm{1}\right){a}_{{n}+\mathrm{1}} {x}^{{n}−\mathrm{1}} \\ $$$${f}''\left({x}\right)\:=\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right){a}_{{n}+\mathrm{2}} {x}^{{n}} \\ $$$${xf}''\left({x}\right)+\mathrm{2}{f}'\left({x}\right)+{xf}\left({x}\right)\:=\:\mathrm{0} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right){a}_{{n}+\mathrm{2}} {x}^{{n}+\mathrm{1}} +\mathrm{2}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right){a}_{{n}+\mathrm{1}} {x}^{{n}} \\ $$$$+\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}{a}_{{n}} {x}^{{n}+\mathrm{1}} \:=\:\mathrm{0} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right){a}_{{n}+\mathrm{2}} {x}^{{n}+\mathrm{1}} +\mathrm{2}{a}_{\mathrm{1}} +\mathrm{2}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{2}\right){a}_{{n}+\mathrm{2}} {x}^{{n}+\mathrm{1}} \\ $$$$+\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}{a}_{{n}} {x}^{{n}+\mathrm{1}} \:=\:\mathrm{0} \\ $$$$\mathrm{Necessairement}\:{a}_{\mathrm{1}} \:=\:\mathrm{0} \\ $$$$\mathrm{Et}\:\mathrm{par}\:\mathrm{identification}\:\mathrm{des}\:\mathrm{puissances} \\ $$$$\mathrm{comparables}\:: \\ $$$$\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right){a}_{{n}+\mathrm{2}} +\mathrm{2}\left({n}+\mathrm{2}\right){a}_{{n}+\mathrm{2}} +{a}_{{n}} \:=\:\mathrm{0} \\ $$$${a}_{{n}+\mathrm{2}} \:=\:−\frac{{a}_{{n}} }{\left({n}+\mathrm{2}\right)\left({n}+\mathrm{3}\right)} \\ $$$$ \\ $$$$\left(\mathrm{2}\right) \\ $$$$\mathrm{Comme}\:{a}_{\mathrm{1}} \:=\:\mathrm{0}\:\Rightarrow\:{a}_{\mathrm{2}{p}+\mathrm{1}} \:=\:\mathrm{0} \\ $$$$\mathrm{Et}\:\mathrm{pour}\:\mathrm{les}\:\mathrm{indices}\:\mathrm{pairs}\:: \\ $$$${a}_{\mathrm{2}} \:=\:−\frac{{a}_{\mathrm{0}} }{\mathrm{2}.\mathrm{3}}\:=\:−\frac{\mathrm{1}}{\mathrm{2}.\mathrm{3}}\:=\:−\frac{\mathrm{1}}{\mathrm{3}!} \\ $$$${a}_{\mathrm{4}} \:=\:−\frac{{a}_{\mathrm{2}} }{\mathrm{4}.\mathrm{5}}\:=\:\left(−\mathrm{1}\right)^{\mathrm{2}} \frac{\mathrm{1}}{\mathrm{2}.\mathrm{3}.\mathrm{4}.\mathrm{5}}\:=\:\frac{\mathrm{1}}{\mathrm{5}!} \\ $$$$…\mathrm{etc}… \\ $$$${a}_{\mathrm{2}{p}} \:=\:\frac{\left(−\mathrm{1}\right)^{{p}} }{\left(\mathrm{2}{p}+\mathrm{1}\right)!}\:\mathrm{et}\:{a}_{\mathrm{2}{p}+\mathrm{1}} \:=\:\mathrm{0} \\ $$$$ \\ $$$$\left(\mathrm{3}\right) \\ $$$$\mathrm{Compte}\:\mathrm{tenu}\:\mathrm{des}\:\mathrm{criteres}\:\mathrm{sur}\:\mathrm{les}\:\mathrm{series} \\ $$$$\mathrm{alternees},\:{f}\left({x}\right)\:\mathrm{converge}\:\mathrm{partout} \\ $$$$\mathrm{sur}\:\mathbb{R}\:\mathrm{et}\:: \\ $$$${f}\left({x}\right)\:=\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(−\mathrm{1}\right)^{{p}} \frac{{x}^{\mathrm{2}{p}} }{\left(\mathrm{2}{p}+\mathrm{1}\right)!} \\ $$$${xf}\left({x}\right)\:=\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(−\mathrm{1}\right)^{{p}} \frac{{x}^{\mathrm{2}{p}+\mathrm{1}} }{\left(\mathrm{2}{p}+\mathrm{1}\right)!}\:=\:\mathrm{sin}{x} \\ $$$$\mathrm{et}\:\mathrm{donc}\:{f}\left({x}\right)\:=\:\frac{\mathrm{sin}{x}}{{x}} \\ $$$$\mathrm{On}\:\mathrm{pourrait}\:\mathrm{verifier},\:\mathrm{avec}\:\mathrm{sa}\:\mathrm{forme} \\ $$$$\mathrm{explicite}\:\mathrm{que}\:{f}\:\mathrm{verifie}\:\mathrm{bien}\:\mathrm{l}'\mathrm{equation} \\ $$$$\mathrm{differentielle}. \\ $$
Commented by Ar Brandon last updated on 17/Jul/21

$$\mathrm{Merci}\:\mathrm{beaucoup}\:\mathrm{monsieur}. \\ $$$$\mathrm{Bien}\:\mathrm{le}\:\mathrm{jour}\:! \\ $$
