Question Number 14863 by b.e.h.i.8.3.4.1.7@gmail.com last updated on 05/Jun/17

Commented by adelson last updated on 05/Jun/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 05/Jun/17
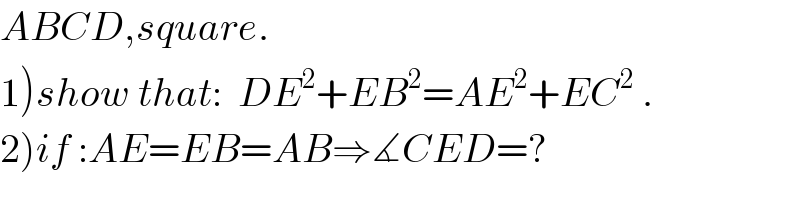
Commented by RasheedSoomro last updated on 05/Jun/17
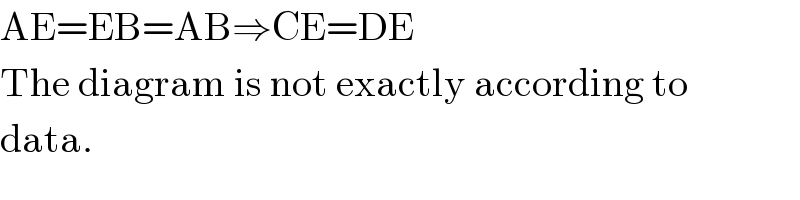
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 05/Jun/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 05/Jun/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 05/Jun/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 05/Jun/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 05/Jun/17
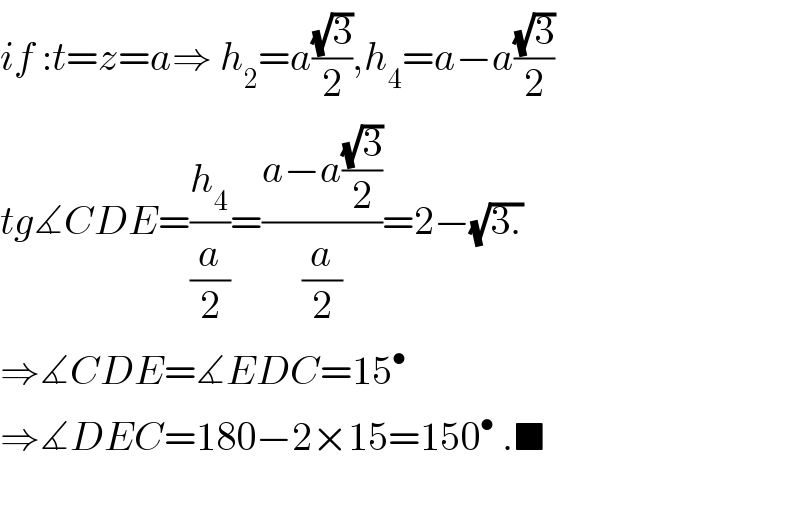
Answered by ajfour last updated on 05/Jun/17
![DE^2 +EB^2 =x^2 +y^2 +(a−x)^2 +(a−y)^2 AE^2 +EC^2 =(a−x)^2 +y^2 +x^2 +(a−y)^2 therefore equal. When △AEB is equilateral, a−y=y or y=(a/2) a−x= acos (π/6) = ((a(√3))/2) ⇒ x =a(1−((√3)/2)) = a(((2−(√3))/2)) ∠CED= 2tan^(−1) (((a−y)/x)) =2tan^(−1) ((y/x)) =2tan^(−1) [(((a/2))/(a(2−(√3))/2)))] =2tan^(−1) (2+(√3)) or ∠CED=π− tan^(−1) ((1/( (√3)))) =π−(π/6)=((5π)/6) .](https://www.tinkutara.com/question/Q14871.png)
Commented by ajfour last updated on 05/Jun/17

Commented by RasheedSoomro last updated on 05/Jun/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 05/Jun/17

Commented by ajfour last updated on 05/Jun/17

