Question Number 148767 by mim24 last updated on 31/Jul/21

Answered by liberty last updated on 31/Jul/21
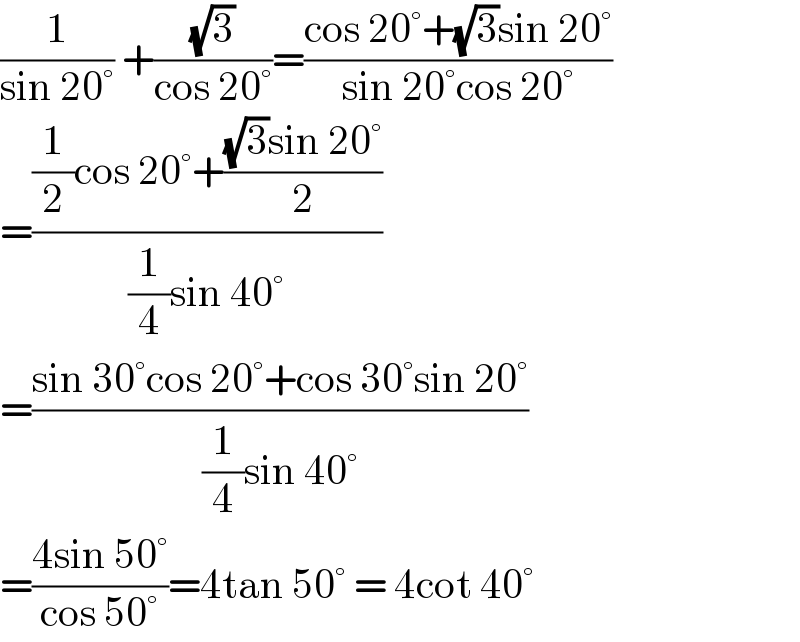
$$\frac{\mathrm{1}}{\mathrm{sin}\:\mathrm{20}°}\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{cos}\:\mathrm{20}°}=\frac{\mathrm{cos}\:\mathrm{20}°+\sqrt{\mathrm{3}}\mathrm{sin}\:\mathrm{20}°}{\mathrm{sin}\:\mathrm{20}°\mathrm{cos}\:\mathrm{20}°} \\ $$$$=\frac{\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\mathrm{20}°+\frac{\sqrt{\mathrm{3}}\mathrm{sin}\:\mathrm{20}°}{\mathrm{2}}}{\frac{\mathrm{1}}{\mathrm{4}}\mathrm{sin}\:\mathrm{40}°} \\ $$$$=\frac{\mathrm{sin}\:\mathrm{30}°\mathrm{cos}\:\mathrm{20}°+\mathrm{cos}\:\mathrm{30}°\mathrm{sin}\:\mathrm{20}°}{\frac{\mathrm{1}}{\mathrm{4}}\mathrm{sin}\:\mathrm{40}°} \\ $$$$=\frac{\mathrm{4sin}\:\mathrm{50}°}{\mathrm{cos}\:\mathrm{50}°}=\mathrm{4tan}\:\mathrm{50}°\:=\:\mathrm{4cot}\:\mathrm{40}° \\ $$
