Question-149031

Question Number 149031 by puissant last updated on 02/Aug/21

Answered by EDWIN88 last updated on 02/Aug/21

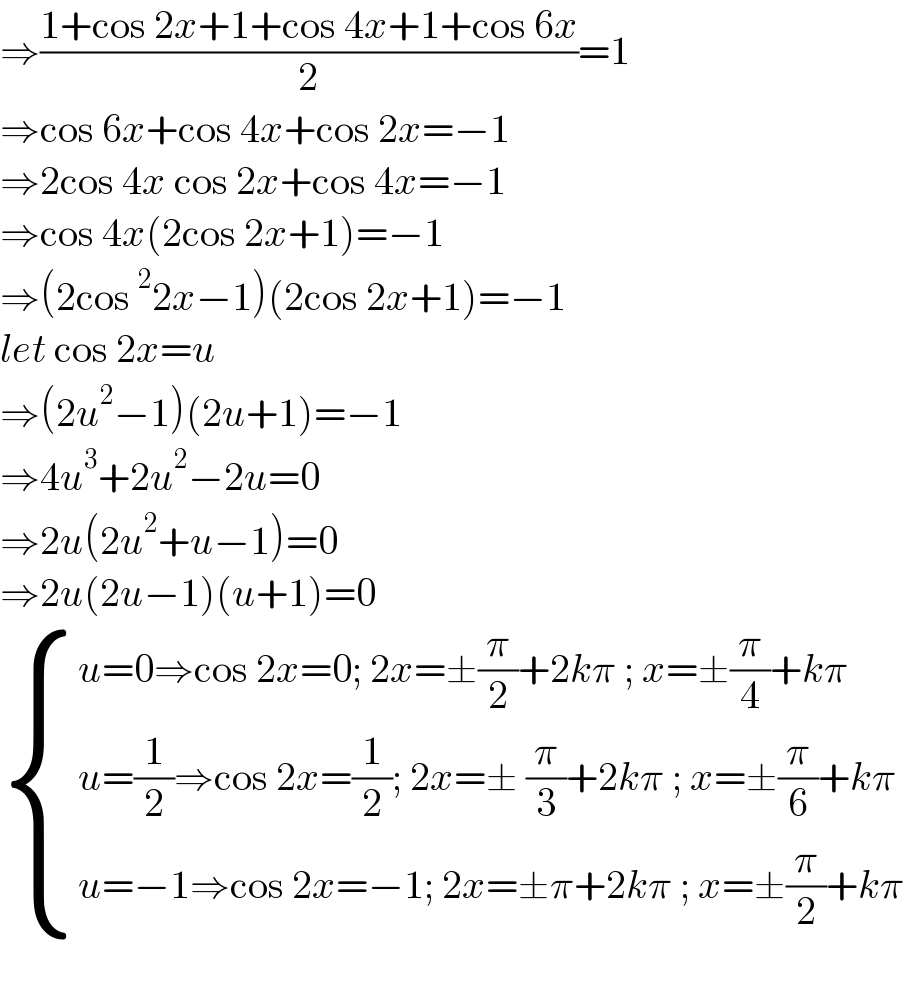
⇒((1+cos 2x+1+cos 4x+1+cos 6x)/2)=1 ⇒cos 6x+cos 4x+cos 2x=−1 ⇒2cos 4x cos 2x+cos 4x=−1 ⇒cos 4x(2cos 2x+1)=−1 ⇒(2cos^2 2x−1)(2cos 2x+1)=−1 let cos 2x=u ⇒(2u^2 −1)(2u+1)=−1 ⇒4u^3 +2u^2 −2u=0 ⇒2u(2u^2 +u−1)=0 ⇒2u(2u−1)(u+1)=0 { ((u=0⇒cos 2x=0; 2x=±(π/2)+2kπ ; x=±(π/4)+kπ)),((u=(1/2)⇒cos 2x=(1/2); 2x=± (π/3)+2kπ ; x=±(π/6)+kπ )),((u=−1⇒cos 2x=−1; 2x=±π+2kπ ; x=±(π/2)+kπ)) :}

$$\Rightarrow\frac{\mathrm{1}+\mathrm{cos}\:\mathrm{2}{x}+\mathrm{1}+\mathrm{cos}\:\mathrm{4}{x}+\mathrm{1}+\mathrm{cos}\:\mathrm{6}{x}}{\mathrm{2}}=\mathrm{1} \\ $$$$\Rightarrow\mathrm{cos}\:\mathrm{6}{x}+\mathrm{cos}\:\mathrm{4}{x}+\mathrm{cos}\:\mathrm{2}{x}=−\mathrm{1} \\ $$$$\Rightarrow\mathrm{2cos}\:\mathrm{4}{x}\:\mathrm{cos}\:\mathrm{2}{x}+\mathrm{cos}\:\mathrm{4}{x}=−\mathrm{1} \\ $$$$\Rightarrow\mathrm{cos}\:\mathrm{4}{x}\left(\mathrm{2cos}\:\mathrm{2}{x}+\mathrm{1}\right)=−\mathrm{1} \\ $$$$\Rightarrow\left(\mathrm{2cos}\:^{\mathrm{2}} \mathrm{2}{x}−\mathrm{1}\right)\left(\mathrm{2cos}\:\mathrm{2}{x}+\mathrm{1}\right)=−\mathrm{1} \\ $$$${let}\:\mathrm{cos}\:\mathrm{2}{x}={u} \\ $$$$\Rightarrow\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}\right)\left(\mathrm{2}{u}+\mathrm{1}\right)=−\mathrm{1} \\ $$$$\Rightarrow\mathrm{4}{u}^{\mathrm{3}} +\mathrm{2}{u}^{\mathrm{2}} −\mathrm{2}{u}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{2}{u}\left(\mathrm{2}{u}^{\mathrm{2}} +{u}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{2}{u}\left(\mathrm{2}{u}−\mathrm{1}\right)\left({u}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\begin{cases}{{u}=\mathrm{0}\Rightarrow\mathrm{cos}\:\mathrm{2}{x}=\mathrm{0};\:\mathrm{2}{x}=\pm\frac{\pi}{\mathrm{2}}+\mathrm{2}{k}\pi\:;\:{x}=\pm\frac{\pi}{\mathrm{4}}+{k}\pi}\\{{u}=\frac{\mathrm{1}}{\mathrm{2}}\Rightarrow\mathrm{cos}\:\mathrm{2}{x}=\frac{\mathrm{1}}{\mathrm{2}};\:\mathrm{2}{x}=\pm\:\frac{\pi}{\mathrm{3}}+\mathrm{2}{k}\pi\:;\:{x}=\pm\frac{\pi}{\mathrm{6}}+{k}\pi\:}\\{{u}=−\mathrm{1}\Rightarrow\mathrm{cos}\:\mathrm{2}{x}=−\mathrm{1};\:\mathrm{2}{x}=\pm\pi+\mathrm{2}{k}\pi\:;\:{x}=\pm\frac{\pi}{\mathrm{2}}+{k}\pi}\end{cases} \\ $$$$ \\ $$

Commented by puissant last updated on 02/Aug/21

$$\mathrm{thanks}… \\ $$

Answered by MJS_new last updated on 02/Aug/21

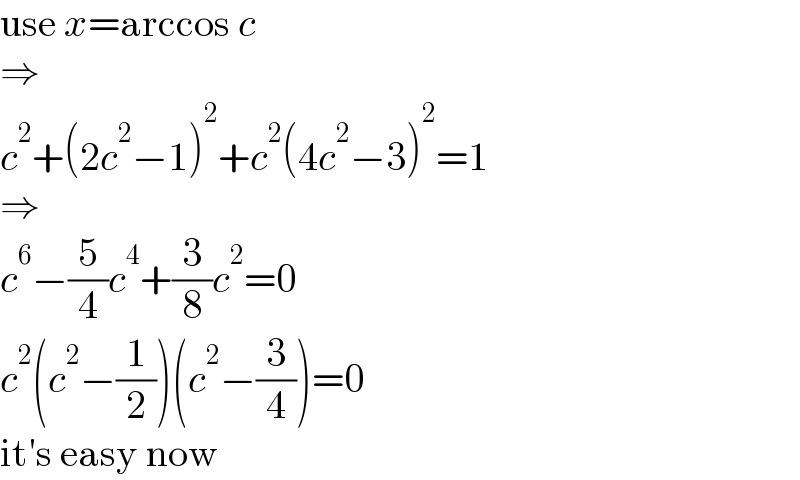
use x=arccos c ⇒ c^2 +(2c^2 −1)^2 +c^2 (4c^2 −3)^2 =1 ⇒ c^6 −(5/4)c^4 +(3/8)c^2 =0 c^2 (c^2 −(1/2))(c^2 −(3/4))=0 it′s easy now

$$\mathrm{use}\:{x}=\mathrm{arccos}\:{c} \\ $$$$\Rightarrow \\ $$$${c}^{\mathrm{2}} +\left(\mathrm{2}{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +{c}^{\mathrm{2}} \left(\mathrm{4}{c}^{\mathrm{2}} −\mathrm{3}\right)^{\mathrm{2}} =\mathrm{1} \\ $$$$\Rightarrow \\ $$$${c}^{\mathrm{6}} −\frac{\mathrm{5}}{\mathrm{4}}{c}^{\mathrm{4}} +\frac{\mathrm{3}}{\mathrm{8}}{c}^{\mathrm{2}} =\mathrm{0} \\ $$$${c}^{\mathrm{2}} \left({c}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{2}}\right)\left({c}^{\mathrm{2}} −\frac{\mathrm{3}}{\mathrm{4}}\right)=\mathrm{0} \\ $$$$\mathrm{it}'\mathrm{s}\:\mathrm{easy}\:\mathrm{now} \\ $$

Commented by puissant last updated on 02/Aug/21

$$\mathrm{thanks}.. \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply