Question Number 149809 by 0731619 last updated on 07/Aug/21

Answered by Ar Brandon last updated on 07/Aug/21
![I=∫_0 ^1 ∫_0 ^(√(1−y^2 )) cos(x^2 +y^2 )dxdy { ((x=rcosθ),(0≤θ≤π)),((y=rsinθ),(0≤r≤(√(1−sin^2 θ))=cosθ)) :} I=∫_0 ^π ∫_0 ^(cosθ) rcos(r^2 )drdθ=(1/2)∫_0 ^π ∫_0 ^(cosθ) cos(r^2 )d(r^2 )dθ =(1/2)∫_0 ^π [sin(r^2 )]_0 ^(cosθ) dθ=(1/2)∫_0 ^π sin(cos^2 θ)dθ](https://www.tinkutara.com/question/Q149811.png)
$${I}=\int_{\mathrm{0}} ^{\mathrm{1}} \int_{\mathrm{0}} ^{\sqrt{\mathrm{1}−{y}^{\mathrm{2}} }} \mathrm{cos}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right){dxdy} \\ $$$$\begin{cases}{{x}={r}\mathrm{cos}\theta}&{\mathrm{0}\leqslant\theta\leqslant\pi}\\{{y}={r}\mathrm{sin}\theta}&{\mathrm{0}\leqslant{r}\leqslant\sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \theta}=\mathrm{cos}\theta}\end{cases} \\ $$$${I}=\int_{\mathrm{0}} ^{\pi} \int_{\mathrm{0}} ^{\mathrm{cos}\theta} {r}\mathrm{cos}\left({r}^{\mathrm{2}} \right){drd}\theta=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\pi} \int_{\mathrm{0}} ^{\mathrm{cos}\theta} \mathrm{cos}\left({r}^{\mathrm{2}} \right){d}\left({r}^{\mathrm{2}} \right){d}\theta \\ $$$$\:\:=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\pi} \left[\mathrm{sin}\left({r}^{\mathrm{2}} \right)\right]_{\mathrm{0}} ^{\mathrm{cos}\theta} {d}\theta=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\pi} \mathrm{sin}\left(\mathrm{cos}^{\mathrm{2}} \theta\right){d}\theta \\ $$
Answered by Kamel last updated on 08/Aug/21
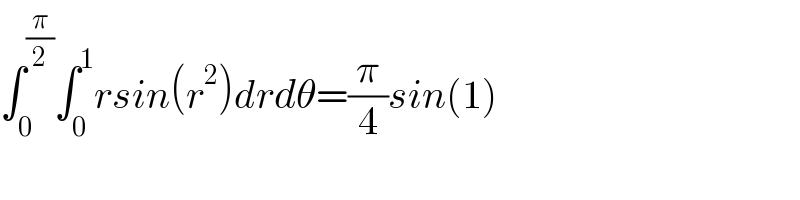
$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \int_{\mathrm{0}} ^{\mathrm{1}} {rsin}\left({r}^{\mathrm{2}} \right){drd}\theta=\frac{\pi}{\mathrm{4}}{sin}\left(\mathrm{1}\right) \\ $$
