Question Number 150410 by ajfour last updated on 12/Aug/21

Commented by ajfour last updated on 12/Aug/21

$${If}\:{length}\:{of}\:{cylinder}\:{and} \\ $$$${both}\:{width}\:{and}\:{length}\:{of} \\ $$$${wedge}\:{are}\:{equal},\:{and}\:{that} \\ $$$${their}\:{material}\:{densities} \\ $$$${are}\:{even}\:{equal},\:{and}\:{their} \\ $$$${acceleration}\:{magnitudes} \\ $$$$\left({from}\:{ground}\:{reference}\right) \\ $$$${are}\:{equal}\:{then}\:{find}\:{x}/{r}. \\ $$$$\left({assume}\:{pure}\:{rolling}\right). \\ $$
Answered by ajfour last updated on 12/Aug/21
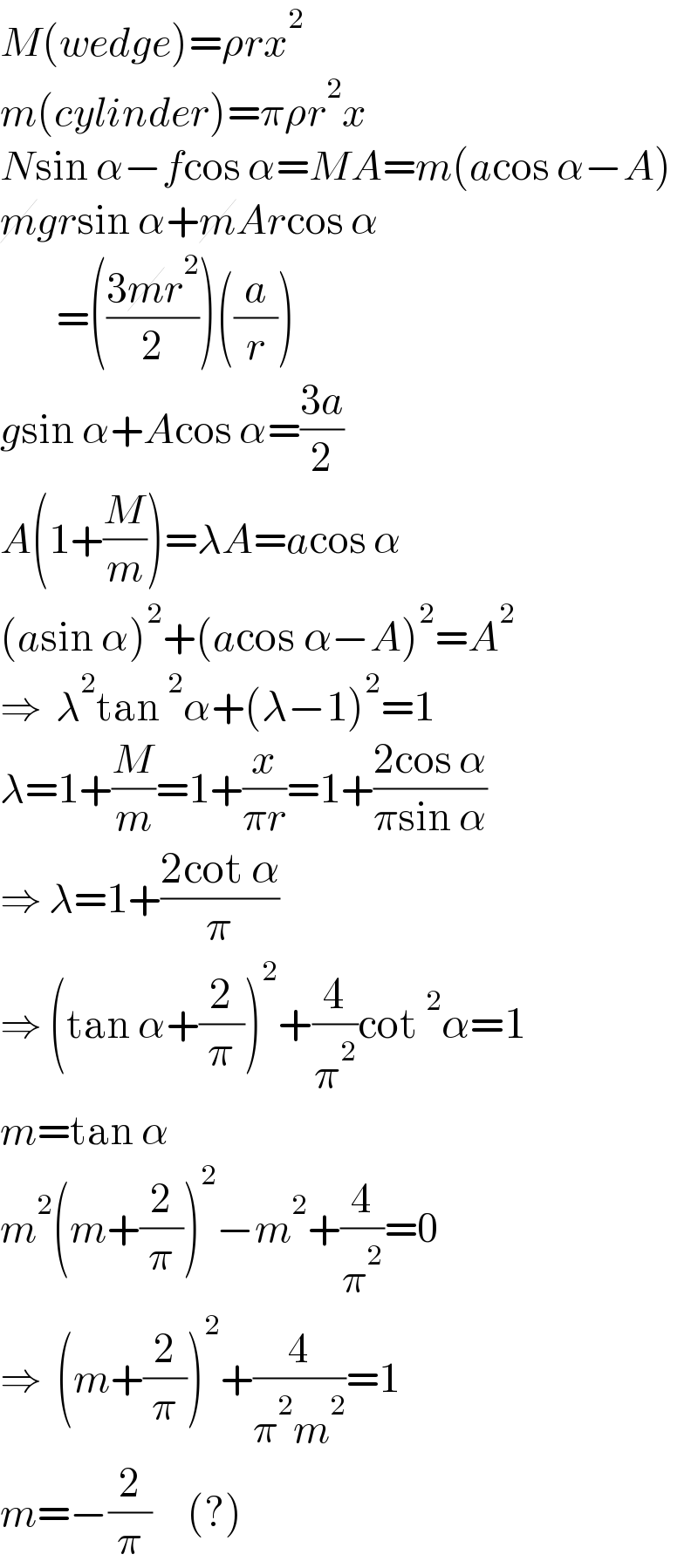
$${M}\left({wedge}\right)=\rho{rx}^{\mathrm{2}} \\ $$$${m}\left({cylinder}\right)=\pi\rho{r}^{\mathrm{2}} {x} \\ $$$${N}\mathrm{sin}\:\alpha−{f}\mathrm{cos}\:\alpha={MA}={m}\left({a}\mathrm{cos}\:\alpha−{A}\right) \\ $$$$\cancel{{m}gr}\mathrm{sin}\:\alpha+\cancel{{m}Ar}\mathrm{cos}\:\alpha \\ $$$$\:\:\:\:\:\:\:\:=\left(\frac{\mathrm{3}\cancel{{m}r}^{\mathrm{2}} }{\mathrm{2}}\right)\left(\frac{{a}}{{r}}\right) \\ $$$${g}\mathrm{sin}\:\alpha+{A}\mathrm{cos}\:\alpha=\frac{\mathrm{3}{a}}{\mathrm{2}} \\ $$$${A}\left(\mathrm{1}+\frac{{M}}{{m}}\right)=\lambda{A}={a}\mathrm{cos}\:\alpha \\ $$$$\left({a}\mathrm{sin}\:\alpha\right)^{\mathrm{2}} +\left({a}\mathrm{cos}\:\alpha−{A}\right)^{\mathrm{2}} ={A}^{\mathrm{2}} \\ $$$$\Rightarrow\:\:\lambda^{\mathrm{2}} \mathrm{tan}\:^{\mathrm{2}} \alpha+\left(\lambda−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{1} \\ $$$$\lambda=\mathrm{1}+\frac{{M}}{{m}}=\mathrm{1}+\frac{{x}}{\pi{r}}=\mathrm{1}+\frac{\mathrm{2cos}\:\alpha}{\pi\mathrm{sin}\:\alpha} \\ $$$$\Rightarrow\:\lambda=\mathrm{1}+\frac{\mathrm{2cot}\:\alpha}{\pi} \\ $$$$\Rightarrow\:\left(\mathrm{tan}\:\alpha+\frac{\mathrm{2}}{\pi}\right)^{\mathrm{2}} +\frac{\mathrm{4}}{\pi^{\mathrm{2}} }\mathrm{cot}\:^{\mathrm{2}} \alpha=\mathrm{1} \\ $$$${m}=\mathrm{tan}\:\alpha \\ $$$${m}^{\mathrm{2}} \left({m}+\frac{\mathrm{2}}{\pi}\right)^{\mathrm{2}} −{m}^{\mathrm{2}} +\frac{\mathrm{4}}{\pi^{\mathrm{2}} }=\mathrm{0} \\ $$$$\Rightarrow\:\:\left({m}+\frac{\mathrm{2}}{\pi}\right)^{\mathrm{2}} +\frac{\mathrm{4}}{\pi^{\mathrm{2}} {m}^{\mathrm{2}} }=\mathrm{1} \\ $$$${m}=−\frac{\mathrm{2}}{\pi}\:\:\:\:\:\left(?\right) \\ $$
