Question Number 150804 by mathdanisur last updated on 15/Aug/21
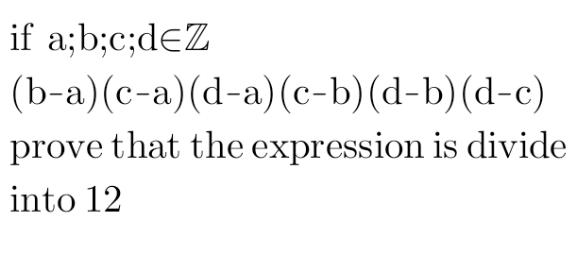
Answered by aleks041103 last updated on 15/Aug/21

$${Let}\:{P}=\left({b}−{a}\right)\left({c}−{a}\right)\left({d}−{a}\right)\left({c}−{b}\right)\left({d}−{b}\right)\left({d}−{c}\right). \\ $$$${Obviously}\:{P}\:\:{is}\:{the}\:{product}\:{of}\:{all} \\ $$$${possible}\:{differences}\:{between}\:{a},{b},{c},{d}. \\ $$$$\mathrm{1}.\:{Divisibility}\:{by}\:\mathrm{3},\:{i}.{e}.\:\mathrm{3}\mid{P}. \\ $$$${If}\:{any}\:{two}\:{of}\:{the}\:{four}\:{numbers} \\ $$$${have}\:{the}\:{same}\:{remainder}\:{when}\:{divided} \\ $$$${by}\:\mathrm{3},\:{their}\:{difference}\:{will}\:{have}\:{a} \\ $$$${remainder}\:\mathrm{0}\:{and}\:{therefore}\:{it}\:{will}\:{be}\:{divisible} \\ $$$${by}\:\mathrm{3}.\:{Therefore}\:\mathrm{3}\mid{P}. \\ $$$${Since}\:{there}\:{are}\:{exactly}\:\mathrm{3}\:{possible}\: \\ $$$${remainders}\:{and}\:{we}\:{have}\:\mathrm{4}\:{numbers}, \\ $$$${it}'{s}\:{unavoidable}\:{to}\:{have}\:{at}\:{least}\:{two} \\ $$$${numbers}\:{with}\:{the}\:{same}\:{remainder}. \\ $$$${Therefore}\:\mathrm{3}\mid{P}. \\ $$$$\mathrm{2}.\:{Divisibility}\:{by}\:\mathrm{4},\:{i}.{e}.\:\mathrm{4}\mid{P}. \\ $$$${The}\:{same}\:{argument}\:{as}\:{in}\:\mathrm{1}.\:{shows}\: \\ $$$${that}\:{if}\:\mathrm{2}\:{of}\:{the}\:\mathrm{4}\:{numbers}\:{have}\:{the} \\ $$$${same}\:{remainder}\:{when}\:{divided}\:{by}\:\mathrm{4}, \\ $$$${then}\:\mathrm{4}\mid{P}. \\ $$$${Let}\:{all}\:{of}\:{the}\:\mathrm{4}\:{numbers}\:{have}\:{different} \\ $$$${remainders}.\:{Then}\:{without}\:{any}\:{restrictions} \\ $$$${let} \\ $$$${a}=\mathrm{4}{A} \\ $$$${b}=\mathrm{4}{B}+\mathrm{1} \\ $$$${c}=\mathrm{4}{C}+\mathrm{2} \\ $$$${d}=\mathrm{4}{D}+\mathrm{3} \\ $$$${Then}: \\ $$$${P}=\left(\mathrm{4}\left({B}−{A}\right)+\mathrm{1}\right)\left(\mathrm{4}\left({C}−{A}\right)+\mathrm{2}\right)\left(\mathrm{4}\left({D}−{A}\right)+\mathrm{3}\right)\left(\mathrm{4}\left({C}−{B}\right)+\mathrm{1}\right)\left(\mathrm{4}\left({D}−{B}\right)+\mathrm{2}\right)\left(\mathrm{4}\left({D}−{C}\right)+\mathrm{1}\right) \\ $$$${But}\:\mathrm{4}\left({C}−{A}\right)+\mathrm{2}=\mathrm{2}\left(\mathrm{2}\left({C}−{A}\right)+\mathrm{1}\right)\:{and} \\ $$$$\mathrm{4}\left({D}−{B}\right)+\mathrm{2}=\mathrm{2}\left(\mathrm{2}\left({D}−{B}\right)+\mathrm{1}\right).\:{Then} \\ $$$${P}=\mathrm{4}\left(\mathrm{4}\left({B}−{A}\right)+\mathrm{1}\right)\left(\mathrm{4}\left({D}−{A}\right)+\mathrm{3}\right)\left(\mathrm{4}\left({C}−{B}\right)+\mathrm{1}\right)\left(\mathrm{4}\left({D}−{C}\right)+\mathrm{1}\right)\left(\mathrm{2}\left({C}−{A}\right)+\mathrm{1}\right)\left(\mathrm{2}\left({D}−{B}\right)+\mathrm{1}\right) \\ $$$${Therefore}\:\mathrm{4}\mid{P}. \\ $$$$\mathrm{3}.\:{Divisibility}\:{by}\:\mathrm{12}. \\ $$$${From}\:\mathrm{1}.\:{and}\:\mathrm{2}.\:{we}\:{get}: \\ $$$$\mathrm{3}\mid{P}\:{and}\:\mathrm{4}\mid{P}\:{and}\:{LCM}\left(\mathrm{3},\mathrm{4}\right)=\mathrm{12} \\ $$$$\Rightarrow\mathrm{12}\mid{P} \\ $$
Commented by mathdanisur last updated on 15/Aug/21
$$\mathrm{nice}\:\mathrm{Ser}\:\mathrm{thank}\:\mathrm{you} \\ $$
