Menu Close

Question-151248

Tinku Tara 0 Comments
Pin




Question Number 151248 by mathdanisur last updated on 19/Aug/21
Answered by EDWIN88 last updated on 19/Aug/21
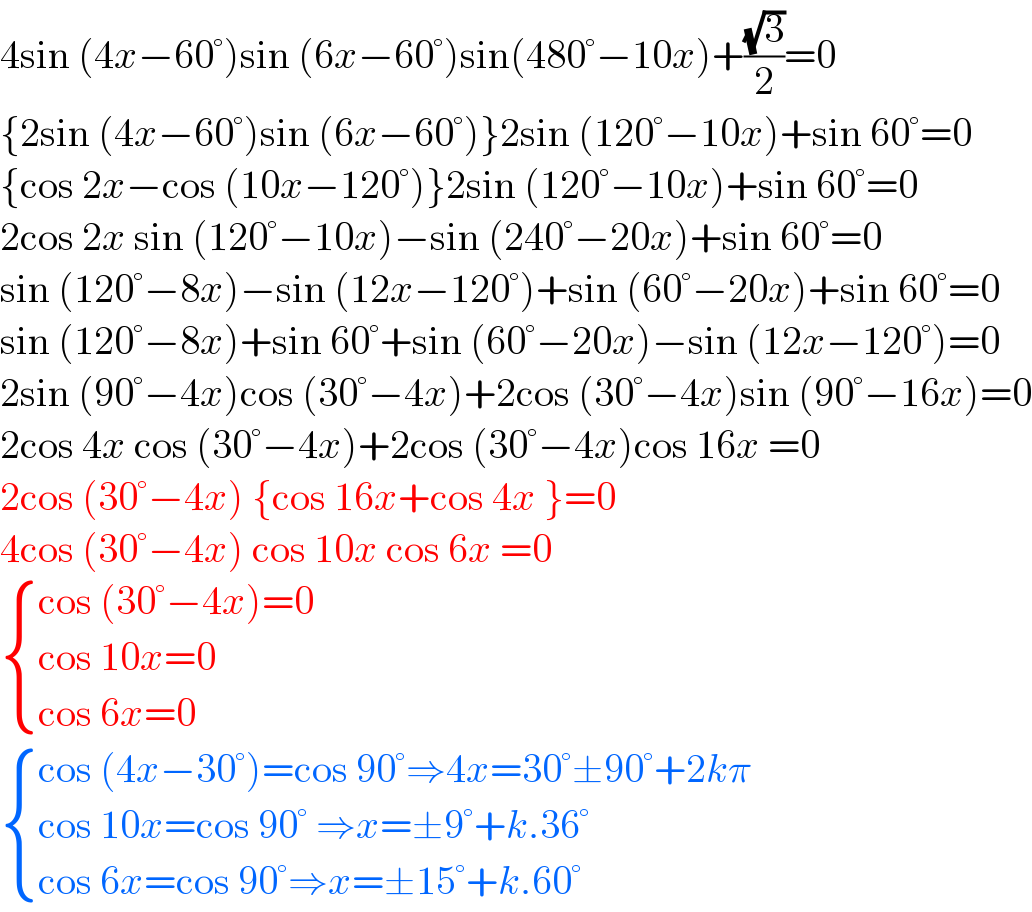
4sin (4x−60°)sin (6x−60°)sin(480°−10x)+((√3)/2)=0 {2sin (4x−60°)sin (6x−60°)}2sin (120°−10x)+sin 60°=0 {cos 2x−cos (10x−120°)}2sin (120°−10x)+sin 60°=0 2cos 2x sin (120°−10x)−sin (240°−20x)+sin 60°=0 sin (120°−8x)−sin (12x−120°)+sin (60°−20x)+sin 60°=0 sin (120°−8x)+sin 60°+sin (60°−20x)−sin (12x−120°)=0 2sin (90°−4x)cos (30°−4x)+2cos (30°−4x)sin (90°−16x)=0 2cos 4x cos (30°−4x)+2cos (30°−4x)cos 16x =0 2cos (30°−4x) {cos 16x+cos 4x }=0 4cos (30°−4x) cos 10x cos 6x =0 { ((cos (30°−4x)=0)),((cos 10x=0)),((cos 6x=0)) :} { ((cos (4x−30°)=cos 90°⇒4x=30°±90°+2kπ)),((cos 10x=cos 90° ⇒x=±9°+k.36°)),((cos 6x=cos 90°⇒x=±15°+k.60°)) :}
$$\mathrm{4sin}\:\left(\mathrm{4}{x}−\mathrm{60}°\right)\mathrm{sin}\:\left(\mathrm{6}{x}−\mathrm{60}°\right)\mathrm{sin}\left(\mathrm{480}°−\mathrm{10}{x}\right)+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=\mathrm{0} \\ $$$$\left\{\mathrm{2sin}\:\left(\mathrm{4}{x}−\mathrm{60}°\right)\mathrm{sin}\:\left(\mathrm{6}{x}−\mathrm{60}°\right)\right\}\mathrm{2sin}\:\left(\mathrm{120}°−\mathrm{10}{x}\right)+\mathrm{sin}\:\mathrm{60}°=\mathrm{0} \\ $$$$\left\{\mathrm{cos}\:\mathrm{2}{x}−\mathrm{cos}\:\left(\mathrm{10}{x}−\mathrm{120}°\right)\right\}\mathrm{2sin}\:\left(\mathrm{120}°−\mathrm{10}{x}\right)+\mathrm{sin}\:\mathrm{60}°=\mathrm{0} \\ $$$$\mathrm{2cos}\:\mathrm{2}{x}\:\mathrm{sin}\:\left(\mathrm{120}°−\mathrm{10}{x}\right)−\mathrm{sin}\:\left(\mathrm{240}°−\mathrm{20}{x}\right)+\mathrm{sin}\:\mathrm{60}°=\mathrm{0} \\ $$$$\mathrm{sin}\:\left(\mathrm{120}°−\mathrm{8}{x}\right)−\mathrm{sin}\:\left(\mathrm{12}{x}−\mathrm{120}°\right)+\mathrm{sin}\:\left(\mathrm{60}°−\mathrm{20}{x}\right)+\mathrm{sin}\:\mathrm{60}°=\mathrm{0} \\ $$$$\mathrm{sin}\:\left(\mathrm{120}°−\mathrm{8}{x}\right)+\mathrm{sin}\:\mathrm{60}°+\mathrm{sin}\:\left(\mathrm{60}°−\mathrm{20}{x}\right)−\mathrm{sin}\:\left(\mathrm{12}{x}−\mathrm{120}°\right)=\mathrm{0} \\ $$$$\mathrm{2sin}\:\left(\mathrm{90}°−\mathrm{4}{x}\right)\mathrm{cos}\:\left(\mathrm{30}°−\mathrm{4}{x}\right)+\mathrm{2cos}\:\left(\mathrm{30}°−\mathrm{4}{x}\right)\mathrm{sin}\:\left(\mathrm{90}°−\mathrm{16}{x}\right)=\mathrm{0} \\ $$$$\mathrm{2cos}\:\mathrm{4}{x}\:\mathrm{cos}\:\left(\mathrm{30}°−\mathrm{4}{x}\right)+\mathrm{2cos}\:\left(\mathrm{30}°−\mathrm{4}{x}\right)\mathrm{cos}\:\mathrm{16}{x}\:=\mathrm{0} \\ $$$$\mathrm{2cos}\:\left(\mathrm{30}°−\mathrm{4}{x}\right)\:\left\{\mathrm{cos}\:\mathrm{16}{x}+\mathrm{cos}\:\mathrm{4}{x}\:\right\}=\mathrm{0} \\ $$$$\mathrm{4cos}\:\left(\mathrm{30}°−\mathrm{4}{x}\right)\:\mathrm{cos}\:\mathrm{10}{x}\:\mathrm{cos}\:\mathrm{6}{x}\:=\mathrm{0} \\ $$$$\begin{cases}{\mathrm{cos}\:\left(\mathrm{30}°−\mathrm{4}{x}\right)=\mathrm{0}}\\{\mathrm{cos}\:\mathrm{10}{x}=\mathrm{0}}\\{\mathrm{cos}\:\mathrm{6}{x}=\mathrm{0}}\end{cases} \\ $$$$\begin{cases}{\mathrm{cos}\:\left(\mathrm{4}{x}−\mathrm{30}°\right)=\mathrm{cos}\:\mathrm{90}°\Rightarrow\mathrm{4}{x}=\mathrm{30}°\pm\mathrm{90}°+\mathrm{2}{k}\pi}\\{\mathrm{cos}\:\mathrm{10}{x}=\mathrm{cos}\:\mathrm{90}°\:\Rightarrow{x}=\pm\mathrm{9}°+{k}.\mathrm{36}°}\\{\mathrm{cos}\:\mathrm{6}{x}=\mathrm{cos}\:\mathrm{90}°\Rightarrow{x}=\pm\mathrm{15}°+{k}.\mathrm{60}°}\end{cases} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *