Menu Close

Question-151641

Tinku Tara 0 Comments
Pin




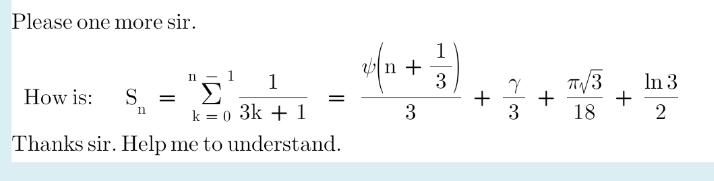
Question Number 151641 by Tawa11 last updated on 22/Aug/21
Answered by Kamel last updated on 22/Aug/21
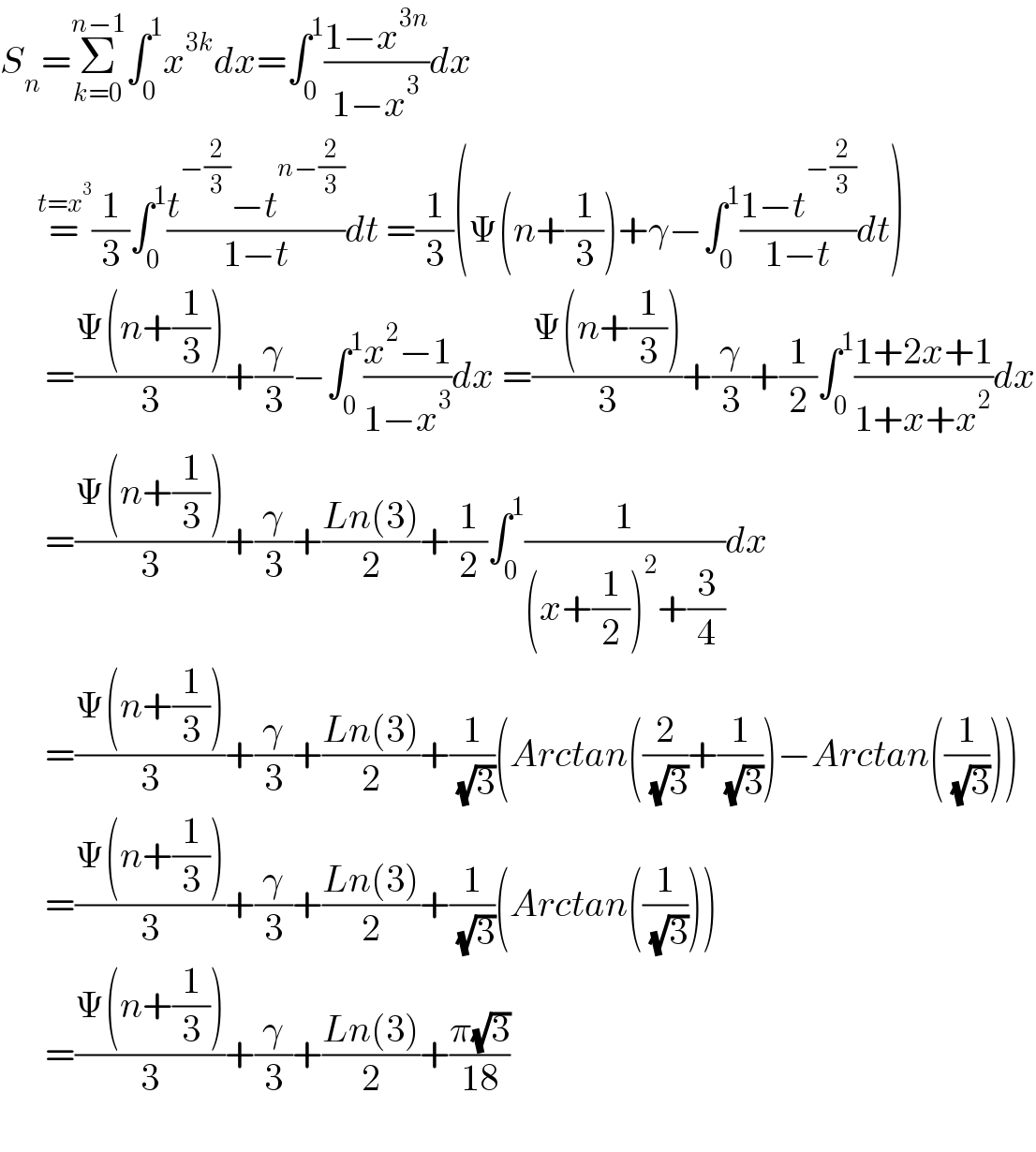
S_n =Σ_(k=0) ^(n−1) ∫_0 ^1 x^(3k) dx=∫_0 ^1 ((1−x^(3n) )/(1−x^3 ))dx =^(t=x^3 ) (1/3)∫_0 ^1 ((t^(−(2/3)) −t^(n−(2/3)) )/(1−t))dt =(1/3)(Ψ(n+(1/3))+γ−∫_0 ^1 ((1−t^(−(2/3)) )/(1−t))dt) =((Ψ(n+(1/3)))/3)+(γ/3)−∫_0 ^1 ((x^2 −1)/(1−x^3 ))dx =((Ψ(n+(1/3)))/3)+(γ/3)+(1/2)∫_0 ^1 ((1+2x+1)/(1+x+x^2 ))dx =((Ψ(n+(1/3)))/3)+(γ/3)+((Ln(3))/2)+(1/2)∫_0 ^1 (1/((x+(1/2))^2 +(3/4)))dx =((Ψ(n+(1/3)))/3)+(γ/3)+((Ln(3))/2)+(1/( (√3)))(Arctan((2/( (√3)))+(1/( (√3))))−Arctan((1/( (√3))))) =((Ψ(n+(1/3)))/3)+(γ/3)+((Ln(3))/2)+(1/( (√3)))(Arctan((1/( (√3))))) =((Ψ(n+(1/3)))/3)+(γ/3)+((Ln(3))/2)+((π(√3))/(18))
$${S}_{{n}} =\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}\int_{\mathrm{0}} ^{\mathrm{1}} {x}^{\mathrm{3}{k}} {dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}−{x}^{\mathrm{3}{n}} }{\mathrm{1}−{x}^{\mathrm{3}} }{dx} \\ $$$$\:\:\:\:\:\overset{{t}={x}^{\mathrm{3}} } {=}\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{t}^{−\frac{\mathrm{2}}{\mathrm{3}}} −{t}^{{n}−\frac{\mathrm{2}}{\mathrm{3}}} }{\mathrm{1}−{t}}{dt}\:=\frac{\mathrm{1}}{\mathrm{3}}\left(\Psi\left({n}+\frac{\mathrm{1}}{\mathrm{3}}\right)+\gamma−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}−{t}^{−\frac{\mathrm{2}}{\mathrm{3}}} }{\mathrm{1}−{t}}{dt}\right) \\ $$$$\:\:\:\:\:\:=\frac{\Psi\left({n}+\frac{\mathrm{1}}{\mathrm{3}}\right)}{\mathrm{3}}+\frac{\gamma}{\mathrm{3}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} −\mathrm{1}}{\mathrm{1}−{x}^{\mathrm{3}} }{dx}\:=\frac{\Psi\left({n}+\frac{\mathrm{1}}{\mathrm{3}}\right)}{\mathrm{3}}+\frac{\gamma}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}+\mathrm{2}{x}+\mathrm{1}}{\mathrm{1}+{x}+{x}^{\mathrm{2}} }{dx} \\ $$$$\:\:\:\:\:\:=\frac{\Psi\left({n}+\frac{\mathrm{1}}{\mathrm{3}}\right)}{\mathrm{3}}+\frac{\gamma}{\mathrm{3}}+\frac{{Ln}\left(\mathrm{3}\right)}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}}{dx} \\ $$$$\:\:\:\:\:\:=\frac{\Psi\left({n}+\frac{\mathrm{1}}{\mathrm{3}}\right)}{\mathrm{3}}+\frac{\gamma}{\mathrm{3}}+\frac{{Ln}\left(\mathrm{3}\right)}{\mathrm{2}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\left({Arctan}\left(\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right)−{Arctan}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right)\right) \\ $$$$\:\:\:\:\:\:=\frac{\Psi\left({n}+\frac{\mathrm{1}}{\mathrm{3}}\right)}{\mathrm{3}}+\frac{\gamma}{\mathrm{3}}+\frac{{Ln}\left(\mathrm{3}\right)}{\mathrm{2}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\left({Arctan}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right)\right) \\ $$$$\:\:\:\:\:\:=\frac{\Psi\left({n}+\frac{\mathrm{1}}{\mathrm{3}}\right)}{\mathrm{3}}+\frac{\gamma}{\mathrm{3}}+\frac{{Ln}\left(\mathrm{3}\right)}{\mathrm{2}}+\frac{\pi\sqrt{\mathrm{3}}}{\mathrm{18}} \\ $$$$\:\:\:\:\: \\ $$
Commented by Tawa11 last updated on 22/Aug/21
Wow. God bless you sir. I appreciate.
$$\mathrm{Wow}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{appreciate}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *