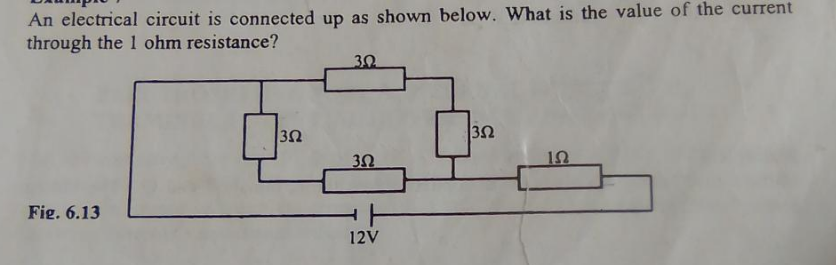
Question Number 151677 by Tawa11 last updated on 22/Aug/21
Answered by Olaf_Thorendsen last updated on 22/Aug/21
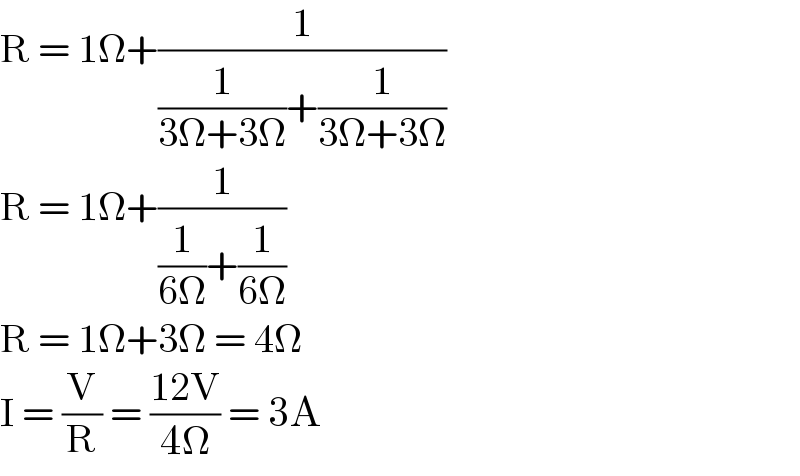
$$\mathrm{R}\:=\:\mathrm{1}\Omega+\frac{\mathrm{1}}{\frac{\mathrm{1}}{\mathrm{3}\Omega+\mathrm{3}\Omega}+\frac{\mathrm{1}}{\mathrm{3}\Omega+\mathrm{3}\Omega}} \\ $$$$\mathrm{R}\:=\:\mathrm{1}\Omega+\frac{\mathrm{1}}{\frac{\mathrm{1}}{\mathrm{6}\Omega}+\frac{\mathrm{1}}{\mathrm{6}\Omega}} \\ $$$$\mathrm{R}\:=\:\mathrm{1}\Omega+\mathrm{3}\Omega\:=\:\mathrm{4}\Omega \\ $$$$\mathrm{I}\:=\:\frac{\mathrm{V}}{\mathrm{R}}\:=\:\frac{\mathrm{12V}}{\mathrm{4}\Omega}\:=\:\mathrm{3A} \\ $$
Commented by Tawa11 last updated on 22/Aug/21
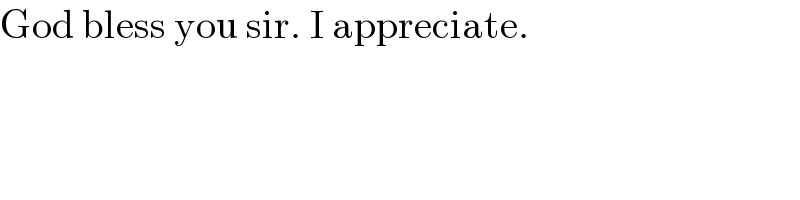
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{appreciate}. \\ $$
Commented by Olaf_Thorendsen last updated on 22/Aug/21

$$\mathrm{It}'\mathrm{s}\:\mathrm{funny}\:\mathrm{because}\:\mathrm{we}\:\mathrm{have}\:\mathrm{a} \\ $$$$\mathrm{resistance}\:\mathrm{of}\:\mathrm{3}\Omega\:\mathrm{by}\:\mathrm{using}\:\mathrm{four} \\ $$$$\mathrm{resistances}\:\mathrm{of}\:\mathrm{3}\Omega. \\ $$$$\mathrm{In}\:\mathrm{the}\:\mathrm{real}\:\mathrm{life},\:\mathrm{only}\:\mathrm{one}\:\mathrm{resistance} \\ $$$$\mathrm{of}\:\mathrm{3}\Omega\:\mathrm{is}\:\mathrm{enough}\:\mathrm{to}\:\mathrm{obtain}\:\mathrm{3}\Omega. \\ $$
Commented by Tawa11 last updated on 22/Aug/21

$$\mathrm{Ohh}.\:\mathrm{Thanks}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{understand}\:\mathrm{better}. \\ $$
