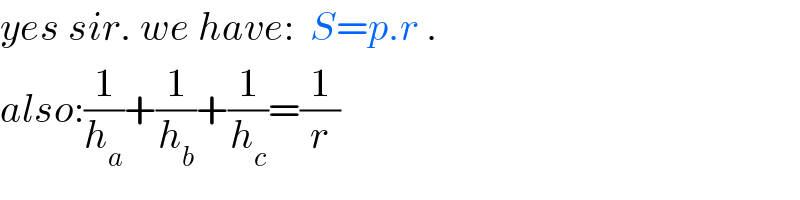Question Number 15212 by ajfour last updated on 08/Jun/17
Commented by ajfour last updated on 08/Jun/17

Answered by ajfour last updated on 08/Jun/17
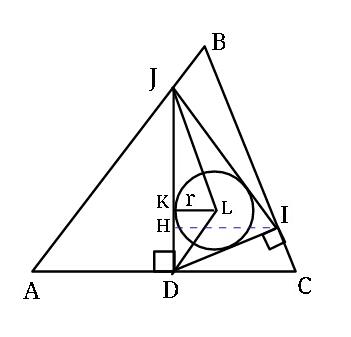
![CD=(b/2), AD=(b/2) ∠HDI+∠CDI=(π/2) ∠HDI+(π/2)−∠C=(π/2) ⇒∠HDI=∠C ∠HDL=(C/2) HD =(b/2)sin Ccos C HI=(b/2)sin^2 C JD=(b/2)tan A , JH=JD−HD JH=(b/2)(tan A−sin Ccos C) ∠IJH=𝛂 , tan α=((HI)/(JH)) tan α=((sin^2 C)/(tan A−sin Ccos C)) ....(i) ∠KJL=(α/2) JK+KD=JD (r/(tan (𝛂/2)))+(r/(tan (C/2)))=(b/2)tan A so, r=(b/2)tan A[((tan (𝛂/2)tan (C/2))/(tan (𝛂/2)+tan (C/2)))] ......... .......... ......... cos A=((b^2 +c^2 −a^2 )/(2bc))=((225+196−169)/(2×15×14)) cos A=(3/5) ⇒ tan A=(4/3) cos C=((a^2 +b^2 −c^2 )/(2ab))=((169+225−196)/(2×13×15)) =((33)/(65)). ⇒ sin C=((56)/(65)) and tan C=((56)/(33)) tan α=((sin^2 C)/(tan A−sin Ccos C)) =(((56/65)^2 )/((4/3)−(56/65)(33/65))) tan α= 0.8285 ⇒ α=0.692rad C=tan^(−1) (56/33)=1.0383 r=(((b/2)tan A)/([(1/(tan (α/2)))+(1/(tan (C/2))])) = (((((15)/2)×(4/3)))/([(1/(tan (0.346)))+(1/(tan (0.519)))])) = ((10)/((2.774+1.75)))= 2.21 units •](https://www.tinkutara.com/question/Q15218.png)
Commented by mrW1 last updated on 08/Jun/17
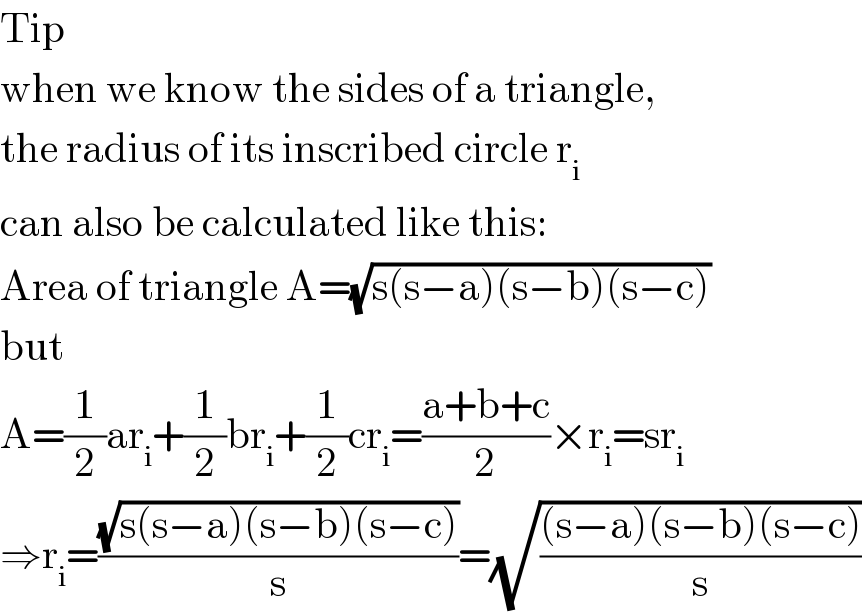
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 08/Jun/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 09/Jun/17