Question Number 152371 by fotosy2k last updated on 27/Aug/21

Commented by fotosy2k last updated on 27/Aug/21

$${pls}\:{belp} \\ $$$$ \\ $$
Answered by Olaf_Thorendsen last updated on 27/Aug/21

$$\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\frac{{x}−\mathrm{2}}{{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}}\:=\:\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}\:}\frac{{x}−\mathrm{2}}{\left({x}−\mathrm{2}\right)\left({x}−\mathrm{1}\right)} \\ $$$$=\:\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\frac{\mathrm{1}}{{x}−\mathrm{1}}\:=\:\mathrm{1} \\ $$
Commented by fotosy2k last updated on 27/Aug/21

$${thank}\:{you} \\ $$
Answered by Rasheed.Sindhi last updated on 28/Aug/21
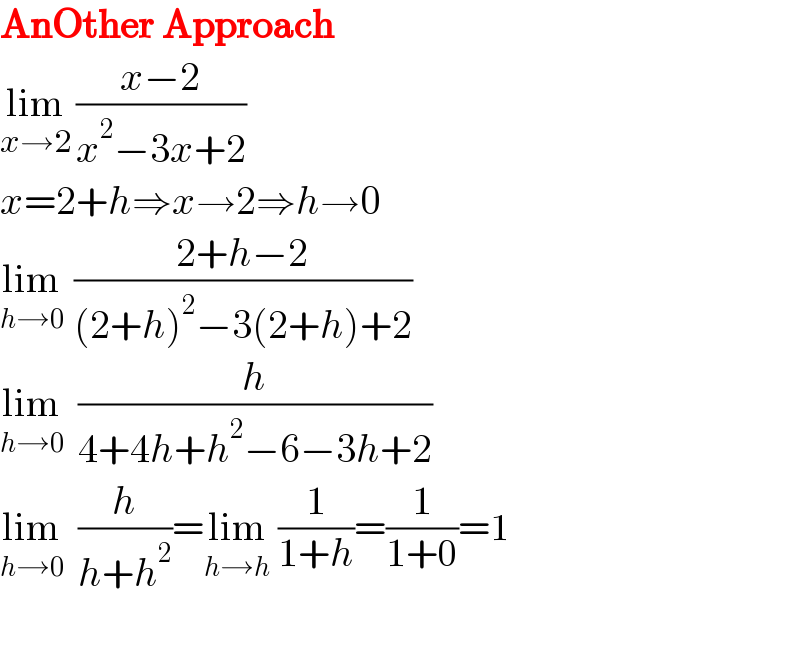
$$\boldsymbol{\mathrm{AnOther}}\:\boldsymbol{\mathrm{Approach}} \\ $$$$\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\:\frac{{x}−\mathrm{2}}{{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}} \\ $$$${x}=\mathrm{2}+{h}\Rightarrow{x}\rightarrow\mathrm{2}\Rightarrow{h}\rightarrow\mathrm{0} \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\:\frac{\mathrm{2}+{h}−\mathrm{2}}{\left(\mathrm{2}+{h}\right)^{\mathrm{2}} −\mathrm{3}\left(\mathrm{2}+{h}\right)+\mathrm{2}} \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\:\frac{{h}}{\mathrm{4}+\mathrm{4}{h}+{h}^{\mathrm{2}} −\mathrm{6}−\mathrm{3}{h}+\mathrm{2}} \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\:\frac{{h}}{{h}+{h}^{\mathrm{2}} }=\underset{{h}\rightarrow{h}} {\mathrm{lim}}\:\frac{\mathrm{1}}{\mathrm{1}+{h}}=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{0}}=\mathrm{1} \\ $$$$ \\ $$
