Question Number 152450 by fotosy2k last updated on 28/Aug/21

Commented by fotosy2k last updated on 28/Aug/21

$${pls}\:{help} \\ $$
Answered by JDamian last updated on 28/Aug/21
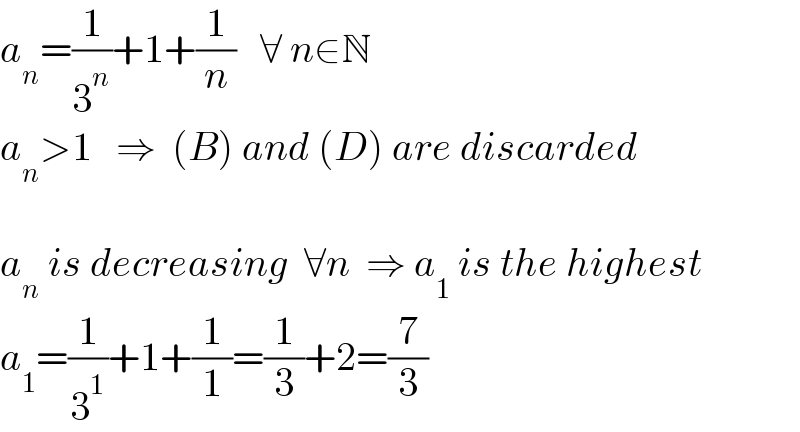
$${a}_{{n}} =\frac{\mathrm{1}}{\mathrm{3}^{{n}} }+\mathrm{1}+\frac{\mathrm{1}}{{n}}\:\:\:\forall\:{n}\in\mathbb{N} \\ $$$${a}_{{n}} >\mathrm{1}\:\:\:\Rightarrow\:\:\left({B}\right)\:{and}\:\left({D}\right)\:{are}\:{discarded} \\ $$$$ \\ $$$${a}_{{n}} \:{is}\:{decreasing}\:\:\forall{n}\:\:\Rightarrow\:{a}_{\mathrm{1}} \:{is}\:{the}\:{highest} \\ $$$${a}_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{1}} }+\mathrm{1}+\frac{\mathrm{1}}{\mathrm{1}}=\frac{\mathrm{1}}{\mathrm{3}}+\mathrm{2}=\frac{\mathrm{7}}{\mathrm{3}} \\ $$
Commented by fotosy2k last updated on 30/Aug/21

$${thank}\:{you} \\ $$
