Menu Close

Question-153189

Tinku Tara 0 Comments
Pin




Question Number 153189 by liberty last updated on 05/Sep/21
Answered by EDWIN88 last updated on 05/Sep/21
eq of circle 16x^2 +16y^2 +48x−8y−43= with center point { ((x=−((48)/(32))=−(3/2))),((y=(8/(32))=(1/4))) :} with radius =(√((9/4)+(1/6)−(((−43)/(16))))) ⇒r=(√((36+1+43)/(16))) =((4(√5))/4)=(√5) let P((√5)cos θ−(3/2), (√5)sin θ+(1/4)) the distance point P to line r ⇒d=((∣8(√5)cos θ−12−4((√5)sin θ+(1/4))+73∣)/( (√(64+16)))) ⇒d=((∣8(√5) cos θ−4(√5)sin θ+60∣)/(4(√5))) minimum λ=8(√5) cos θ−4(√5)sin θ+60 is −(√(64×5+16×5)) +60=40 d_(min) =((40)/(4(√5))) = ((10)/( (√5)))=2(√5) when cos (θ+tan^(−1) ((1/2)))=−1 θ=π−tan^(−1) ((1/2))→ { ((cos θ=−tan^(−1) ((1/2))=−0.45)),((sin θ=tan^(−1) ((1/2))=0.45)) :}
$$\:{eq}\:{of}\:{circle}\:\mathrm{16}{x}^{\mathrm{2}} +\mathrm{16}{y}^{\mathrm{2}} +\mathrm{48}{x}−\mathrm{8}{y}−\mathrm{43}= \\ $$$${with}\:{center}\:{point}\:\begin{cases}{{x}=−\frac{\mathrm{48}}{\mathrm{32}}=−\frac{\mathrm{3}}{\mathrm{2}}}\\{{y}=\frac{\mathrm{8}}{\mathrm{32}}=\frac{\mathrm{1}}{\mathrm{4}}}\end{cases} \\ $$$${with}\:{radius}\:=\sqrt{\frac{\mathrm{9}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{6}}−\left(\frac{−\mathrm{43}}{\mathrm{16}}\right)} \\ $$$$\Rightarrow{r}=\sqrt{\frac{\mathrm{36}+\mathrm{1}+\mathrm{43}}{\mathrm{16}}}\:=\frac{\mathrm{4}\sqrt{\mathrm{5}}}{\mathrm{4}}=\sqrt{\mathrm{5}}\: \\ $$$${let}\:{P}\left(\sqrt{\mathrm{5}}\mathrm{cos}\:\:\theta−\frac{\mathrm{3}}{\mathrm{2}},\:\sqrt{\mathrm{5}}\mathrm{sin}\:\theta+\frac{\mathrm{1}}{\mathrm{4}}\right) \\ $$$${the}\:{distance}\:{point}\:{P}\:{to}\:{line}\:{r} \\ $$$$\Rightarrow{d}=\frac{\mid\mathrm{8}\sqrt{\mathrm{5}}\mathrm{cos}\:\theta−\mathrm{12}−\mathrm{4}\left(\sqrt{\mathrm{5}}\mathrm{sin}\:\theta+\frac{\mathrm{1}}{\mathrm{4}}\right)+\mathrm{73}\mid}{\:\sqrt{\mathrm{64}+\mathrm{16}}} \\ $$$$\Rightarrow{d}=\frac{\mid\mathrm{8}\sqrt{\mathrm{5}}\:\mathrm{cos}\:\theta−\mathrm{4}\sqrt{\mathrm{5}}\mathrm{sin}\:\theta+\mathrm{60}\mid}{\mathrm{4}\sqrt{\mathrm{5}}} \\ $$$${minimum}\:\lambda=\mathrm{8}\sqrt{\mathrm{5}}\:\mathrm{cos}\:\theta−\mathrm{4}\sqrt{\mathrm{5}}\mathrm{sin}\:\theta+\mathrm{60} \\ $$$${is}\:−\sqrt{\mathrm{64}×\mathrm{5}+\mathrm{16}×\mathrm{5}}\:+\mathrm{60}=\mathrm{40} \\ $$$${d}_{{min}} =\frac{\mathrm{40}}{\mathrm{4}\sqrt{\mathrm{5}}}\:=\:\frac{\mathrm{10}}{\:\sqrt{\mathrm{5}}}=\mathrm{2}\sqrt{\mathrm{5}} \\ $$$${when}\:\mathrm{cos}\:\left(\theta+\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)=−\mathrm{1} \\ $$$$\theta=\pi−\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\rightarrow\begin{cases}{\mathrm{cos}\:\theta=−\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)=−\mathrm{0}.\mathrm{45}}\\{\mathrm{sin}\:\theta=\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)=\mathrm{0}.\mathrm{45}}\end{cases} \\ $$
Commented by EDWIN88 last updated on 05/Sep/21
in other way d_(min) = d[(−(3/2),(1/4)),8x−4y+73=0]−radius d[(−(3/2),(1/4)),8x−4y+73=0]= ((∣8(−(3/2))−4((1/4))+73∣)/(4(√5))) = ((60)/(4(√5))) =((15)/( (√5)))=3(√5) d_(min) = 3(√5)−(√5) =2(√5)
$${in}\:{other}\:{way}\: \\ $$$${d}_{{min}} =\:{d}\left[\left(−\frac{\mathrm{3}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{4}}\right),\mathrm{8}{x}−\mathrm{4}{y}+\mathrm{73}=\mathrm{0}\right]−{radius} \\ $$$${d}\left[\left(−\frac{\mathrm{3}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{4}}\right),\mathrm{8}{x}−\mathrm{4}{y}+\mathrm{73}=\mathrm{0}\right]= \\ $$$$\:\frac{\mid\mathrm{8}\left(−\frac{\mathrm{3}}{\mathrm{2}}\right)−\mathrm{4}\left(\frac{\mathrm{1}}{\mathrm{4}}\right)+\mathrm{73}\mid}{\mathrm{4}\sqrt{\mathrm{5}}}\:=\:\frac{\mathrm{60}}{\mathrm{4}\sqrt{\mathrm{5}}}\:=\frac{\mathrm{15}}{\:\sqrt{\mathrm{5}}}=\mathrm{3}\sqrt{\mathrm{5}} \\ $$$${d}_{{min}} =\:\mathrm{3}\sqrt{\mathrm{5}}−\sqrt{\mathrm{5}}\:=\mathrm{2}\sqrt{\mathrm{5}}\: \\ $$
Answered by mr W last updated on 05/Sep/21
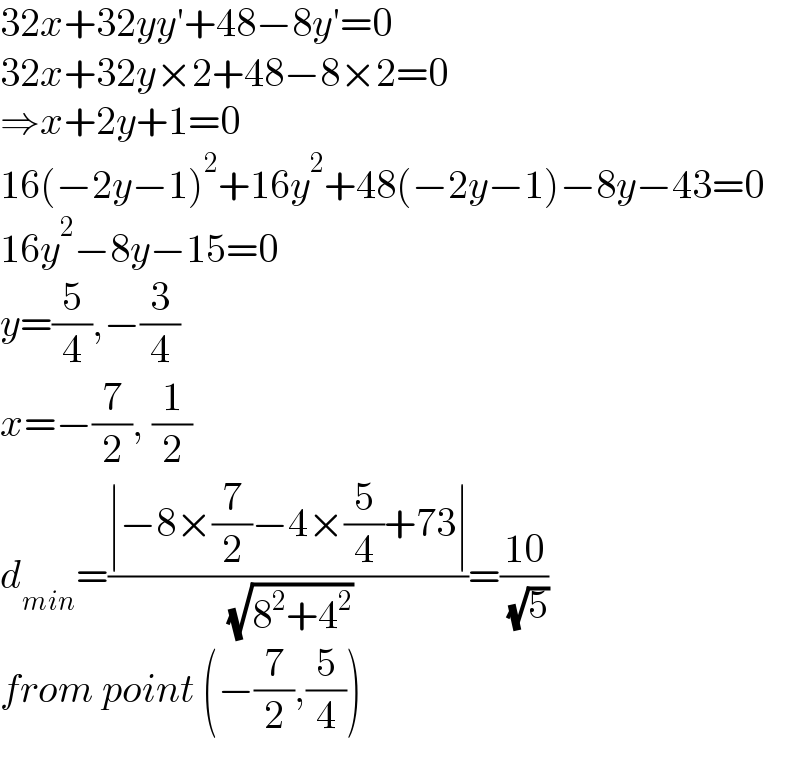
32x+32yy′+48−8y′=0 32x+32y×2+48−8×2=0 ⇒x+2y+1=0 16(−2y−1)^2 +16y^2 +48(−2y−1)−8y−43=0 16y^2 −8y−15=0 y=(5/4),−(3/4) x=−(7/2), (1/2) d_(min) =((∣−8×(7/2)−4×(5/4)+73∣)/( (√(8^2 +4^2 ))))=((10)/( (√5))) from point (−(7/2),(5/4))
$$\mathrm{32}{x}+\mathrm{32}{yy}'+\mathrm{48}−\mathrm{8}{y}'=\mathrm{0} \\ $$$$\mathrm{32}{x}+\mathrm{32}{y}×\mathrm{2}+\mathrm{48}−\mathrm{8}×\mathrm{2}=\mathrm{0} \\ $$$$\Rightarrow{x}+\mathrm{2}{y}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{16}\left(−\mathrm{2}{y}−\mathrm{1}\right)^{\mathrm{2}} +\mathrm{16}{y}^{\mathrm{2}} +\mathrm{48}\left(−\mathrm{2}{y}−\mathrm{1}\right)−\mathrm{8}{y}−\mathrm{43}=\mathrm{0} \\ $$$$\mathrm{16}{y}^{\mathrm{2}} −\mathrm{8}{y}−\mathrm{15}=\mathrm{0} \\ $$$${y}=\frac{\mathrm{5}}{\mathrm{4}},−\frac{\mathrm{3}}{\mathrm{4}} \\ $$$${x}=−\frac{\mathrm{7}}{\mathrm{2}},\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${d}_{{min}} =\frac{\mid−\mathrm{8}×\frac{\mathrm{7}}{\mathrm{2}}−\mathrm{4}×\frac{\mathrm{5}}{\mathrm{4}}+\mathrm{73}\mid}{\:\sqrt{\mathrm{8}^{\mathrm{2}} +\mathrm{4}^{\mathrm{2}} }}=\frac{\mathrm{10}}{\:\sqrt{\mathrm{5}}} \\ $$$${from}\:{point}\:\left(−\frac{\mathrm{7}}{\mathrm{2}},\frac{\mathrm{5}}{\mathrm{4}}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *