Question Number 153249 by SANOGO last updated on 06/Sep/21

Answered by qaz last updated on 06/Sep/21
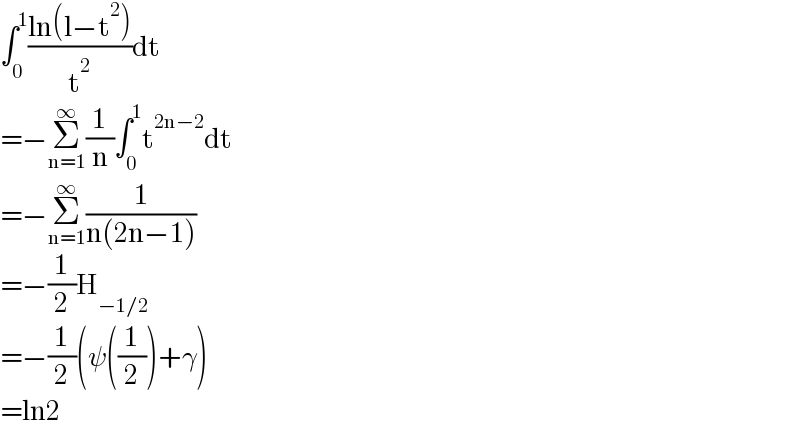
$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{l}−\mathrm{t}^{\mathrm{2}} \right)}{\mathrm{t}^{\mathrm{2}} }\mathrm{dt} \\ $$$$=−\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{n}}\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{t}^{\mathrm{2n}−\mathrm{2}} \mathrm{dt} \\ $$$$=−\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{n}\left(\mathrm{2n}−\mathrm{1}\right)} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{H}_{−\mathrm{1}/\mathrm{2}} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\left(\psi\left(\frac{\mathrm{1}}{\mathrm{2}}\right)+\gamma\right) \\ $$$$=\mathrm{ln2} \\ $$
Commented by SANOGO last updated on 06/Sep/21
$${merci}\:{beaucoup}\:{mon}\:{doyen} \\ $$
