Question Number 153896 by SANOGO last updated on 11/Sep/21
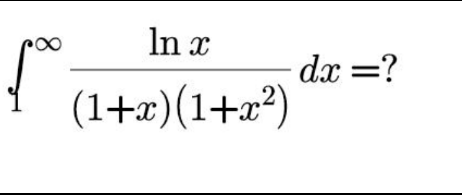
Answered by ARUNG_Brandon_MBU last updated on 12/Sep/21
![Ω=∫_1 ^∞ ((lnx)/((1+x)(1+x^2 )))dx, x=(1/u)⇒dx=−(1/u^2 )du =−∫_0 ^1 ((ulnu)/((u+1)(u^2 +1)))du (u/((u+1)(u^2 +1)))=(a/(u+1))+((bu+c)/(u^2 +1))=((a(u^2 +1)+(bu+c)(u+1))/((u+1)(u^2 +1))) { ((a+b=0)),((b+c=1)),((a+c=0)) :}⇒a=−(1/2), b=(1/2), c=(1/2) Ω=(1/2)∫_0 ^1 ((1/(u+1))−(u/(u^2 +1))−(1/(u^2 +1)))lnudu =(1/2)[∫_0 ^1 ((lnu)/(u+1))du−(1/4)∫_0 ^1 ((lnv)/(v+1))dv−(1/4)∫_0 ^1 ((v^(−(1/2)) lnv)/(v+1))dv] =(1/2)∫_0 ^1 (((1−u)lnu)/(1−u^2 ))du−(1/8)∫_0 ^1 (((1−v)lnv)/(1−v^2 ))dv−(1/8)∫_0 ^1 ((v^(−(1/2)) (1−v)lnv)/(1−v^2 ))dv =(1/8)∫_0 ^1 (((t^(−(1/2)) −1)lnt)/(1−t))dt−(1/(32))∫_0 ^1 (((t^(−(1/2)) −1)lnt)/(1−t))dt−(1/(32))∫_0 ^1 (((t^(−(1/4)) −t^(1/4) )lnt)/(1−t))dt =(1/8)(ψ′(1)−ψ′((1/2)))−(1/(32))(ψ′(1)−ψ′((1/2)))−(1/(32))(ψ′((5/4))−ψ′((3/4))) =(1/8)(ζ(2)−3ζ(2))−(1/(32))(ζ(2)−3ζ(2))−(1/(32))(ψ′((1/4))−ψ′((3/4))−16) =−(3/(16))ζ(2)−(1/(32))(2ψ′((1/4))−π^2 cosec^2 ((π/4)))+(1/2)](https://www.tinkutara.com/question/Q153904.png)
$$\Omega=\int_{\mathrm{1}} ^{\infty} \frac{\mathrm{ln}{x}}{\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{dx},\:{x}=\frac{\mathrm{1}}{{u}}\Rightarrow{dx}=−\frac{\mathrm{1}}{{u}^{\mathrm{2}} }{du} \\ $$$$\:\:\:\:=−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{u}\mathrm{ln}{u}}{\left({u}+\mathrm{1}\right)\left({u}^{\mathrm{2}} +\mathrm{1}\right)}{du} \\ $$$$\frac{{u}}{\left({u}+\mathrm{1}\right)\left({u}^{\mathrm{2}} +\mathrm{1}\right)}=\frac{{a}}{{u}+\mathrm{1}}+\frac{{bu}+{c}}{{u}^{\mathrm{2}} +\mathrm{1}}=\frac{{a}\left({u}^{\mathrm{2}} +\mathrm{1}\right)+\left({bu}+{c}\right)\left({u}+\mathrm{1}\right)}{\left({u}+\mathrm{1}\right)\left({u}^{\mathrm{2}} +\mathrm{1}\right)} \\ $$$$\begin{cases}{{a}+{b}=\mathrm{0}}\\{{b}+{c}=\mathrm{1}}\\{{a}+{c}=\mathrm{0}}\end{cases}\Rightarrow{a}=−\frac{\mathrm{1}}{\mathrm{2}},\:{b}=\frac{\mathrm{1}}{\mathrm{2}},\:{c}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Omega=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \left(\frac{\mathrm{1}}{{u}+\mathrm{1}}−\frac{{u}}{{u}^{\mathrm{2}} +\mathrm{1}}−\frac{\mathrm{1}}{{u}^{\mathrm{2}} +\mathrm{1}}\right)\mathrm{ln}{udu} \\ $$$$\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\left[\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}{u}}{{u}+\mathrm{1}}{du}−\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}{v}}{{v}+\mathrm{1}}{dv}−\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{v}^{−\frac{\mathrm{1}}{\mathrm{2}}} \mathrm{ln}{v}}{{v}+\mathrm{1}}{dv}\right] \\ $$$$\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left(\mathrm{1}−{u}\right)\mathrm{ln}{u}}{\mathrm{1}−{u}^{\mathrm{2}} }{du}−\frac{\mathrm{1}}{\mathrm{8}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left(\mathrm{1}−{v}\right)\mathrm{ln}{v}}{\mathrm{1}−{v}^{\mathrm{2}} }{dv}−\frac{\mathrm{1}}{\mathrm{8}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{v}^{−\frac{\mathrm{1}}{\mathrm{2}}} \left(\mathrm{1}−{v}\right)\mathrm{ln}{v}}{\mathrm{1}−{v}^{\mathrm{2}} }{dv} \\ $$$$\:\:\:\:=\frac{\mathrm{1}}{\mathrm{8}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left({t}^{−\frac{\mathrm{1}}{\mathrm{2}}} −\mathrm{1}\right)\mathrm{ln}{t}}{\mathrm{1}−{t}}{dt}−\frac{\mathrm{1}}{\mathrm{32}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left({t}^{−\frac{\mathrm{1}}{\mathrm{2}}} −\mathrm{1}\right)\mathrm{ln}{t}}{\mathrm{1}−{t}}{dt}−\frac{\mathrm{1}}{\mathrm{32}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left({t}^{−\frac{\mathrm{1}}{\mathrm{4}}} −{t}^{\frac{\mathrm{1}}{\mathrm{4}}} \right)\mathrm{ln}{t}}{\mathrm{1}−{t}}{dt} \\ $$$$\:\:\:\:=\frac{\mathrm{1}}{\mathrm{8}}\left(\psi'\left(\mathrm{1}\right)−\psi'\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)−\frac{\mathrm{1}}{\mathrm{32}}\left(\psi'\left(\mathrm{1}\right)−\psi'\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)−\frac{\mathrm{1}}{\mathrm{32}}\left(\psi'\left(\frac{\mathrm{5}}{\mathrm{4}}\right)−\psi'\left(\frac{\mathrm{3}}{\mathrm{4}}\right)\right) \\ $$$$\:\:\:\:=\frac{\mathrm{1}}{\mathrm{8}}\left(\zeta\left(\mathrm{2}\right)−\mathrm{3}\zeta\left(\mathrm{2}\right)\right)−\frac{\mathrm{1}}{\mathrm{32}}\left(\zeta\left(\mathrm{2}\right)−\mathrm{3}\zeta\left(\mathrm{2}\right)\right)−\frac{\mathrm{1}}{\mathrm{32}}\left(\psi'\left(\frac{\mathrm{1}}{\mathrm{4}}\right)−\psi'\left(\frac{\mathrm{3}}{\mathrm{4}}\right)−\mathrm{16}\right) \\ $$$$\:\:\:\:=−\frac{\mathrm{3}}{\mathrm{16}}\zeta\left(\mathrm{2}\right)−\frac{\mathrm{1}}{\mathrm{32}}\left(\mathrm{2}\psi'\left(\frac{\mathrm{1}}{\mathrm{4}}\right)−\pi^{\mathrm{2}} \mathrm{cosec}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{4}}\right)\right)+\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Commented by SANOGO last updated on 12/Sep/21
$${merci}\:{bien}\:{le}\:{cerveau} \\ $$
