Question-154089

Question Number 154089 by iloveisrael last updated on 14/Sep/21

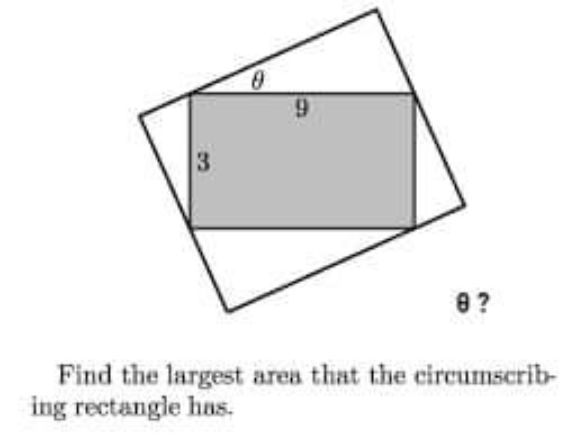
Answered by talminator2856791 last updated on 14/Sep/21

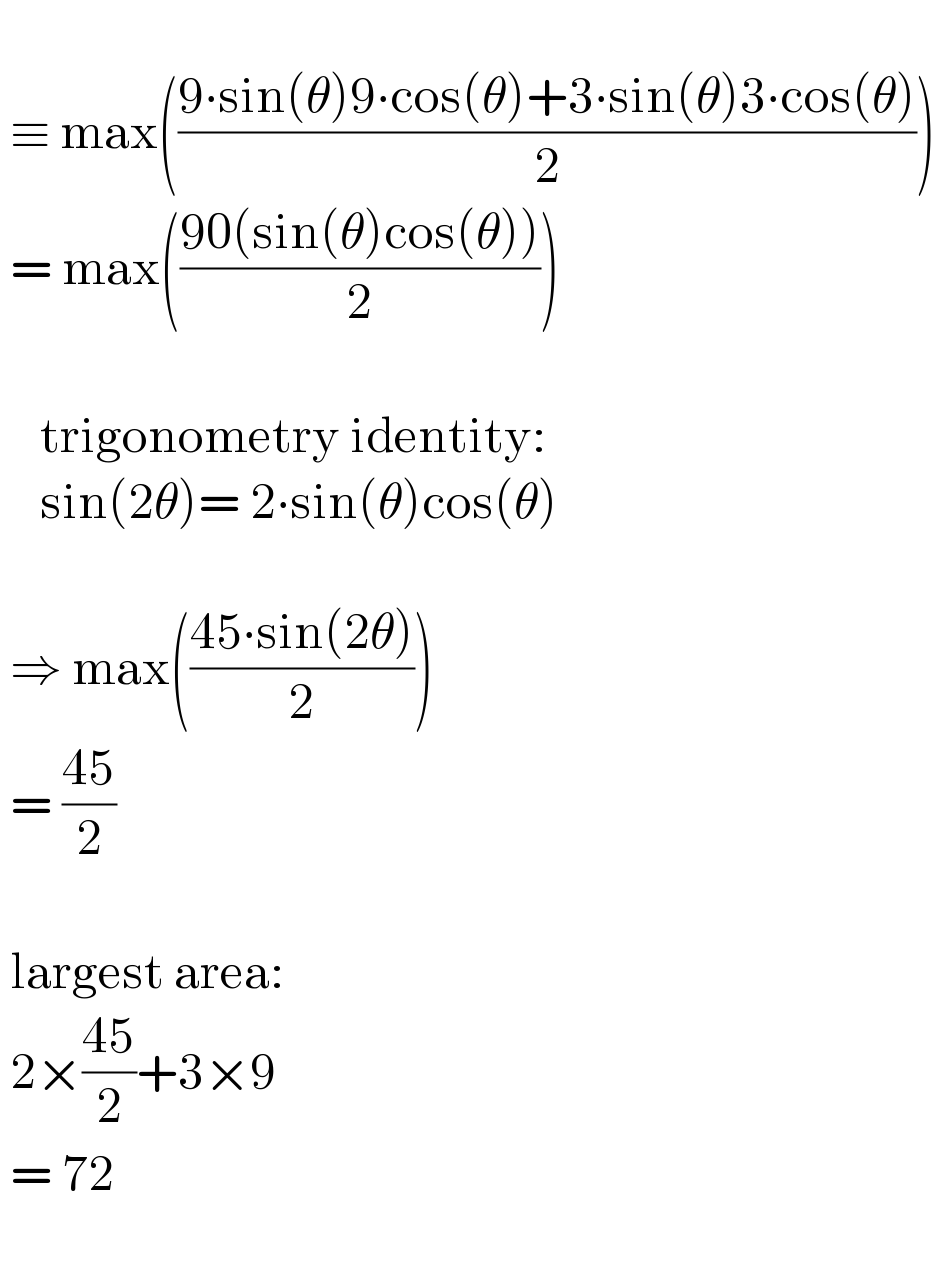
≡ max(((9∙sin(θ)9∙cos(θ)+3∙sin(θ)3∙cos(θ))/2)) = max(((90(sin(θ)cos(θ)))/2)) trigonometry identity: sin(2θ)= 2∙sin(θ)cos(θ) ⇒ max(((45∙sin(2θ))/2)) = ((45)/2) largest area: 2×((45)/2)+3×9 = 72

$$\: \\ $$$$\:\equiv\:\mathrm{max}\left(\frac{\mathrm{9}\centerdot\mathrm{sin}\left(\theta\right)\mathrm{9}\centerdot\mathrm{cos}\left(\theta\right)+\mathrm{3}\centerdot\mathrm{sin}\left(\theta\right)\mathrm{3}\centerdot\mathrm{cos}\left(\theta\right)}{\mathrm{2}}\right) \\ $$$$\:=\:\mathrm{max}\left(\frac{\mathrm{90}\left(\mathrm{sin}\left(\theta\right)\mathrm{cos}\left(\theta\right)\right)}{\mathrm{2}}\right) \\ $$$$\:\:\:\: \\ $$$$\:\:\:\:\mathrm{trigonometry}\:\mathrm{identity}: \\ $$$$\:\:\:\:\mathrm{sin}\left(\mathrm{2}\theta\right)=\:\mathrm{2}\centerdot\mathrm{sin}\left(\theta\right)\mathrm{cos}\left(\theta\right) \\ $$$$\: \\ $$$$\:\Rightarrow\:\mathrm{max}\left(\frac{\mathrm{45}\centerdot\mathrm{sin}\left(\mathrm{2}\theta\right)}{\mathrm{2}}\right) \\ $$$$\:=\:\frac{\mathrm{45}}{\mathrm{2}} \\ $$$$\: \\ $$$$\:\mathrm{largest}\:\mathrm{area}: \\ $$$$\:\mathrm{2}×\frac{\mathrm{45}}{\mathrm{2}}+\mathrm{3}×\mathrm{9} \\ $$$$\:=\:\mathrm{72} \\ $$$$\: \\ $$

Answered by mr W last updated on 14/Sep/21

due to symmetry: a_(max) =((3+9)/( (√2)))=6(√2) A_(max) =(6(√2))^2 =72 at θ=45°

$${due}\:{to}\:{symmetry}: \\ $$$${a}_{{max}} =\frac{\mathrm{3}+\mathrm{9}}{\:\sqrt{\mathrm{2}}}=\mathrm{6}\sqrt{\mathrm{2}} \\ $$$${A}_{{max}} =\left(\mathrm{6}\sqrt{\mathrm{2}}\right)^{\mathrm{2}} =\mathrm{72} \\ $$$${at}\:\theta=\mathrm{45}° \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply