Question-155537

Question Number 155537 by SANOGO last updated on 01/Oct/21

Answered by amin96 last updated on 01/Oct/21

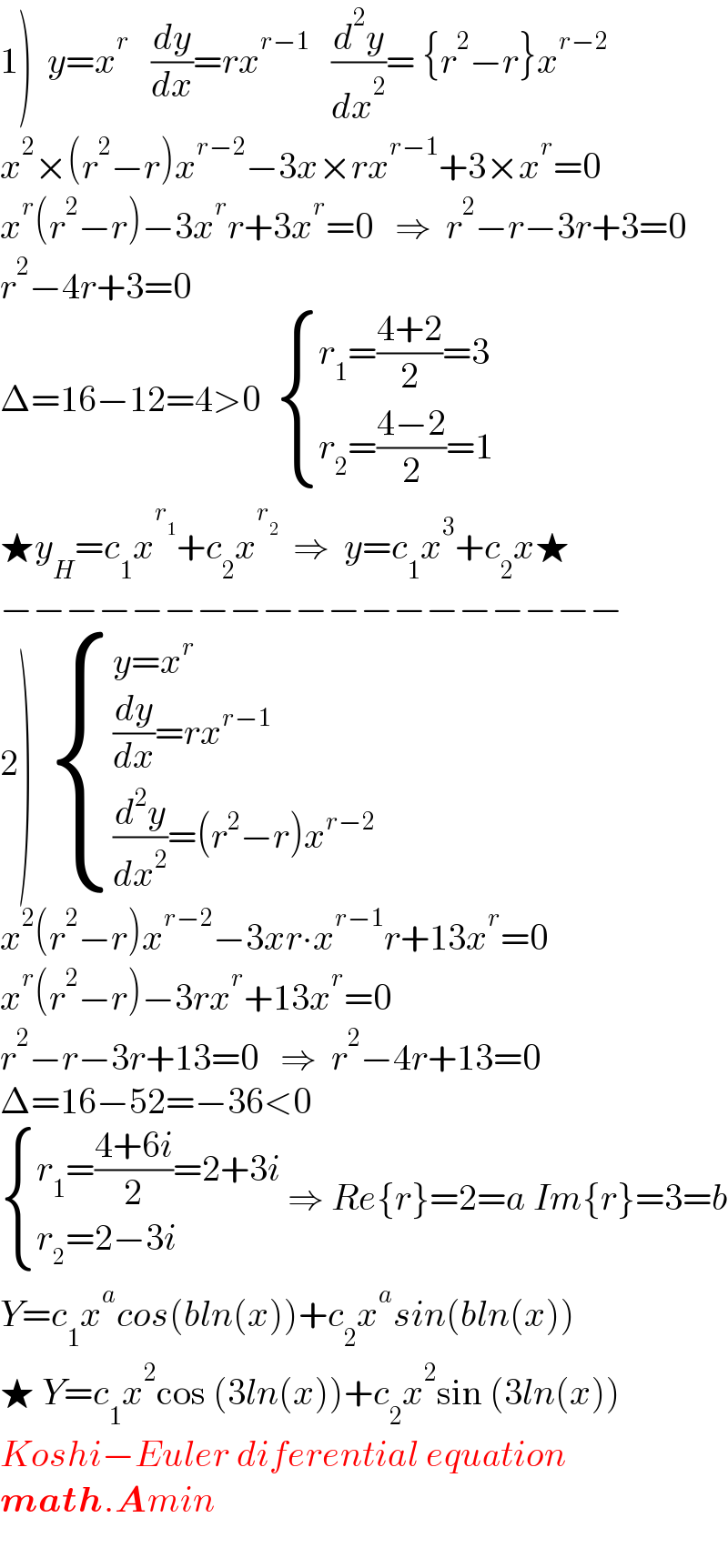
1) y=x^r (dy/dx)=rx^(r−1 ) (d^2 y/dx^2 )= {r^2 −r}x^(r−2) x^2 ×(r^2 −r)x^(r−2) −3x×rx^(r−1) +3×x^r =0 x^r (r^2 −r)−3x^r r+3x^r =0 ⇒ r^2 −r−3r+3=0 r^2 −4r+3=0 Δ=16−12=4>0 { ((r_1 =((4+2)/2)=3)),((r_2 =((4−2)/2)=1)) :} ★y_H =c_1 x^r_1 +c_2 x^r_2 ⇒ y=c_1 x^3 +c_2 x★ −−−−−−−−−−−−−−−−−−− 2) { ((y=x^r )),(((dy/dx)=rx^(r−1) )),(((d^2 y/dx^2 )=(r^2 −r)x^(r−2) )) :} x^2 (r^2 −r)x^(r−2) −3xr∙x^(r−1) r+13x^r =0 x^r (r^2 −r)−3rx^r +13x^r =0 r^2 −r−3r+13=0 ⇒ r^2 −4r+13=0 Δ=16−52=−36<0 { ((r_1 =((4+6i)/2)=2+3i)),((r_2 =2−3i)) :} ⇒ Re{r}=2=a Im{r}=3=b Y=c_1 x^a cos(bln(x))+c_2 x^a sin(bln(x)) ★ Y=c_1 x^2 cos (3ln(x))+c_2 x^2 sin (3ln(x)) Koshi−Euler diferential equation math.Amin

$$\left.\mathrm{1}\right)\:\:{y}={x}^{{r}} \:\:\:\frac{{dy}}{{dx}}={rx}^{{r}−\mathrm{1}\:\:\:\:} \:\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }=\:\left\{{r}^{\mathrm{2}} −{r}\right\}{x}^{{r}−\mathrm{2}} \\ $$$${x}^{\mathrm{2}} ×\left({r}^{\mathrm{2}} −{r}\right){x}^{{r}−\mathrm{2}} −\mathrm{3}{x}×{rx}^{{r}−\mathrm{1}} +\mathrm{3}×{x}^{{r}} =\mathrm{0} \\ $$$${x}^{{r}} \left({r}^{\mathrm{2}} −{r}\right)−\mathrm{3}{x}^{{r}} {r}+\mathrm{3}{x}^{{r}} =\mathrm{0}\:\:\:\Rightarrow\:\:{r}^{\mathrm{2}} −{r}−\mathrm{3}{r}+\mathrm{3}=\mathrm{0} \\ $$$${r}^{\mathrm{2}} −\mathrm{4}{r}+\mathrm{3}=\mathrm{0} \\ $$$$\Delta=\mathrm{16}−\mathrm{12}=\mathrm{4}>\mathrm{0}\:\:\begin{cases}{{r}_{\mathrm{1}} =\frac{\mathrm{4}+\mathrm{2}}{\mathrm{2}}=\mathrm{3}}\\{{r}_{\mathrm{2}} =\frac{\mathrm{4}−\mathrm{2}}{\mathrm{2}}=\mathrm{1}}\end{cases} \\ $$$$\bigstar{y}_{{H}} ={c}_{\mathrm{1}} {x}^{{r}_{\mathrm{1}} } +{c}_{\mathrm{2}} {x}^{{r}_{\mathrm{2}} } \:\:\Rightarrow\:\:{y}={c}_{\mathrm{1}} {x}^{\mathrm{3}} +{c}_{\mathrm{2}} {x}\bigstar \\ $$$$−−−−−−−−−−−−−−−−−−− \\ $$$$\left.\mathrm{2}\right)\:\:\begin{cases}{{y}={x}^{{r}} }\\{\frac{{dy}}{{dx}}={rx}^{{r}−\mathrm{1}} }\\{\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }=\left({r}^{\mathrm{2}} −{r}\right){x}^{{r}−\mathrm{2}} }\end{cases} \\ $$$${x}^{\mathrm{2}} \left({r}^{\mathrm{2}} −{r}\right){x}^{{r}−\mathrm{2}} −\mathrm{3}{xr}\centerdot{x}^{{r}−\mathrm{1}} {r}+\mathrm{13}{x}^{{r}} =\mathrm{0} \\ $$$${x}^{{r}} \left({r}^{\mathrm{2}} −{r}\right)−\mathrm{3}{rx}^{{r}} +\mathrm{13}{x}^{{r}} =\mathrm{0} \\ $$$${r}^{\mathrm{2}} −{r}−\mathrm{3}{r}+\mathrm{13}=\mathrm{0}\:\:\:\Rightarrow\:\:{r}^{\mathrm{2}} −\mathrm{4}{r}+\mathrm{13}=\mathrm{0} \\ $$$$\Delta=\mathrm{16}−\mathrm{52}=−\mathrm{36}<\mathrm{0} \\ $$$$\begin{cases}{{r}_{\mathrm{1}} =\frac{\mathrm{4}+\mathrm{6}{i}}{\mathrm{2}}=\mathrm{2}+\mathrm{3}{i}}\\{{r}_{\mathrm{2}} =\mathrm{2}−\mathrm{3}{i}}\end{cases}\:\Rightarrow\:{Re}\left\{{r}\right\}=\mathrm{2}={a}\:{Im}\left\{{r}\right\}=\mathrm{3}={b} \\ $$$${Y}={c}_{\mathrm{1}} {x}^{{a}} {cos}\left({bln}\left({x}\right)\right)+{c}_{\mathrm{2}} {x}^{{a}} {sin}\left({bln}\left({x}\right)\right) \\ $$$$\bigstar\:{Y}={c}_{\mathrm{1}} {x}^{\mathrm{2}} \mathrm{cos}\:\left(\mathrm{3}{ln}\left({x}\right)\right)+{c}_{\mathrm{2}} {x}^{\mathrm{2}} \mathrm{sin}\:\left(\mathrm{3}{ln}\left({x}\right)\right) \\ $$$${Koshi}−{Euler}\:{diferential}\:{equation} \\ $$$$\boldsymbol{{math}}.\boldsymbol{{A}}{min} \\ $$$$ \\ $$

Commented by SANOGO last updated on 02/Oct/21

$${merci}\:{bien}\:{le}\:\:{general} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply