Question-156676

Question Number 156676 by mathlove last updated on 14/Oct/21

Commented by MathSh last updated on 14/Oct/21

\frac{2}{a} + \frac{1}{b} + \frac{1}{c} = 3 \Rightarrow \frac{21}{3} = 7

Answered by som(math1967) last updated on 14/Oct/21

60 = 27^{a} \Rightarrow 60^{\frac{1}{a}} = 27

60^{\frac{1}{b}} = 64, 60^{\frac{1}{c}} = 125

60^{\frac{1}{a} + \frac{1}{b} + \frac{1}{c}} = 3^{3} \times 4^{3} \times 5^{3}

{(60)}^{\frac{a b + b c + c a}{a b c}} = {(60)}^{3}

\frac{a b + b c + c a}{a b c} = 3

\frac{a b c}{a b + b c + c a} = \frac{1}{3}

∴ \frac{21 a b c}{a b + b c + c a} = 21 \times \frac{1}{3} = 7 a n s

Answered by Rasheed.Sindhi last updated on 14/Oct/21

A n A lternate A pproach

{(3^{a})}^{3} = {(4^{b})}^{3} = {(5^{c})}^{3} = 60

{(3^{a})}^{3} {(4^{b})}^{3} {(5^{c})}^{3} = {(60)}^{3}

3^{a} 4^{b} 5^{c} = 60 = 3^{1} . 4^{1} . 5^{1}

a = b = c = 1

\frac{21 a b c}{a b + b c + c a} = \frac{21.1.1.1}{1.1 + 1.1 + 1.1} = \frac{21}{3} = 7

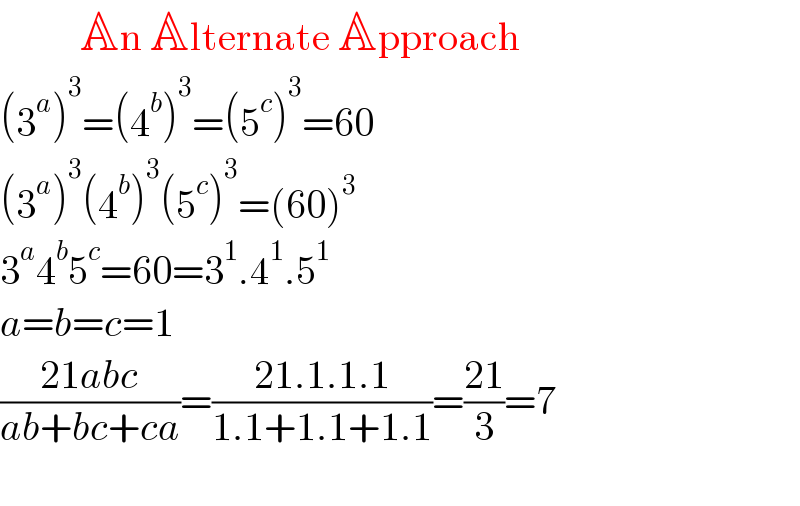
Commented by mr W last updated on 14/Oct/21

b u t a = b = c = 1 {d o e s n}^{'} t f u l f i l l t h e

g i v e n c o n d i t i o n .

Commented by Rasheed.Sindhi last updated on 14/Oct/21

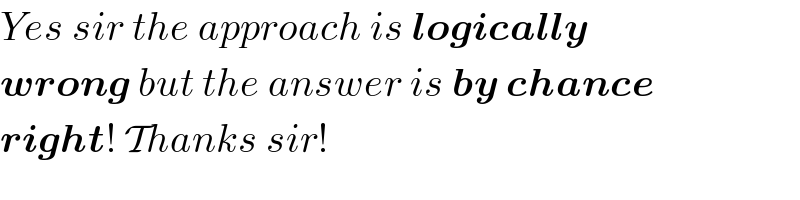
Y e s s i r t h e a p p r o a c h i s l o g i c a l l y

w r o n g b u t t h e a n s w e r i s b y c h a n c e

r i g h t! T h a n k s s i r!

Answered by john_santu last updated on 14/Oct/21

\frac{21}{\frac{1}{c} + \frac{1}{a} + \frac{1}{b}} = k

{\begin{cases} 27^{a} = 60 \Rightarrow a = \log_{27} (60) \\ 64^{b} = 60 \Rightarrow b = \log_{64} (60) \\ 125^{c} = 60 \Rightarrow c = \log_{125} (60) \end{cases}

t h e r e f o r e k = \frac{21}{\log_{60} (125) + \log_{60} (27) + \log_{60} (64)}

k = \frac{21}{\log_{60} (125 \times 27 \times 64)} = \frac{27}{\log_{60} (60^{3})}

k = \frac{21}{3 \log_{60} (60)} = \frac{21}{3} = 7 .

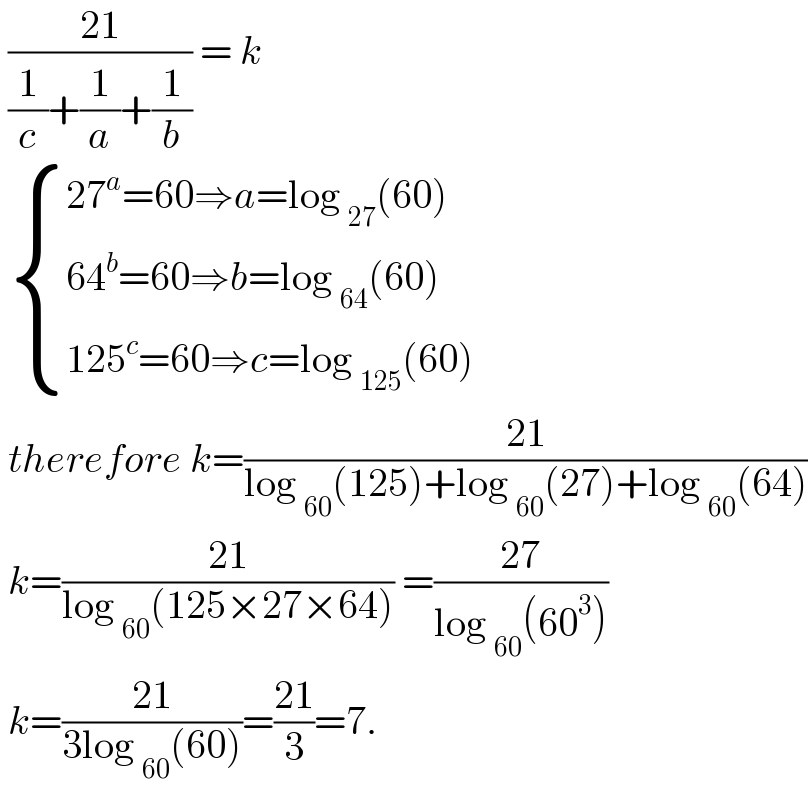
Commented by cortano last updated on 14/Oct/21

waw

Commented by peter frank last updated on 14/Oct/21

great