Question Number 156867 by cortano last updated on 16/Oct/21

Answered by qaz last updated on 16/Oct/21
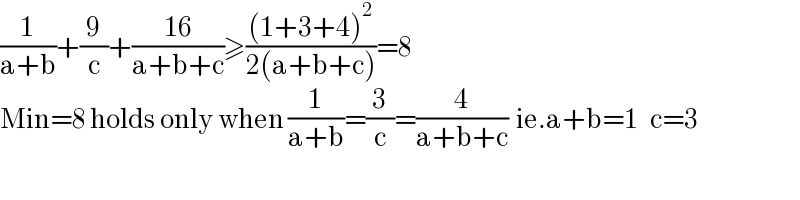
$$\frac{\mathrm{1}}{\mathrm{a}+\mathrm{b}}+\frac{\mathrm{9}}{\mathrm{c}}+\frac{\mathrm{16}}{\mathrm{a}+\mathrm{b}+\mathrm{c}}\geqslant\frac{\left(\mathrm{1}+\mathrm{3}+\mathrm{4}\right)^{\mathrm{2}} }{\mathrm{2}\left(\mathrm{a}+\mathrm{b}+\mathrm{c}\right)}=\mathrm{8} \\ $$$$\mathrm{Min}=\mathrm{8}\:\mathrm{holds}\:\mathrm{only}\:\mathrm{when}\:\frac{\mathrm{1}}{\mathrm{a}+\mathrm{b}}=\frac{\mathrm{3}}{\mathrm{c}}=\frac{\mathrm{4}}{\mathrm{a}+\mathrm{b}+\mathrm{c}}\:\:\mathrm{ie}.\mathrm{a}+\mathrm{b}=\mathrm{1}\:\:\:\mathrm{c}=\mathrm{3} \\ $$
Commented by cortano last updated on 16/Oct/21

$${Tittus}\:{lemma} \\ $$
