Menu Close

Question-156966

Tinku Tara 0 Comments
Pin




Question Number 156966 by Armindo last updated on 17/Oct/21
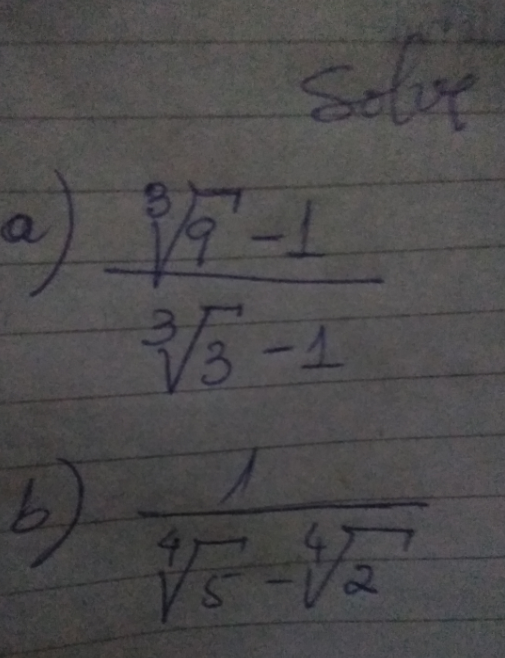
Commented by Armindo last updated on 17/Oct/21
Helô, I need help...
Answered by Rasheed.Sindhi last updated on 18/Oct/21
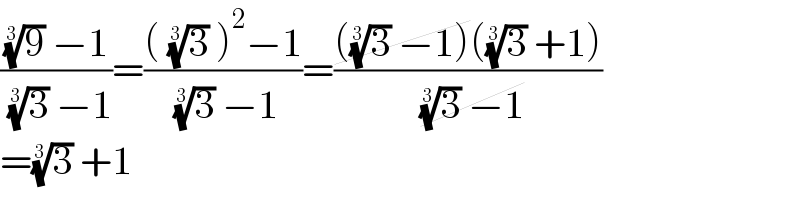
(((9)^(1/3) −1)/( (3)^(1/3) −1))=((( (3)^(1/3) )^2 −1)/( (3)^(1/3) −1))=((((3)^(1/3) −1)((3)^(1/3) +1))/( (3)^(1/3) −1)) =(3)^(1/3) +1
93−133−1=(33)2−133−1=(33−1)(33+1)33−1=33+1
Answered by depressiveshrek last updated on 18/Oct/21
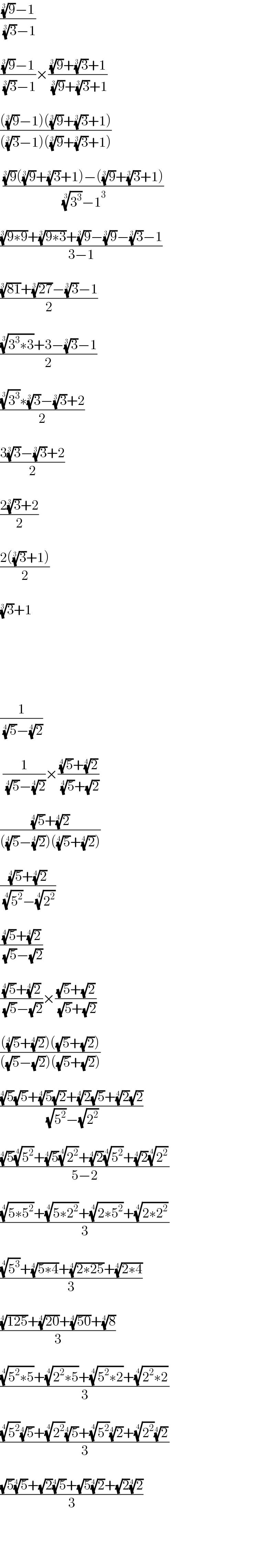
(((9)^(1/3) −1)/( (3)^(1/3) −1)) (((9)^(1/3) −1)/( (3)^(1/3) −1))×(((9)^(1/3) +(3)^(1/3) +1)/( (9)^(1/3) +(3)^(1/3) +1)) ((((9)^(1/3) −1)((9)^(1/3) +(3)^(1/3) +1))/(((3)^(1/3) −1)((9)^(1/3) +(3)^(1/3) +1))) (((9)^(1/3) ((9)^(1/3) +(3)^(1/3) +1)−((9)^(1/3) +(3)^(1/3) +1))/( (3^3 )^(1/3) −1^3 )) ((((9∗9))^(1/3) +((9∗3))^(1/3) +(9)^(1/3) −(9)^(1/3) −(3)^(1/3) −1)/(3−1)) ((((81))^(1/3) +((27))^(1/3) −(3)^(1/3) −1)/2) ((((3^3 ∗3))^(1/3) +3−(3)^(1/3) −1)/2) (((3^3 )^(1/3) ∗(3)^(1/3) −(3)^(1/3) +2)/2) ((3(3)^(1/3) −(3)^(1/3) +2)/2) ((2(3)^(1/3) +2)/2) ((2((3)^(1/3) +1))/2) (3)^(1/3) +1 (1/( (5)^(1/4) −(2)^(1/4) )) (1/( (5)^(1/4) −(2)^(1/4) ))×(((5)^(1/4) +(2)^(1/4) )/( (5)^(1/4) +(√2))) (((5)^(1/4) +(2)^(1/4) )/(((5)^(1/4) −(2)^(1/4) )((5)^(1/4) +(2)^(1/4) ))) (((5)^(1/4) +(2)^(1/4) )/( (5^2 )^(1/4) −(2^2 )^(1/4) )) (((5)^(1/4) +(2)^(1/4) )/( (√5)−(√2))) (((5)^(1/4) +(2)^(1/4) )/( (√5)−(√2)))×(((√5)+(√2))/( (√5)+(√2))) ((((5)^(1/4) +(2)^(1/4) )((√5)+(√2)))/(((√5)−(√2))((√5)+(√2)))) (((5)^(1/4) (√5)+(5)^(1/4) (√2)+(2)^(1/4) (√5)+(2)^(1/4) (√2))/( (√5^2 )−(√2^2 ))) (((5)^(1/4) (5^2 )^(1/4) +(5)^(1/4) (2^2 )^(1/4) +(2)^(1/4) (5^2 )^(1/4) +(2)^(1/4) (2^2 )^(1/4) )/(5−2)) ((((5∗5^2 ))^(1/4) +((5∗2^2 ))^(1/4) +((2∗5^2 ))^(1/4) +((2∗2^2 ))^(1/4) )/3) (((5^3 )^(1/4) +((5∗4))^(1/4) +((2∗25))^(1/4) +((2∗4))^(1/4) )/3) ((((125))^(1/4) +((20))^(1/4) +((50))^(1/4) +(8)^(1/4) )/3) ((((5^2 ∗5))^(1/4) +((2^2 ∗5))^(1/4) +((5^2 ∗2))^(1/4) +((2^2 ∗2))^(1/4) )/3) (((5^2 )^(1/4) (5)^(1/4) +(2^2 )^(1/4) (5)^(1/4) +(5^2 )^(1/4) (2)^(1/4) +(2^2 )^(1/4) (2)^(1/4) )/3) (((√5)(5)^(1/4) +(√2)(5)^(1/4) +(√5)(2)^(1/4) +(√2)(2)^(1/4) )/3)
93−133−193−133−1×93+33+193+33+1(93−1)(93+33+1)(33−1)(93+33+1)93(93+33+1)−(93+33+1)333−139∗93+9∗33+93−93−33−13−1813+273−33−1233∗33+3−33−12333∗33−33+22333−33+22233+222(33+1)233+1154−24154−24×54+2454+254+24(54−24)(54+24)54+24524−22454+245−254+245−2×5+25+2(54+24)(5+2)(5−2)(5+2)545+542+245+24252−2254524+54224+24524+242245−25∗524+5∗224+2∗524+2∗2243534+5∗44+2∗254+2∗4431254+204+504+84352∗54+22∗54+52∗24+22∗24352454+22454+52424+224243554+254+524+2243
Commented by Armindo last updated on 18/Oct/21
thanks...I will check the procedure! do you have a book, when I find that solve or This procedure?

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *