Question Number 157630 by Oberon last updated on 25/Oct/21

Commented by ehab last updated on 25/Oct/21

$$ \\ $$
Answered by mindispower last updated on 26/Oct/21
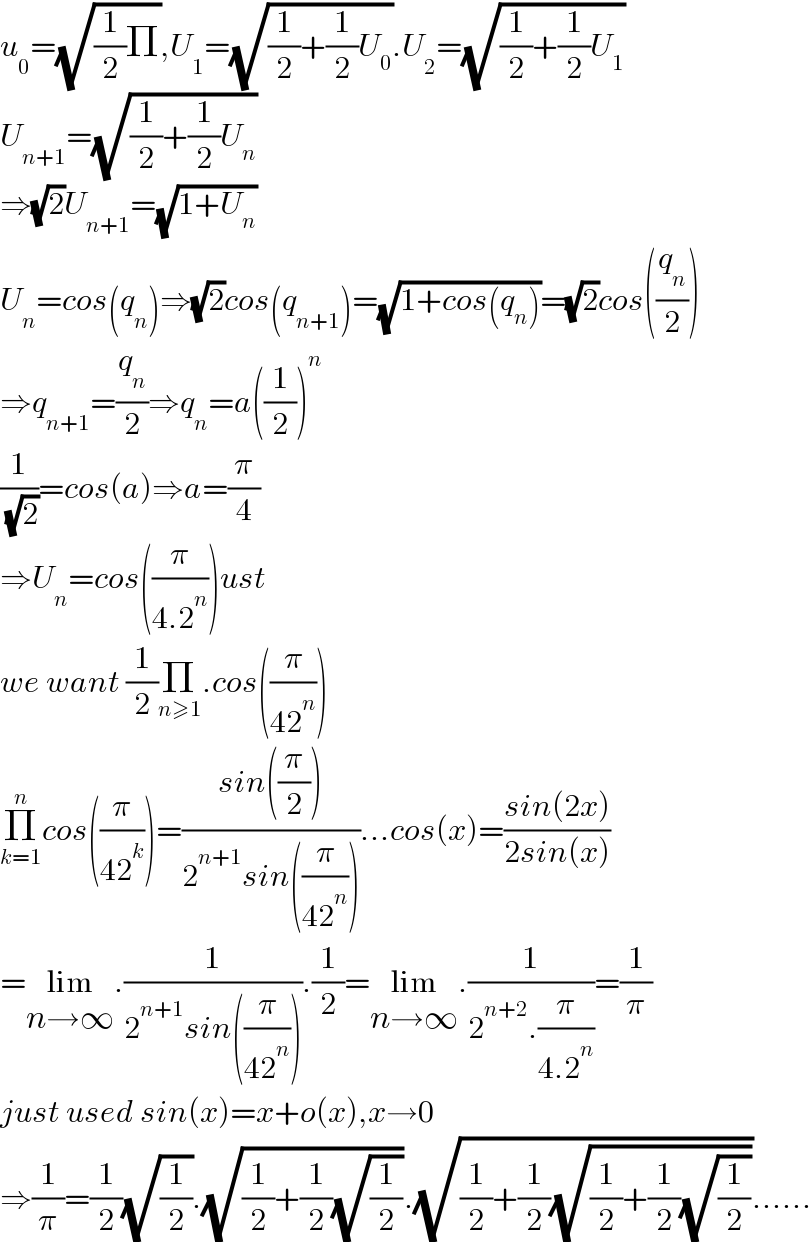
$${u}_{\mathrm{0}} =\sqrt{\frac{\mathrm{1}}{\mathrm{2}}\Pi},{U}_{\mathrm{1}} =\sqrt{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}{U}_{\mathrm{0}} }.{U}_{\mathrm{2}} =\sqrt{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}{U}_{\mathrm{1}} } \\ $$$${U}_{{n}+\mathrm{1}} =\sqrt{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}{U}_{{n}} } \\ $$$$\Rightarrow\sqrt{\mathrm{2}}{U}_{{n}+\mathrm{1}} =\sqrt{\mathrm{1}+{U}_{{n}} } \\ $$$${U}_{{n}} ={cos}\left({q}_{{n}} \right)\Rightarrow\sqrt{\mathrm{2}}{cos}\left({q}_{{n}+\mathrm{1}} \right)=\sqrt{\mathrm{1}+{cos}\left({q}_{{n}} \right)}=\sqrt{\mathrm{2}}{cos}\left(\frac{{q}_{{n}} }{\mathrm{2}}\right) \\ $$$$\Rightarrow{q}_{{n}+\mathrm{1}} =\frac{{q}_{{n}} }{\mathrm{2}}\Rightarrow{q}_{{n}} ={a}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} \\ $$$$\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}={cos}\left({a}\right)\Rightarrow{a}=\frac{\pi}{\mathrm{4}} \\ $$$$\Rightarrow{U}_{{n}} ={cos}\left(\frac{\pi}{\mathrm{4}.\mathrm{2}^{{n}} }\right){ust} \\ $$$${we}\:{want}\:\frac{\mathrm{1}}{\mathrm{2}}\underset{{n}\geqslant\mathrm{1}} {\prod}.{cos}\left(\frac{\pi}{\mathrm{42}^{{n}} }\right) \\ $$$$\underset{{k}=\mathrm{1}} {\overset{{n}} {\prod}}{cos}\left(\frac{\pi}{\mathrm{42}^{{k}} }\right)=\frac{{sin}\left(\frac{\pi}{\mathrm{2}}\right)}{\mathrm{2}^{{n}+\mathrm{1}} {sin}\left(\frac{\pi}{\mathrm{42}^{{n}} }\right)}…{cos}\left({x}\right)=\frac{{sin}\left(\mathrm{2}{x}\right)}{\mathrm{2}{sin}\left({x}\right)} \\ $$$$=\underset{{n}\rightarrow\infty} {\mathrm{lim}}.\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} {sin}\left(\frac{\pi}{\mathrm{42}^{{n}} }\right)}.\frac{\mathrm{1}}{\mathrm{2}}=\underset{{n}\rightarrow\infty} {\mathrm{lim}}.\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{2}} .\frac{\pi}{\mathrm{4}.\mathrm{2}^{{n}} }}=\frac{\mathrm{1}}{\pi} \\ $$$${just}\:{used}\:{sin}\left({x}\right)={x}+{o}\left({x}\right),{x}\rightarrow\mathrm{0} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\pi}=\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\mathrm{1}}{\mathrm{2}}}.\sqrt{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\mathrm{1}}{\mathrm{2}}}}.\sqrt{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\mathrm{1}}{\mathrm{2}}}}}…… \\ $$
