Question-159396

Question Number 159396 by quvonnn last updated on 16/Nov/21

Answered by som(math1967) last updated on 16/Nov/21

a r e a o f △ B C E = A r e a o f △ E C F

= \frac{1}{2} \times 4 \times 3 = 6 s q . u n i t

C M i s b i s e c t o r o f ∠ C

∴ \frac{E M}{M B} = \frac{E C}{C B} = \frac{3}{4}

\frac{A r △ E M C}{A r △ C M B} = \frac{3}{4}

∴ A r . △ E M C = \frac{3}{7} \times 6 = \frac{18}{7} s q u n i t

S = A r . △ E M F = 6 - \frac{18}{7} = \frac{24}{7} s q u n i t a n s

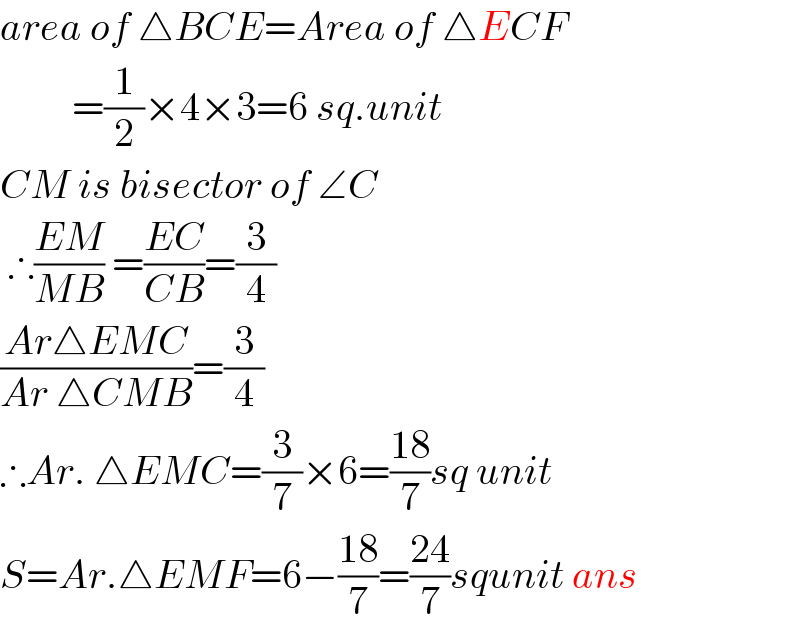
Commented by som(math1967) last updated on 16/Nov/21

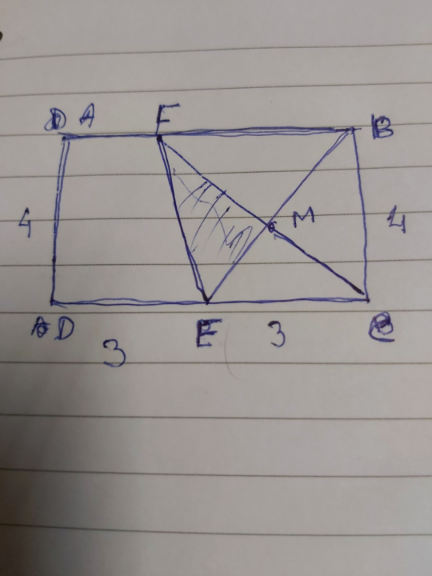
Commented by Rasheed.Sindhi last updated on 16/Nov/21

a r e a o f △ B C E \overset{?}{=} A r e a o f △ B C F

A r e a o f △ B C F = 4 \times 4 \times \frac{1}{2} = 8 {u n i t}^{2}

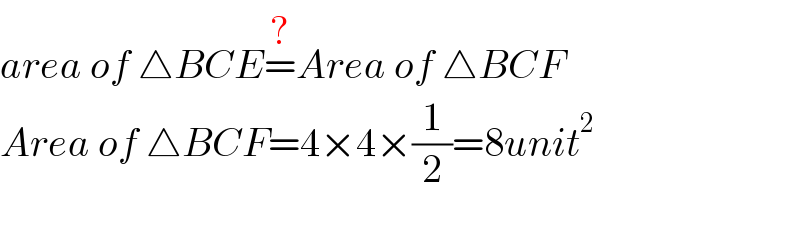
Commented by som(math1967) last updated on 16/Nov/21

t y p o s i r i t △ E C F

Commented by Avijit007 last updated on 16/Nov/21

y e s .