Question-159611

Question Number 159611 by cortano last updated on 19/Nov/21

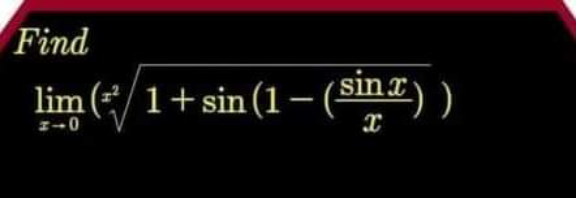
Answered by qaz last updated on 19/Nov/21

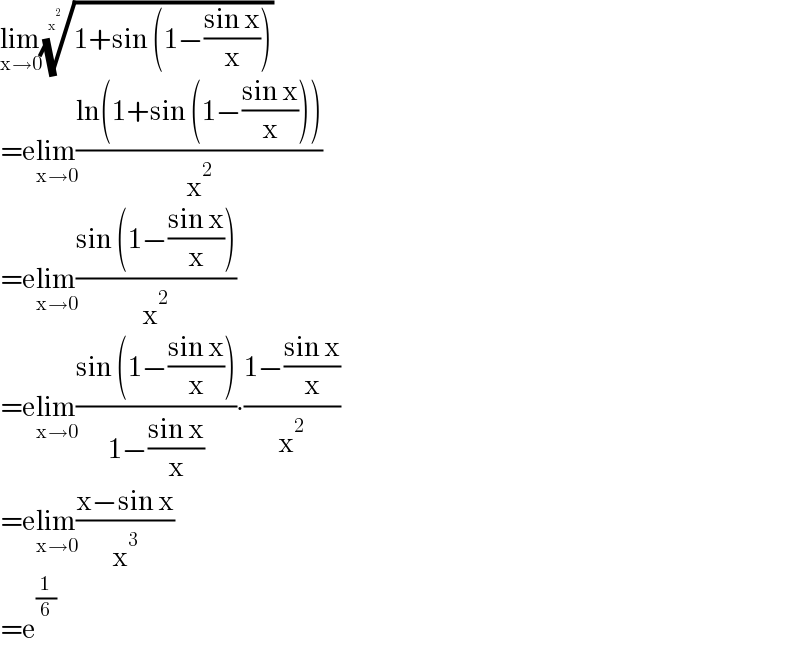
lim_(x→0) ((1+sin (1−((sin x)/x))))^(1/x^2 ) =elim_(x→0) ((ln(1+sin (1−((sin x)/x))))/x^2 ) =elim_(x→0) ((sin (1−((sin x)/x)))/x^2 ) =elim_(x→0) ((sin (1−((sin x)/x)))/(1−((sin x)/x)))∙((1−((sin x)/x))/x^2 ) =elim_(x→0) ((x−sin x)/x^3 ) =e^(1/6)

$$\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\sqrt[{\mathrm{x}^{\mathrm{2}} }]{\mathrm{1}+\mathrm{sin}\:\left(\mathrm{1}−\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}\right)} \\ $$$$=\mathrm{e}\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{sin}\:\left(\mathrm{1}−\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}\right)\right)}{\mathrm{x}^{\mathrm{2}} } \\ $$$$=\mathrm{e}\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sin}\:\left(\mathrm{1}−\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}\right)}{\mathrm{x}^{\mathrm{2}} } \\ $$$$=\mathrm{e}\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sin}\:\left(\mathrm{1}−\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}\right)}{\mathrm{1}−\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}}\centerdot\frac{\mathrm{1}−\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}}{\mathrm{x}^{\mathrm{2}} } \\ $$$$=\mathrm{e}\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{x}−\mathrm{sin}\:\mathrm{x}}{\mathrm{x}^{\mathrm{3}} } \\ $$$$=\mathrm{e}^{\frac{\mathrm{1}}{\mathrm{6}}} \\ $$

Answered by tounghoungko last updated on 19/Nov/21

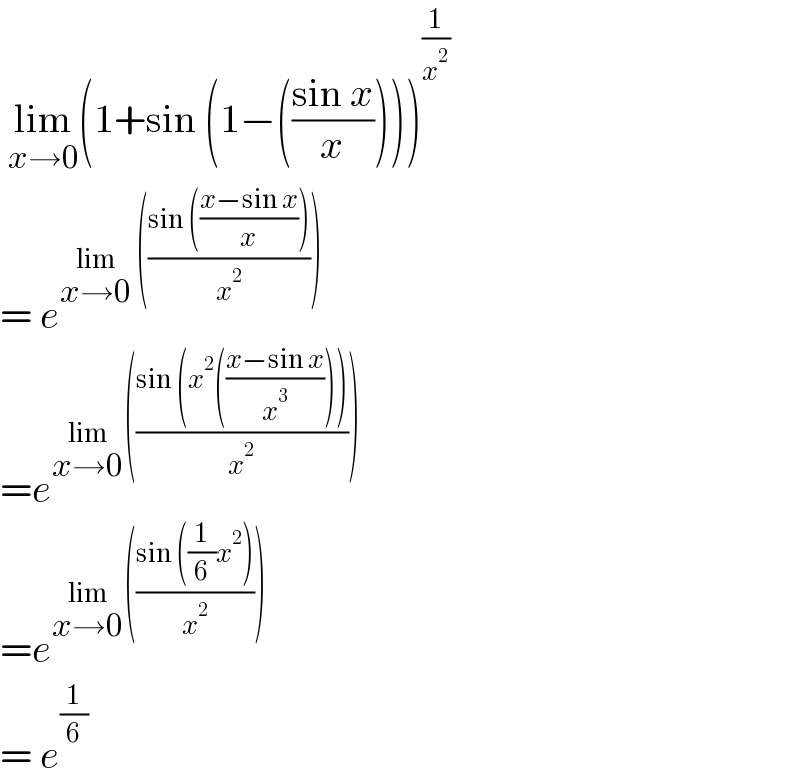
lim_(x→0) (1+sin (1−(((sin x)/x))))^(1/x^2 ) = e^(lim_(x→0) (((sin (((x−sin x)/x)))/x^2 ))) =e^(lim_(x→0) (((sin (x^2 (((x−sin x)/x^3 ))))/x^2 ))) =e^(lim_(x→0) (((sin ((1/6)x^2 ))/x^2 ))) = e^(1/6)

$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\mathrm{1}+\mathrm{sin}\:\left(\mathrm{1}−\left(\frac{\mathrm{sin}\:{x}}{{x}}\right)\right)\right)^{\frac{\mathrm{1}}{{x}^{\mathrm{2}} }} \\ $$$$=\:{e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left(\frac{\mathrm{sin}\:\left(\frac{{x}−\mathrm{sin}\:{x}}{{x}}\right)}{{x}^{\mathrm{2}} }\right)} \\ $$$$={e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{\mathrm{sin}\:\left({x}^{\mathrm{2}} \left(\frac{{x}−\mathrm{sin}\:{x}}{{x}^{\mathrm{3}} }\right)\right)}{{x}^{\mathrm{2}} }\right)} \\ $$$$={e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{\mathrm{sin}\:\left(\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{2}} \right)}{{x}^{\mathrm{2}} }\right)} \\ $$$$=\:{e}^{\frac{\mathrm{1}}{\mathrm{6}}} \: \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply