Menu Close

Question-160530

Tinku Tara 0 Comments
Pin




Question Number 160530 by alcohol last updated on 01/Dec/21
Answered by Rasheed.Sindhi last updated on 01/Dec/21
I(1) v=(s/t); s: distance,v: average speed, t:time a. 10km/h t=(s/v)=((40km)/(10km/h))=4 hours First 20km with 20km/h t=((20km)/(20km/h))=1 hour Remaining time 3 hours to cover 20km v=((20km)/(3h))=6(1/3)km/h b. 30km/h t=(s/v)=((40km)/(30km/h))=1(1/3)hours First 20km with 20km/h t=((20km)/(20km/h))=1 hour Remaining time 1/3 hours to cover 20km v=((20km)/(1/3 h))=60km/h
$$\mathrm{I}\left(\mathrm{1}\right) \\ $$$$\mathrm{v}=\frac{\mathrm{s}}{\mathrm{t}};\:\mathrm{s}:\:\mathrm{distance},\mathrm{v}:\:\mathrm{average}\:\mathrm{speed},\:\mathrm{t}:\mathrm{time} \\ $$$$\underline{{a}.\:\:\mathrm{10km}/\mathrm{h}} \\ $$$$\mathrm{t}=\frac{\mathrm{s}}{\mathrm{v}}=\frac{\mathrm{40km}}{\mathrm{10km}/\mathrm{h}}=\mathrm{4}\:\mathrm{hours} \\ $$$$\mathrm{First}\:\mathrm{20km}\:\mathrm{with}\:\mathrm{20km}/\mathrm{h} \\ $$$$\mathrm{t}=\frac{\mathrm{20km}}{\mathrm{20km}/\mathrm{h}}=\mathrm{1}\:\mathrm{hour} \\ $$$$\mathrm{Remaining}\:\mathrm{time}\:\mathrm{3}\:\mathrm{hours}\:\mathrm{to}\:\mathrm{cover}\:\mathrm{20km} \\ $$$$\mathrm{v}=\frac{\mathrm{20km}}{\mathrm{3h}}=\mathrm{6}\frac{\mathrm{1}}{\mathrm{3}}\mathrm{km}/\mathrm{h} \\ $$$$\underline{{b}.\:\:\mathrm{30km}/\mathrm{h}} \\ $$$$\mathrm{t}=\frac{\mathrm{s}}{\mathrm{v}}=\frac{\mathrm{40km}}{\mathrm{30km}/\mathrm{h}}=\mathrm{1}\frac{\mathrm{1}}{\mathrm{3}}\mathrm{hours} \\ $$$$\mathrm{First}\:\mathrm{20km}\:\mathrm{with}\:\mathrm{20km}/\mathrm{h} \\ $$$$\mathrm{t}=\frac{\mathrm{20km}}{\mathrm{20km}/\mathrm{h}}=\mathrm{1}\:\mathrm{hour} \\ $$$$\mathrm{Remaining}\:\mathrm{time}\:\mathrm{1}/\mathrm{3}\:\mathrm{hours}\:\mathrm{to}\:\mathrm{cover}\:\mathrm{20km} \\ $$$$\mathrm{v}=\frac{\mathrm{20km}}{\mathrm{1}/\mathrm{3}\:\mathrm{h}}=\mathrm{60km}/\mathrm{h} \\ $$$$ \\ $$
Answered by FongXD last updated on 01/Dec/21
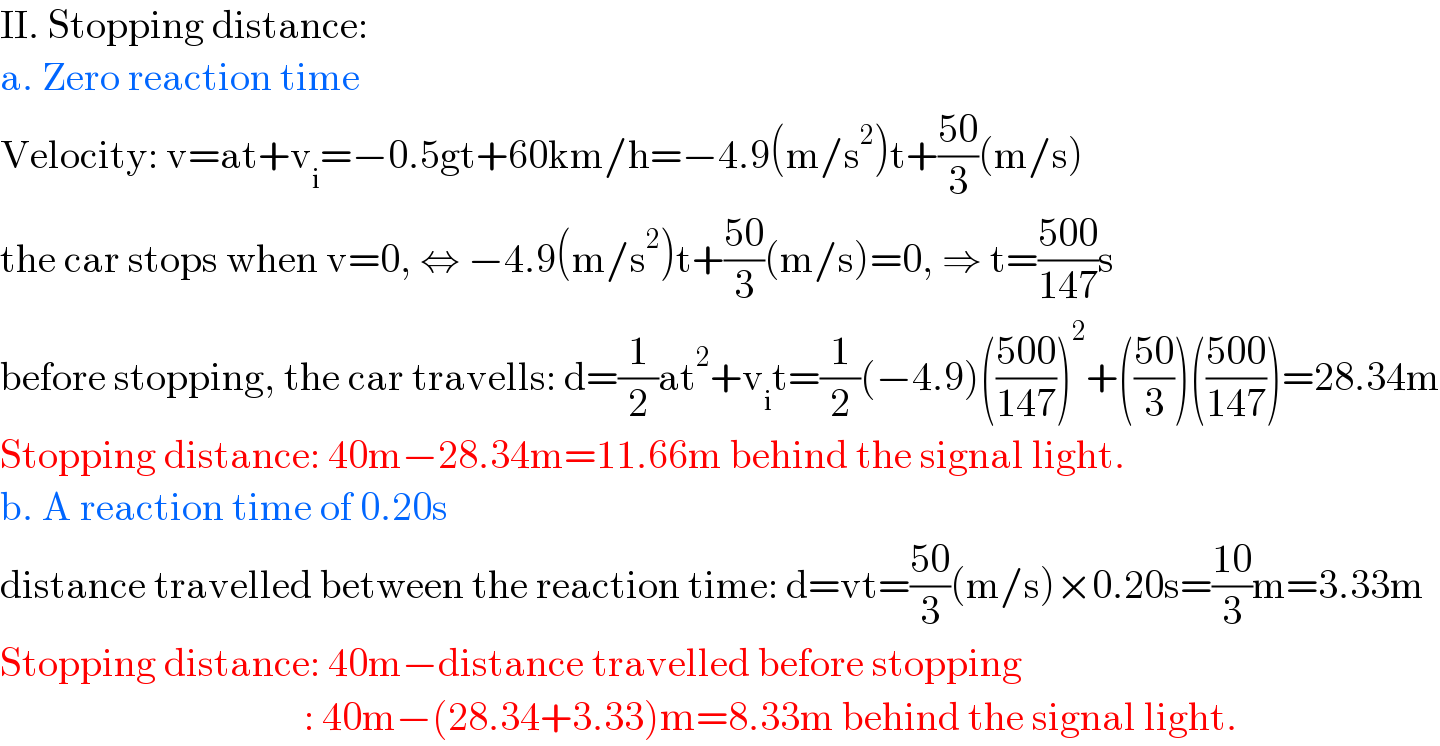
II. Stopping distance: a. Zero reaction time Velocity: v=at+v_i =−0.5gt+60km/h=−4.9(m/s^2 )t+((50)/3)(m/s) the car stops when v=0, ⇔ −4.9(m/s^2 )t+((50)/3)(m/s)=0, ⇒ t=((500)/(147))s before stopping, the car travells: d=(1/2)at^2 +v_i t=(1/2)(−4.9)(((500)/(147)))^2 +(((50)/3))(((500)/(147)))=28.34m Stopping distance: 40m−28.34m=11.66m behind the signal light. b. A reaction time of 0.20s distance travelled between the reaction time: d=vt=((50)/3)(m/s)×0.20s=((10)/3)m=3.33m Stopping distance: 40m−distance travelled before stopping : 40m−(28.34+3.33)m=8.33m behind the signal light.
$$\mathrm{II}.\:\mathrm{Stopping}\:\mathrm{distance}: \\ $$$$\mathrm{a}.\:\mathrm{Zero}\:\mathrm{reaction}\:\mathrm{time} \\ $$$$\mathrm{Velocity}:\:\mathrm{v}=\mathrm{at}+\mathrm{v}_{\mathrm{i}} =−\mathrm{0}.\mathrm{5gt}+\mathrm{60km}/\mathrm{h}=−\mathrm{4}.\mathrm{9}\left(\mathrm{m}/\mathrm{s}^{\mathrm{2}} \right)\mathrm{t}+\frac{\mathrm{50}}{\mathrm{3}}\left(\mathrm{m}/\mathrm{s}\right) \\ $$$$\mathrm{the}\:\mathrm{car}\:\mathrm{stops}\:\mathrm{when}\:\mathrm{v}=\mathrm{0},\:\Leftrightarrow\:−\mathrm{4}.\mathrm{9}\left(\mathrm{m}/\mathrm{s}^{\mathrm{2}} \right)\mathrm{t}+\frac{\mathrm{50}}{\mathrm{3}}\left(\mathrm{m}/\mathrm{s}\right)=\mathrm{0},\:\Rightarrow\:\mathrm{t}=\frac{\mathrm{500}}{\mathrm{147}}\mathrm{s} \\ $$$$\mathrm{before}\:\mathrm{stopping},\:\mathrm{the}\:\mathrm{car}\:\mathrm{travells}:\:\mathrm{d}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{at}^{\mathrm{2}} +\mathrm{v}_{\mathrm{i}} \mathrm{t}=\frac{\mathrm{1}}{\mathrm{2}}\left(−\mathrm{4}.\mathrm{9}\right)\left(\frac{\mathrm{500}}{\mathrm{147}}\right)^{\mathrm{2}} +\left(\frac{\mathrm{50}}{\mathrm{3}}\right)\left(\frac{\mathrm{500}}{\mathrm{147}}\right)=\mathrm{28}.\mathrm{34m} \\ $$$$\mathrm{Stopping}\:\mathrm{distance}:\:\mathrm{40m}−\mathrm{28}.\mathrm{34m}=\mathrm{11}.\mathrm{66m}\:\mathrm{behind}\:\mathrm{the}\:\mathrm{signal}\:\mathrm{light}. \\ $$$$\mathrm{b}.\:\mathrm{A}\:\mathrm{reaction}\:\mathrm{time}\:\mathrm{of}\:\mathrm{0}.\mathrm{20s} \\ $$$$\mathrm{distance}\:\mathrm{travelled}\:\mathrm{between}\:\mathrm{the}\:\mathrm{reaction}\:\mathrm{time}:\:\mathrm{d}=\mathrm{vt}=\frac{\mathrm{50}}{\mathrm{3}}\left(\mathrm{m}/\mathrm{s}\right)×\mathrm{0}.\mathrm{20s}=\frac{\mathrm{10}}{\mathrm{3}}\mathrm{m}=\mathrm{3}.\mathrm{33m} \\ $$$$\mathrm{Stopping}\:\mathrm{distance}:\:\mathrm{40m}−\mathrm{distance}\:\mathrm{travelled}\:\mathrm{before}\:\mathrm{stopping} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\::\:\mathrm{40m}−\left(\mathrm{28}.\mathrm{34}+\mathrm{3}.\mathrm{33}\right)\mathrm{m}=\mathrm{8}.\mathrm{33m}\:\mathrm{behind}\:\mathrm{the}\:\mathrm{signal}\:\mathrm{light}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *