Question-160719

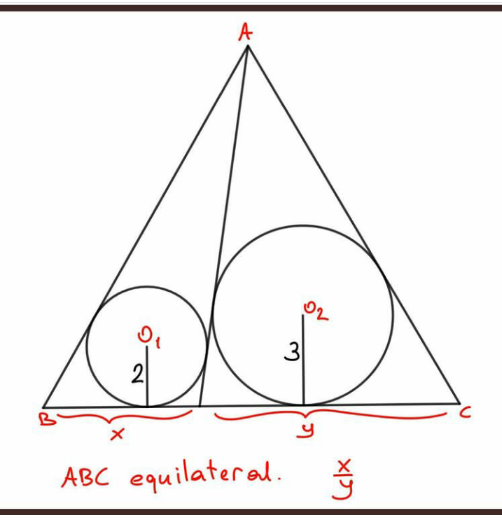
Question Number 160719 by mnjuly1970 last updated on 05/Dec/21

Commented by kowalsky78 last updated on 05/Dec/21

I g o t \frac{3}{5} . M a y b e I m a d e s o m e m i s t a k e .

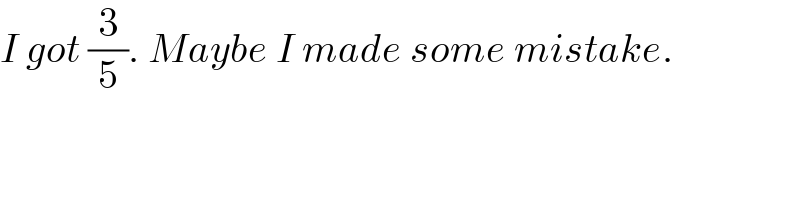
Commented by mr W last updated on 05/Dec/21

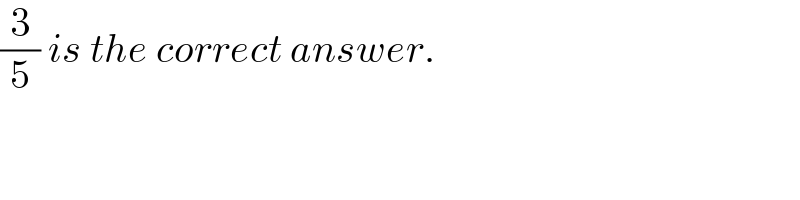
\frac{3}{5} i s t h e c o r r e c t a n s w e r .

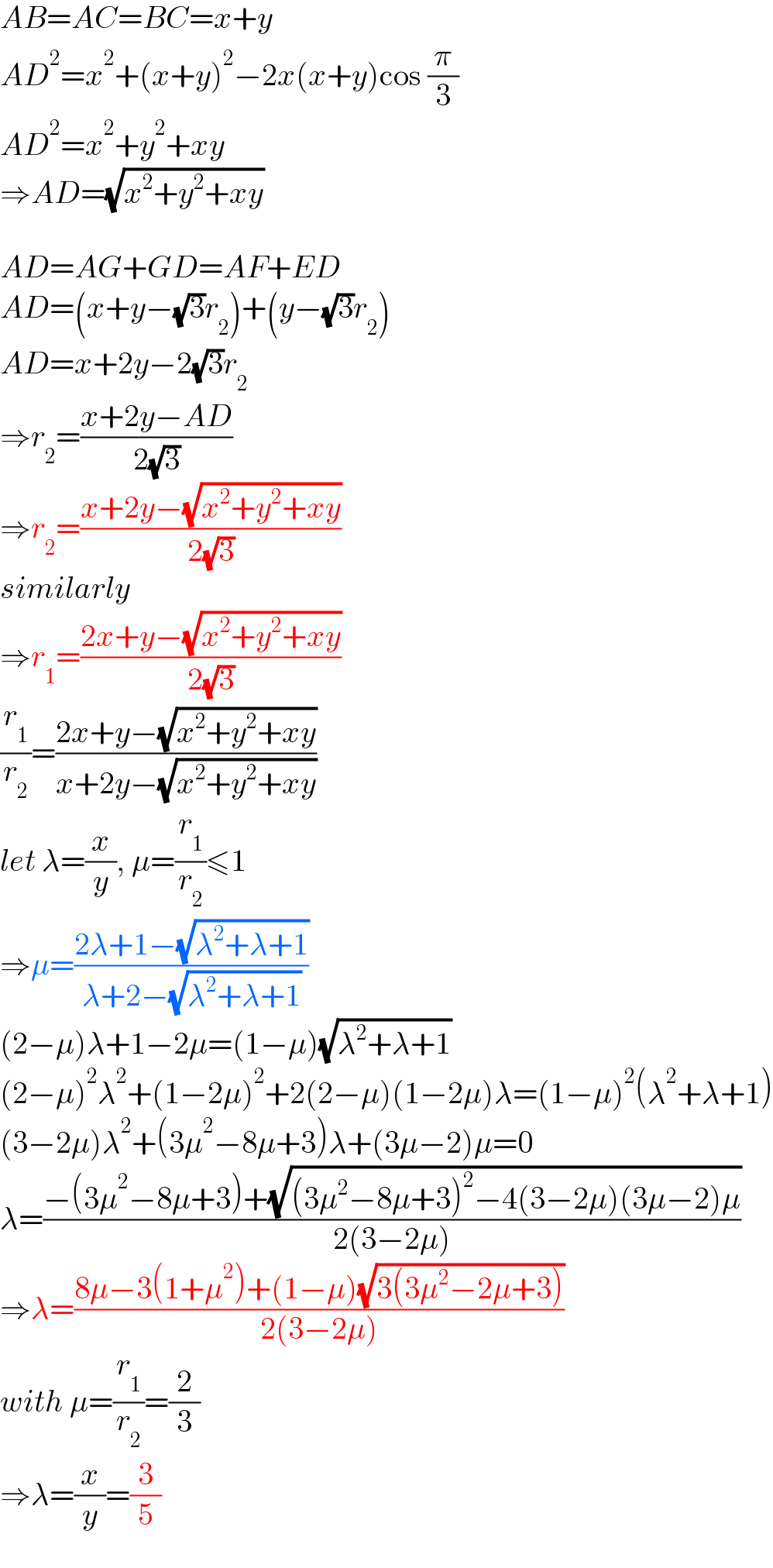
Answered by mr W last updated on 05/Dec/21

Commented by mr W last updated on 05/Dec/21

A B = A C = B C = x + y

{A D}^{2} = x^{2} + {(x + y)}^{2} - 2 x (x + y) \cos \frac{π}{3}

{A D}^{2} = x^{2} + y^{2} + x y

\Rightarrow A D = \sqrt{x^{2} + y^{2} + x y}

A D = A G + G D = A F + E D

A D = (x + y - \sqrt{3} r_{2}) + (y - \sqrt{3} r_{2})

A D = x + 2 y - 2 \sqrt{3} r_{2}

\Rightarrow r_{2} = \frac{x + 2 y - A D}{2 \sqrt{3}}

\Rightarrow r_{2} = \frac{x + 2 y - \sqrt{x^{2} + y^{2} + x y}}{2 \sqrt{3}}

s i m i l a r l y

\Rightarrow r_{1} = \frac{2 x + y - \sqrt{x^{2} + y^{2} + x y}}{2 \sqrt{3}}

\frac{r_{1}}{r_{2}} = \frac{2 x + y - \sqrt{x^{2} + y^{2} + x y}}{x + 2 y - \sqrt{x^{2} + y^{2} + x y}}

l e t λ = \frac{x}{y}, μ = \frac{r_{1}}{r_{2}} ⩽ 1

\Rightarrow μ = \frac{2 λ + 1 - \sqrt{λ^{2} + λ + 1}}{λ + 2 - \sqrt{λ^{2} + λ + 1}}

(2 - μ) λ + 1 - 2 μ = (1 - μ) \sqrt{λ^{2} + λ + 1}

{(2 - μ)}^{2} λ^{2} + {(1 - 2 μ)}^{2} + 2 (2 - μ) (1 - 2 μ) λ = {(1 - μ)}^{2} (λ^{2} + λ + 1)

(3 - 2 μ) λ^{2} + (3 μ^{2} - 8 μ + 3) λ + (3 μ - 2) μ = 0

λ = \frac{- (3 μ^{2} - 8 μ + 3) + \sqrt{{(3 μ^{2} - 8 μ + 3)}^{2} - 4 (3 - 2 μ) (3 μ - 2) μ}}{2 (3 - 2 μ)}

\Rightarrow λ = \frac{8 μ - 3 (1 + μ^{2}) + (1 - μ) \sqrt{3 (3 μ^{2} - 2 μ + 3)}}{2 (3 - 2 μ)}

w i t h μ = \frac{r_{1}}{r_{2}} = \frac{2}{3}

\Rightarrow λ = \frac{x}{y} = \frac{3}{5}

Commented by mnjuly1970 last updated on 05/Dec/21

g r a t e f u l s i r W

Commented by mr W last updated on 05/Dec/21

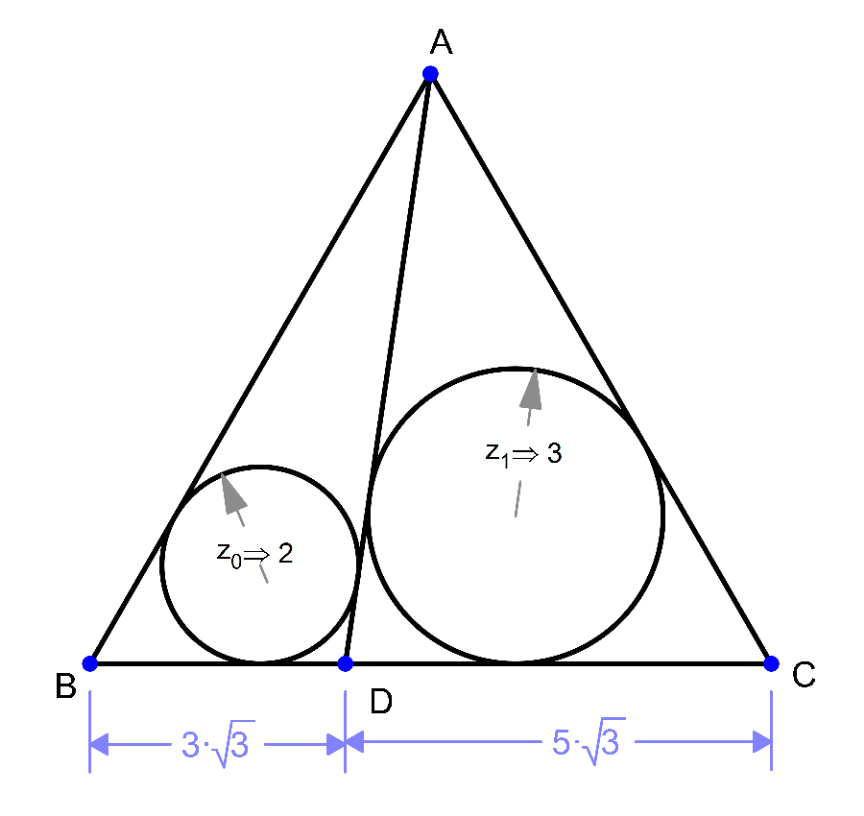
Commented by Tawa11 last updated on 05/Dec/21

Great sir .

Facebook Tweet Pin