Question Number 160844 by Tawa11 last updated on 07/Dec/21

Answered by mr W last updated on 08/Dec/21

Commented by mr W last updated on 08/Dec/21
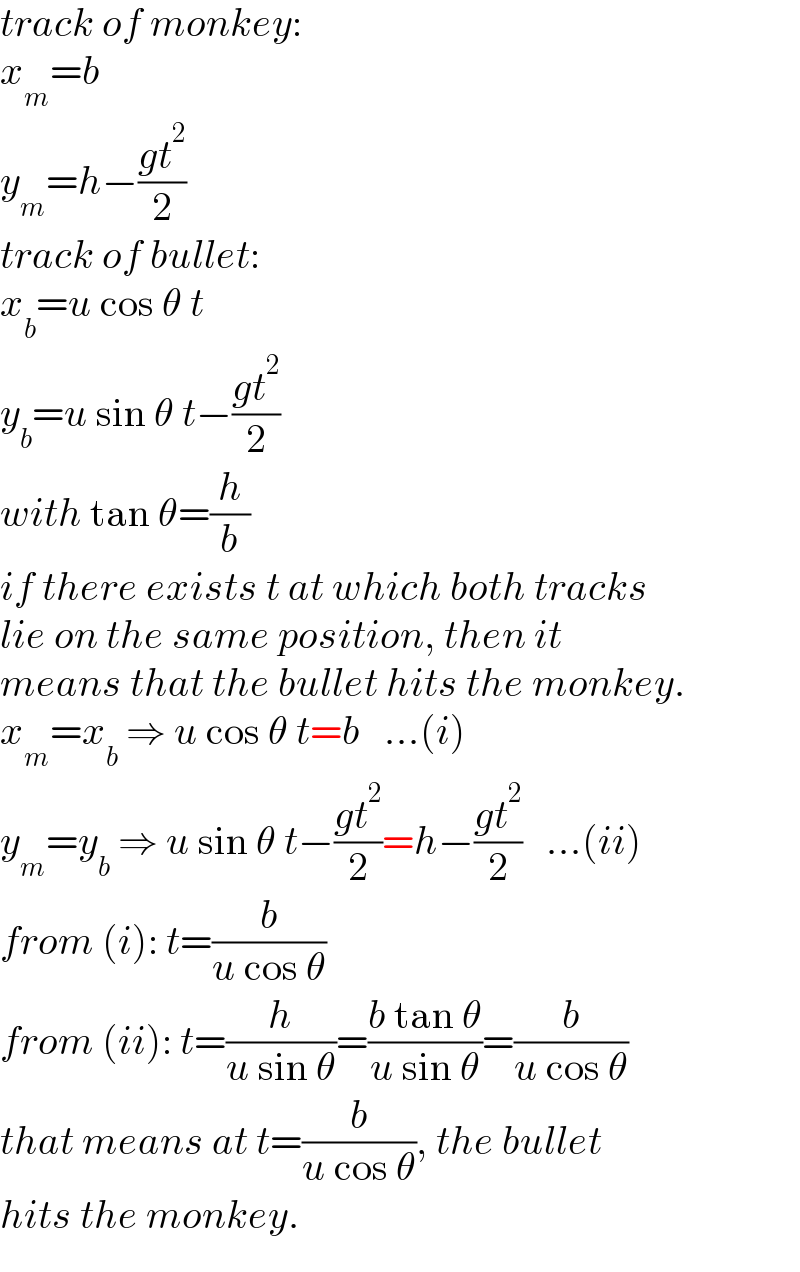
$${track}\:{of}\:{monkey}: \\ $$$${x}_{{m}} ={b} \\ $$$${y}_{{m}} ={h}−\frac{{gt}^{\mathrm{2}} }{\mathrm{2}} \\ $$$${track}\:{of}\:{bullet}: \\ $$$${x}_{{b}} ={u}\:\mathrm{cos}\:\theta\:{t} \\ $$$${y}_{{b}} ={u}\:\mathrm{sin}\:\theta\:{t}−\frac{{gt}^{\mathrm{2}} }{\mathrm{2}} \\ $$$${with}\:\mathrm{tan}\:\theta=\frac{{h}}{{b}} \\ $$$${if}\:{there}\:{exists}\:{t}\:{at}\:{which}\:{both}\:{tracks} \\ $$$${lie}\:{on}\:{the}\:{same}\:{position},\:{then}\:{it}\: \\ $$$${means}\:{that}\:{the}\:{bullet}\:{hits}\:{the}\:{monkey}. \\ $$$${x}_{{m}} ={x}_{{b}} \:\Rightarrow\:{u}\:\mathrm{cos}\:\theta\:{t}={b}\:\:\:…\left({i}\right) \\ $$$${y}_{{m}} ={y}_{{b}} \:\Rightarrow\:{u}\:\mathrm{sin}\:\theta\:{t}−\frac{{gt}^{\mathrm{2}} }{\mathrm{2}}={h}−\frac{{gt}^{\mathrm{2}} }{\mathrm{2}}\:\:\:…\left({ii}\right) \\ $$$${from}\:\left({i}\right):\:{t}=\frac{{b}}{{u}\:\mathrm{cos}\:\theta} \\ $$$${from}\:\left({ii}\right):\:{t}=\frac{{h}}{{u}\:\mathrm{sin}\:\theta}=\frac{{b}\:\mathrm{tan}\:\theta}{{u}\:\mathrm{sin}\:\theta}=\frac{{b}}{{u}\:\mathrm{cos}\:\theta} \\ $$$${that}\:{means}\:{at}\:{t}=\frac{{b}}{{u}\:\mathrm{cos}\:\theta},\:{the}\:{bullet} \\ $$$${hits}\:{the}\:{monkey}. \\ $$
Commented by Tawa11 last updated on 13/Dec/21
$$\mathrm{Wow},\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
