Question-162071

Question Number 162071 by mr W last updated on 26/Dec/21

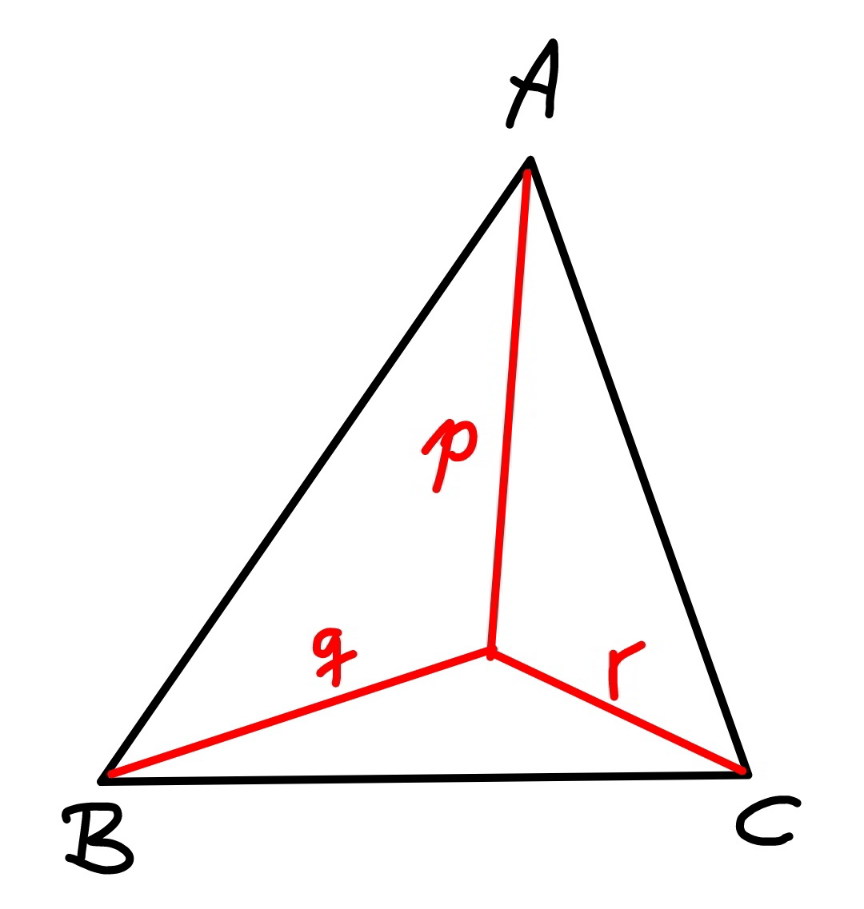
Commented by mr W last updated on 27/Dec/21

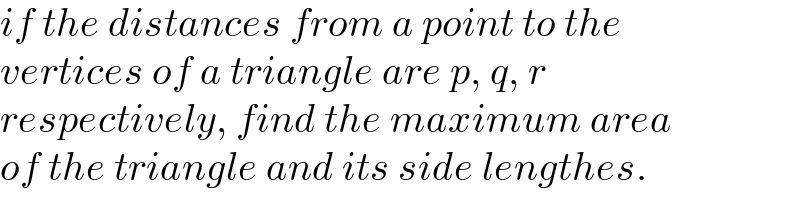
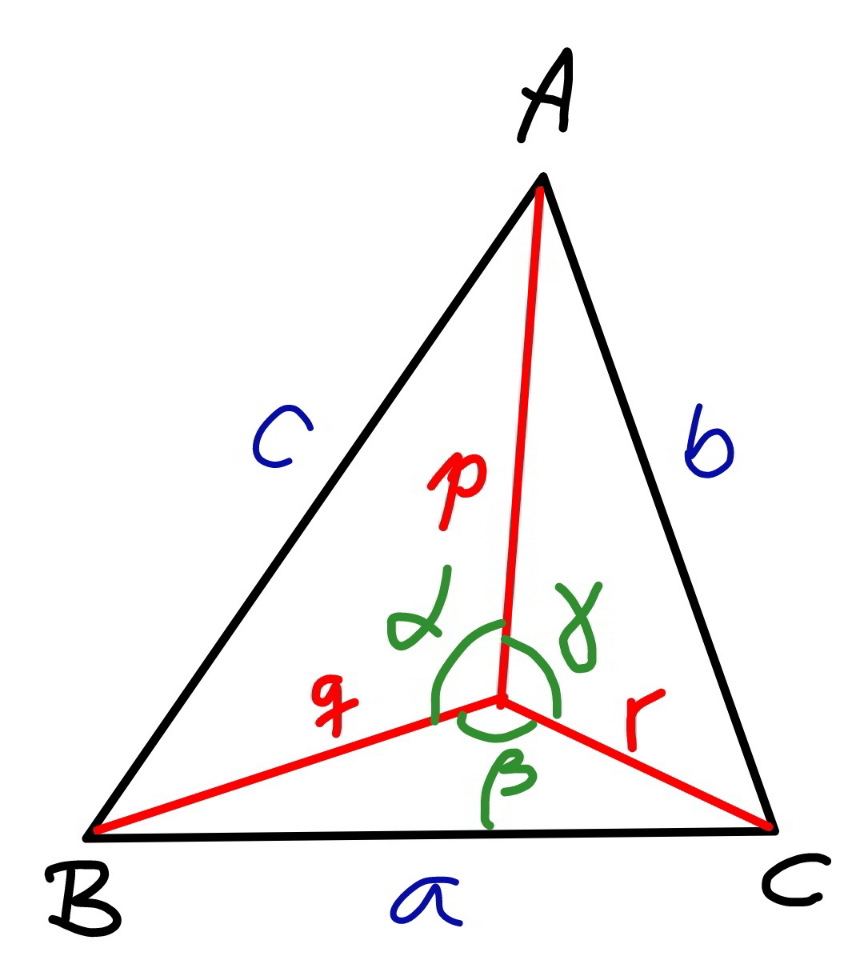
$${if}\:{the}\:{distances}\:{from}\:{a}\:{point}\:{to}\:{the} \\ $$$${vertices}\:{of}\:{a}\:{triangle}\:{are}\:{p},\:{q},\:{r} \\ $$$${respectively},\:{find}\:{the}\:{maximum}\:{area} \\ $$$${of}\:{the}\:{triangle}\:{and}\:{its}\:{side}\:{lengthes}. \\ $$

Commented by mr W last updated on 26/Dec/21

Commented by mr W last updated on 27/Dec/21

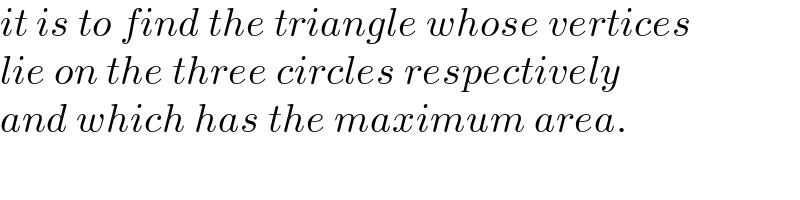
$${it}\:{is}\:{to}\:{find}\:{the}\:{triangle}\:{whose}\:{vertices} \\ $$$${lie}\:{on}\:{the}\:{three}\:{circles}\:{respectively} \\ $$$${and}\:{which}\:{has}\:{the}\:{maximum}\:{area}. \\ $$

Commented by Tawa11 last updated on 27/Dec/21

$$\mathrm{Wow},\:\mathrm{great}\:\mathrm{sir}. \\ $$

Commented by mr W last updated on 29/Dec/21

$${thanks}\:{for}\:{this}\:{vector}\:{way}\:{sir}! \\ $$$${i}\:{came}\:{to}\:{the}\:{same}\:{conclusion}\:{as} \\ $$$${stated}\:{below}. \\ $$

Commented by aleks041103 last updated on 29/Dec/21

$${Let}\:{p},{q},{r}\:{represent}\:{the}\:{vectors}. \\ $$$$\overset{\rightarrow} {{S}}={S}\overset{\rightarrow} {{e}_{{z}} }=\frac{\mathrm{1}}{\mathrm{2}}\left(\overset{\rightarrow} {{p}}×\overset{\rightarrow} {{q}}+\overset{\rightarrow} {{q}}×\overset{\rightarrow} {{r}}+\overset{\rightarrow} {{r}}×\overset{\rightarrow} {{p}}\right) \\ $$$${Since}\:{S}\:{is}\:{extremal},\:{then}\:{we}\:{may} \\ $$$${variate}\:{the}\:{vectors}\:{as} \\ $$$$\overset{\rightarrow} {{p}}\rightarrow\overset{\rightarrow} {{p}}+\delta\overset{\rightarrow} {{p}} \\ $$$$\overset{\rightarrow} {{q}}\rightarrow\overset{\rightarrow} {{q}}+\delta\overset{\rightarrow} {{q}} \\ $$$$\overset{\rightarrow} {{r}}\rightarrow\overset{\rightarrow} {{r}}+\delta\overset{\rightarrow} {{r}} \\ $$$${And}\:{search}\:{for}\:\overset{\rightarrow} {{p}},\overset{\rightarrow} {{q}},\overset{\rightarrow} {{r}}\:{which}\:{make}\:\delta{S}=\mathrm{0}\:{and} \\ $$$${so}\:\delta\overset{\rightarrow} {{S}}=\mathrm{0}. \\ $$$${Also}\:{the}\:{variations}\:{must}\:{be}\:{such}\:{that} \\ $$$${they}\:{preserve}\:\mid{p}\mid,\mid{q}\mid\:{and}\:\mid{r}\mid. \\ $$$$\delta\left(\overset{\rightarrow} {{p}}.\overset{\rightarrow} {{p}}\right)=\mathrm{2}\overset{\rightarrow} {{p}}.\delta\overset{\rightarrow} {{p}}=\mathrm{0}\Rightarrow\overset{\rightarrow} {{p}}\bot\delta\overset{\rightarrow} {{p}}. \\ $$$${Without}\:{restriction}\:{we}\:{may}\:{choose} \\ $$$$\delta\overset{\rightarrow} {{q}}=\delta\overset{\rightarrow} {{r}}=\mathrm{0}\:{since}\:{the}\:{variations}\:{are}\:{arbitrary} \\ $$$$\Rightarrow\delta{S}\overset{\rightarrow} {{e}_{{z}} }=\frac{\mathrm{1}}{\mathrm{2}}\left(\delta\overset{\rightarrow} {{p}}×\overset{\rightarrow} {{q}}+\overset{\rightarrow} {{r}}×\delta\overset{\rightarrow} {{p}}\right)=\mathrm{0} \\ $$$$\Rightarrow\delta\overset{\rightarrow} {{p}}×\left(\overset{\rightarrow} {{q}}−\overset{\rightarrow} {{r}}\right)=\mathrm{0} \\ $$$$\Rightarrow\overset{\rightarrow} {{p}}\bot\delta\overset{\rightarrow} {{p}}\parallel\overset{\rightarrow} {{q}}−\overset{\rightarrow} {{r}}\Rightarrow\overset{\rightarrow} {{p}}\bot\left(\overset{\rightarrow} {{q}}−\overset{\rightarrow} {{r}}\right) \\ $$$${But}\:\left(\overset{\rightarrow} {{q}}−\overset{\rightarrow} {{r}}\right)\:{is}\:{the}\:{side}\:{of}\:{the}\:{triangle} \\ $$$${opposite}\:{the}\:{vertex}\:{defined}\:{by}\:\overset{\rightarrow} {{p}}. \\ $$$$\Rightarrow\overset{\rightarrow} {{p}}\:{must}\:{be}\:{colinear}\:{to}\:{the}\:{height} \\ $$$${This}\:{must}\:{be}\:{true}\:{for}\:\overset{\rightarrow} {{q}}\:{and}\:\overset{\rightarrow} {{r}}\:{too}. \\ $$$$\Rightarrow\:{The}\:{starting}\:{point}\:{is}\:{the}\:{orthocenter}. \\ $$

Commented by papa last updated on 04/May/22

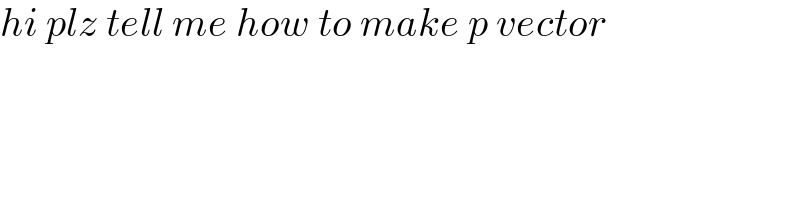
$${hi}\:{plz}\:{tell}\:{me}\:{how}\:{to}\:{make}\:{p}\:{vector} \\ $$

Commented by mr W last updated on 04/May/22

Commented by mr W last updated on 04/May/22

Answered by mr W last updated on 27/Dec/21

Commented by mr W last updated on 27/Dec/21

$${thanks}\:{for}\:{reviewing}\:{sir}!\:{you}\:{seem} \\ $$$${to}\:{have}\:{been}\:{absent}\:{for}\:{a}\:{long}\:{time}, \\ $$$${welcome}\:{back}! \\ $$

Commented by mr W last updated on 27/Dec/21

$${the}\:{area}\:{of}\:{ABC}\:{is} \\ $$$$\Delta=\frac{\mathrm{1}}{\mathrm{2}}\left({pq}\:\mathrm{sin}\:\alpha+{qr}\:\mathrm{sin}\:\beta+{rp}\:\mathrm{sin}\:\gamma\right) \\ $$$$\alpha+\beta+\gamma=\mathrm{2}\pi \\ $$$$\Delta=\frac{\mathrm{1}}{\mathrm{2}}\left[{pq}\:\mathrm{sin}\:\alpha+{qr}\:\mathrm{sin}\:\beta−{rp}\:\mathrm{sin}\:\left(\alpha+\beta\right)\right] \\ $$$${such}\:{that}\:\Delta\:{is}\:{maximum}, \\ $$$$\frac{\partial\Delta}{\partial\alpha}=\frac{\mathrm{1}}{\mathrm{2}}\left[{pq}\:\mathrm{cos}\:\alpha−{rp}\:\mathrm{cos}\:\left(\alpha+\beta\right)\right]=\mathrm{0} \\ $$$$\Rightarrow{pq}\:\mathrm{cos}\:\alpha={rp}\:\mathrm{cos}\:\left(\alpha+\beta\right)={rp}\:\mathrm{cos}\:\gamma \\ $$$$\frac{\partial\Delta}{\partial\beta}=\frac{\mathrm{1}}{\mathrm{2}}\left[{qr}\:\mathrm{cos}\:\beta−{rp}\:\mathrm{cos}\:\left(\alpha+\beta\right)\right]=\mathrm{0} \\ $$$$\Rightarrow{qr}\:\mathrm{cos}\:\beta={rp}\:\mathrm{cos}\:\left(\alpha+\beta\right)={rp}\:\mathrm{cos}\:\gamma \\ $$$$ \\ $$$${so}\:{we}\:{have} \\ $$$${pq}\:\mathrm{cos}\:\alpha={qr}\:\mathrm{cos}\:\beta={rp}\:\mathrm{cos}\:\gamma={k}\:{say} \\ $$$$\mathrm{cos}\:\alpha=\frac{{k}}{{pq}}\:\Rightarrow\mathrm{sin}\:\alpha=\sqrt{\mathrm{1}−\frac{{k}^{\mathrm{2}} }{{p}^{\mathrm{2}} {q}^{\mathrm{2}} }} \\ $$$$\mathrm{cos}\:\beta=\frac{{k}}{{qr}}\:\Rightarrow\mathrm{sin}\:\beta=\sqrt{\mathrm{1}−\frac{{k}^{\mathrm{2}} }{{q}^{\mathrm{2}} {r}^{\mathrm{2}} }} \\ $$$$\mathrm{cos}\:\gamma=\frac{{k}}{{rp}}\:\Rightarrow\mathrm{sin}\:\gamma=\sqrt{\mathrm{1}−\frac{{k}^{\mathrm{2}} }{{r}^{\mathrm{2}} {p}^{\mathrm{2}} }} \\ $$$${k}−{rp}\:\left[\frac{{k}^{\mathrm{2}} }{{pq}^{\mathrm{2}} {r}}−\sqrt{\left(\mathrm{1}−\frac{{k}^{\mathrm{2}} }{{p}^{\mathrm{2}} {q}^{\mathrm{2}} }\right)\left(\mathrm{1}−\frac{{k}^{\mathrm{2}} }{{q}^{\mathrm{2}} {r}^{\mathrm{2}} }\right)}\right]=\mathrm{0} \\ $$$$\sqrt{\left({p}^{\mathrm{2}} {q}^{\mathrm{2}} −{k}^{\mathrm{2}} \right)\left({q}^{\mathrm{2}} {r}^{\mathrm{2}} −{k}^{\mathrm{2}} \right)}={k}^{\mathrm{2}} −{q}^{\mathrm{2}} {k} \\ $$$${p}^{\mathrm{2}} {q}^{\mathrm{4}} {r}^{\mathrm{2}} −\left({p}^{\mathrm{2}} {q}^{\mathrm{2}} +{q}^{\mathrm{2}} {r}^{\mathrm{2}} \right){k}^{\mathrm{2}} +{k}^{\mathrm{4}} ={k}^{\mathrm{4}} +{q}^{\mathrm{4}} {k}^{\mathrm{2}} −\mathrm{2}{q}^{\mathrm{2}} {k}^{\mathrm{3}} \\ $$$${p}^{\mathrm{2}} {q}^{\mathrm{2}} {r}^{\mathrm{2}} −\left({p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} \right){k}^{\mathrm{2}} +\mathrm{2}{k}^{\mathrm{3}} =\mathrm{0} \\ $$$$\begin{array}{|c|}{\frac{\mathrm{1}}{{k}^{\mathrm{3}} }−\left(\frac{{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} }{{p}^{\mathrm{2}} {q}^{\mathrm{2}} {r}^{\mathrm{2}} }\right)\frac{\mathrm{1}}{{k}}+\frac{\mathrm{2}}{{p}^{\mathrm{2}} {q}^{\mathrm{2}} {r}^{\mathrm{2}} }=\mathrm{0}}\\\hline\end{array} \\ $$$${p}^{\mathrm{2}} {q}^{\mathrm{2}} {r}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{27}}\left({p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} \right)^{\mathrm{3}} \leqslant\mathrm{0}\:\Rightarrow{three}\:{roots} \\ $$$$\frac{\mathrm{1}}{{k}}=\frac{\mathrm{2}}{{pqr}}\sqrt{\frac{{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} }{\mathrm{3}}}\:\mathrm{sin}\:\left\{\frac{\mathrm{2}{n}\pi}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{3}}\:\mathrm{sin}^{−\mathrm{1}} \:{pqr}\left(\frac{\mathrm{3}}{{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} }\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \right\}\:\:\left({n}=\mathrm{0},\mathrm{1},\mathrm{2}\right) \\ $$$${but}\:{suitable}\:{for}\:{maximum}\:\Delta\:{is}\:{only}\: \\ $$$${the}\:{root}\:{with}\:{n}=\mathrm{2}: \\ $$$$\begin{array}{|c|}{\frac{\mathrm{1}}{{k}}=−\frac{\mathrm{2}}{{pqr}}\sqrt{\frac{{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} }{\mathrm{3}}}\:\mathrm{sin}\:\left\{\frac{\pi}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{3}}\:\mathrm{sin}^{−\mathrm{1}} \:{pqr}\left(\frac{\mathrm{3}}{{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} }\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \right\}}\\\hline\end{array} \\ $$$$ \\ $$$$\Delta_{{max}} =\frac{\mathrm{1}}{\mathrm{2}}\left(\sqrt{{p}^{\mathrm{2}} {q}^{\mathrm{2}} −{k}^{\mathrm{2}} }+\sqrt{{q}^{\mathrm{2}} {r}^{\mathrm{2}} −{k}^{\mathrm{2}} }+\sqrt{{r}^{\mathrm{2}} {p}^{\mathrm{2}} −{k}^{\mathrm{2}} }\right) \\ $$$${a}^{\mathrm{2}} ={q}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{qr}\:\mathrm{cos}\:\beta={q}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{k} \\ $$$${a}=\sqrt{{q}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{k}} \\ $$$${b}=\sqrt{{r}^{\mathrm{2}} +{p}^{\mathrm{2}} −\mathrm{2}{k}} \\ $$$${c}=\sqrt{{p}^{\mathrm{2}} +{q}^{\mathrm{2}} −\mathrm{2}{k}} \\ $$$$ \\ $$$$\underline{{example}:\:{p}=\mathrm{10},\:{q}=\mathrm{8},\:{r}=\mathrm{4}} \\ $$$$\Delta_{{max}} \approx\mathrm{67}.\mathrm{303409} \\ $$$${a}\approx\mathrm{11}.\mathrm{084677} \\ $$$${b}\approx\mathrm{12}.\mathrm{604367} \\ $$$${c}\approx\mathrm{14}.\mathrm{382978} \\ $$$${coresponding}\:{equilateral}\:{triangle}: \\ $$$${s}\approx\mathrm{11}.\mathrm{943609},\:\Delta\approx\mathrm{61}.\mathrm{769169} \\ $$

Commented by mr W last updated on 27/Dec/21

Commented by mr W last updated on 27/Dec/21

Commented by mr W last updated on 27/Dec/21

Commented by mr W last updated on 27/Dec/21

Commented by mr W last updated on 27/Dec/21

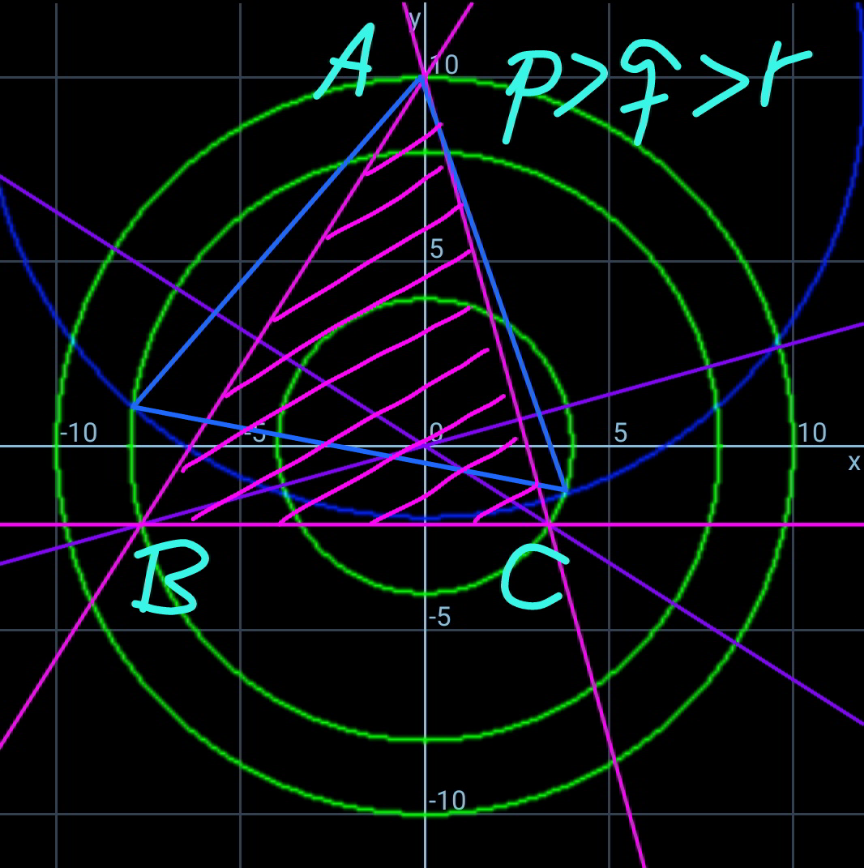
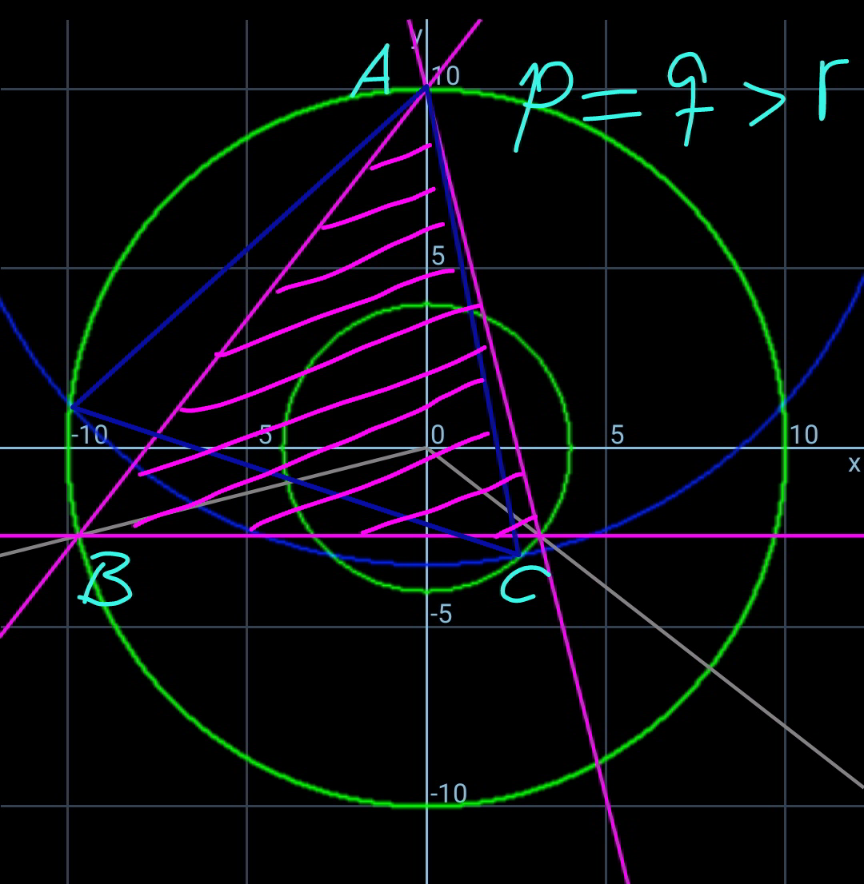
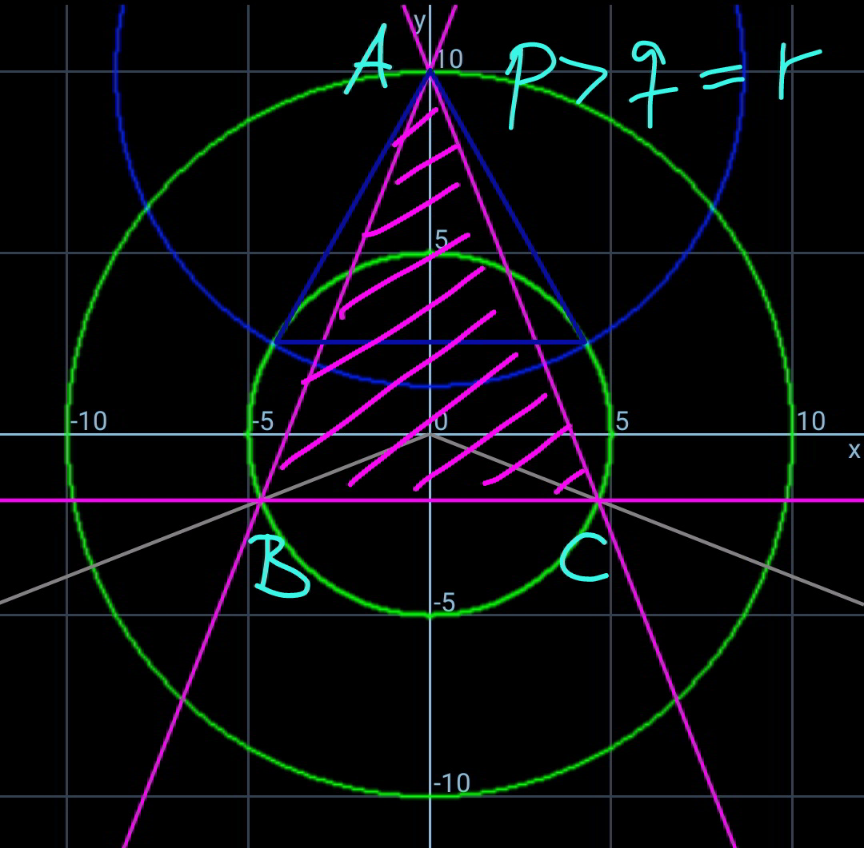
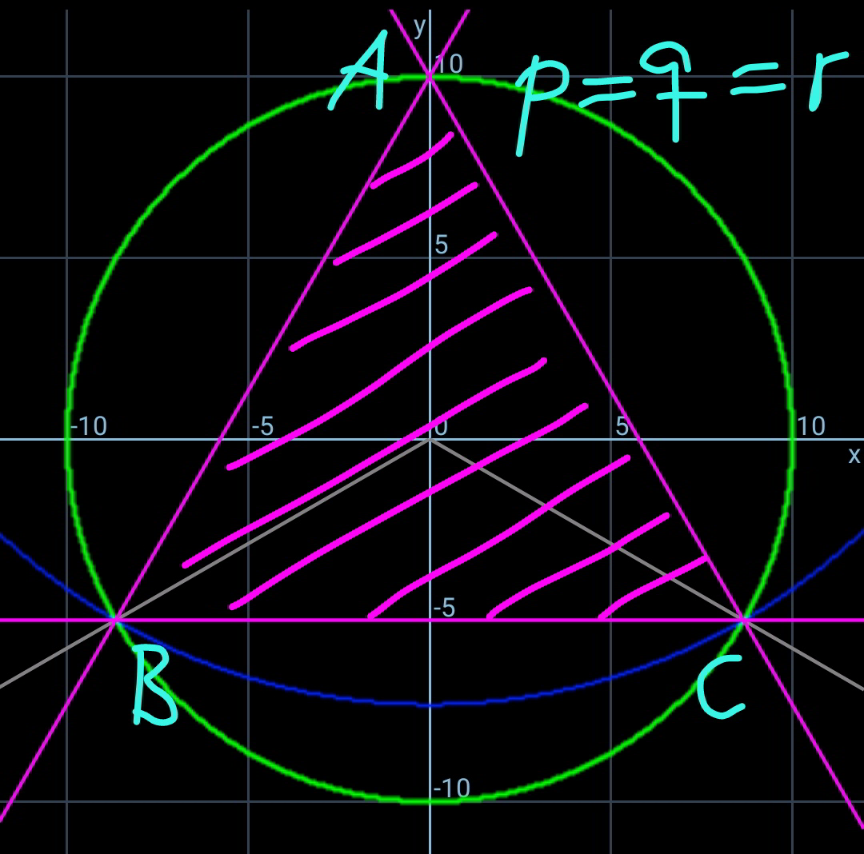
$${we}\:{see}\:{generally}\:{the}\:{coresponding} \\ $$$${equilateral}\:{triangle}\:\left({blue}\:{marked}\:{in}\right. \\ $$$$\left.{diagrams}\right)\:\left({see}\:{also}\:{Q}\mathrm{123040}\right)\:{is}\:{not} \\ $$$${the}\:{triangle}\:{with}\:{the}\:{maximum}\: \\ $$$${area}. \\ $$

Commented by mr W last updated on 27/Dec/21

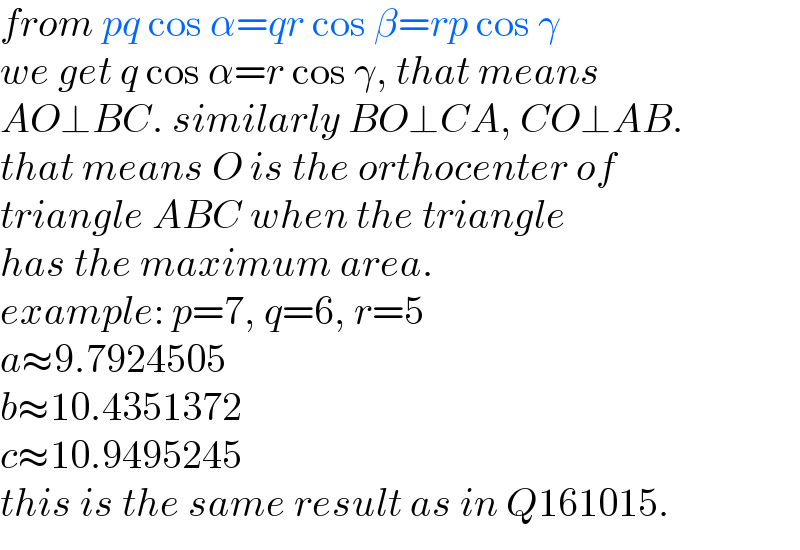
$${from}\:{pq}\:\mathrm{cos}\:\alpha={qr}\:\mathrm{cos}\:\beta={rp}\:\mathrm{cos}\:\gamma \\ $$$${we}\:{get}\:{q}\:\mathrm{cos}\:\alpha={r}\:\mathrm{cos}\:\gamma,\:{that}\:{means} \\ $$$${AO}\bot{BC}.\:{similarly}\:{BO}\bot{CA},\:{CO}\bot{AB}. \\ $$$${that}\:{means}\:{O}\:{is}\:{the}\:{orthocenter}\:{of} \\ $$$${triangle}\:{ABC}\:{when}\:{the}\:{triangle} \\ $$$${has}\:{the}\:{maximum}\:{area}. \\ $$$${example}:\:{p}=\mathrm{7},\:{q}=\mathrm{6},\:{r}=\mathrm{5} \\ $$$${a}\approx\mathrm{9}.\mathrm{7924505} \\ $$$${b}\approx\mathrm{10}.\mathrm{4351372} \\ $$$${c}\approx\mathrm{10}.\mathrm{9495245} \\ $$$${this}\:{is}\:{the}\:{same}\:{result}\:{as}\:{in}\:{Q}\mathrm{161015}. \\ $$

Commented by Tawa11 last updated on 27/Dec/21

$$\mathrm{Great}\:\mathrm{sir}. \\ $$

Commented by behi834171 last updated on 27/Dec/21

$${greaaaaaaaaaat}\:{dear}\:{master}. \\ $$$${thanks}\:{a}\:{lot}. \\ $$

Commented by behi834171 last updated on 27/Dec/21

$$ \\ $$Thank you so much,dear master,for your favor.
I'm here,from now on.

Commented by behi834171 last updated on 27/Dec/21

$$\gamma=\mathrm{90}^{\bullet} \:{is}\:{an}\:\:{interesting}\:{case}.{I}\:{think}. \\ $$

Commented by mr W last updated on 27/Dec/21

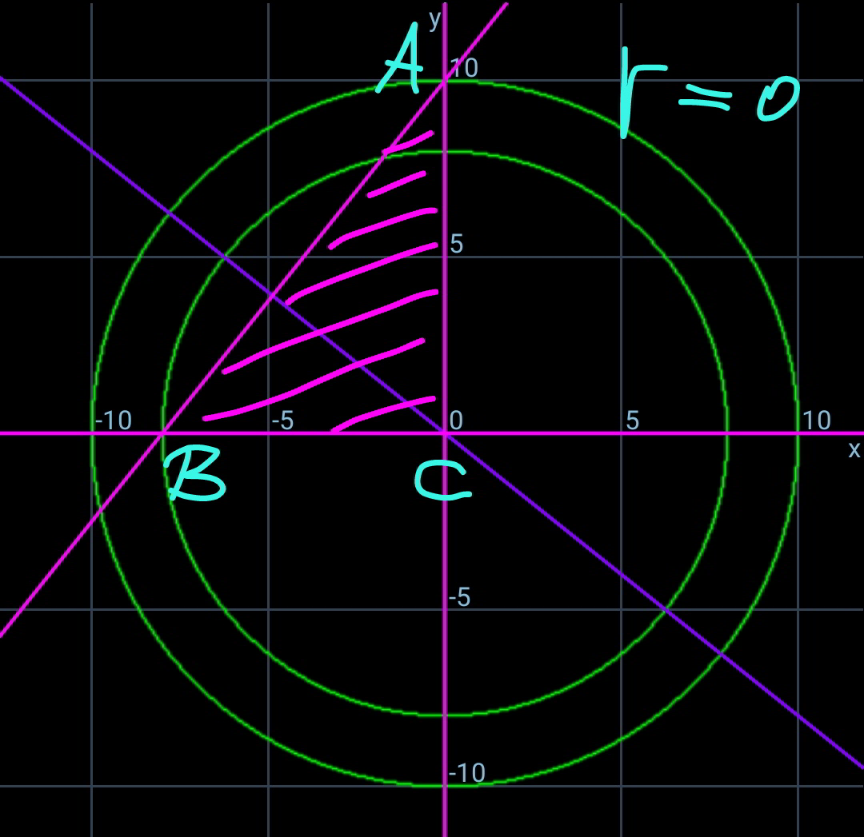
$${when}\:{one}\:{of}\:{p},{q},{r}\:{is}\:{zero},\:{then}\:{the} \\ $$$${coresponding}\:{vertex}\:{is}\:{also}\:{the} \\ $$$${orthocenter}.\:{in}\:{this}\:{case}\:{the}\:{triangle} \\ $$$${is}\:{right}\:{angled}. \\ $$

Commented by mr W last updated on 27/Dec/21

Commented by behi834171 last updated on 27/Dec/21

Commented by behi834171 last updated on 27/Dec/21

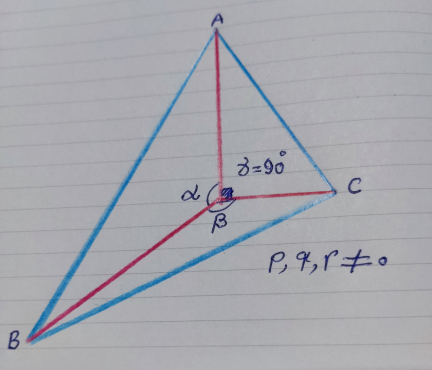
$$\gamma=\mathrm{90}^{\bullet} ,{p},{q},{r}\neq\mathrm{0} \\ $$

Commented by mr W last updated on 27/Dec/21

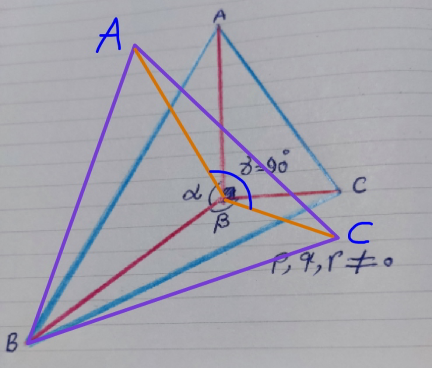
$${the}\:{task}\:{here}\:{is}\:{to}\:{find}\:{the}\:{triangle} \\ $$$${with}\:{maximum}\:{area}\:{when}\:{p},{q},{r}\:{are} \\ $$$${given}.\:{it}\:{is}\:{not}\:{to}\:{find}\:{the}\:{triangle} \\ $$$${with}\:{given}\:\alpha,\beta\:{or}\:\gamma.\: \\ $$$${so}\:{far}\:{as}\:{p},{q},{r}\neq\mathrm{0},\:{the}\:{triangle}\:{with}\: \\ $$$${maxixum}\:{area}\:{can}\:{never}\:{be}\:{a}\:{triangle} \\ $$$${with}\:\gamma=\mathrm{90}°\:! \\ $$$${the}\:{triangle}\:{you}\:{gave}\:{with}\:\gamma=\mathrm{90}°\:{is} \\ $$$${not}\:{the}\:{triangle}\:{which}\:{has}\:{the} \\ $$$${maximum}\:{area}. \\ $$

Commented by mr W last updated on 27/Dec/21

Commented by behi834171 last updated on 27/Dec/21

$${thank}\:{you}\:{very}\:{much}\:{for}\:{your}\:{attention}. \\ $$$${can}\:{we}\:{find}\:{the}\:{sides}\:{of}\:{such}\:{triangle} \\ $$$${whit}:\gamma=\mathrm{90}^{\bullet} \:? \\ $$$${i}\:{have}\:{the}\:{such}\:{unsolved}\:{problem}\:{in}\:{forum} \\ $$$${but}\:{i}\:{don}'{t}\:{remember}\:{its}\:{q}.{ID}… \\ $$

Commented by mr W last updated on 27/Dec/21

$${you}\:{must}\:{specify}\:{exactly}\:{what}\:{is}\:{your} \\ $$$${task}.\:{in}\:{the}\:{current}\:{question}\:{the} \\ $$$${point}\:{O}\:{is}\:{the}\:{orthocenter}\:{of}\:{the} \\ $$$${triangle}.\:{we}\:{can}\:{not}\:{find}\:{a}\:{triangle} \\ $$$${whose}\:{orthocenter}\:{lies}\:{inside}\:{the} \\ $$$${triangle}\:{and}\:{makes}\:{an}\:{angle}\:\gamma=\mathrm{90}°. \\ $$$${this}\:{is}\:{only}\:{the}\:{case}\:{when}\:{the}\: \\ $$$${orthocenter}\:{lies}\:{at}\:{one}\:{vertex}\:{of}\:{a} \\ $$$${right}\:{angled}\:{triangle},\:{but}\:{in}\:{this} \\ $$$${case}\:{one}\:{of}\:{p},{q},{r}\:{is}\:{zero}. \\ $$

Commented by behi834171 last updated on 27/Dec/21

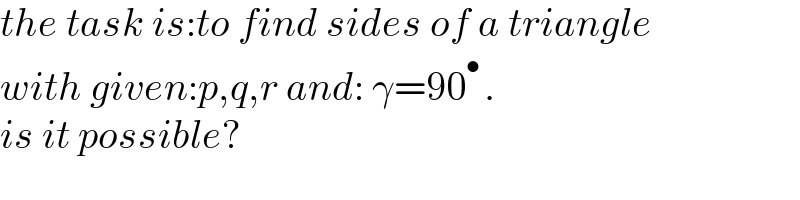
$${the}\:{task}\:{is}:{to}\:{find}\:{sides}\:{of}\:{a}\:{triangle} \\ $$$${with}\:{given}:{p},{q},{r}\:{and}:\:\gamma=\mathrm{90}^{\bullet\:} . \\ $$$${is}\:{it}\:{possible}? \\ $$

Commented by mr W last updated on 27/Dec/21

$${then}\:{infinite}\:{triangles}\:{are}\:{possible}. \\ $$$${only}\:{AC}=\sqrt{{r}^{\mathrm{2}} +{p}^{\mathrm{2}} }\:{is}\:{fixed}. \\ $$

Commented by mr W last updated on 27/Dec/21

Terms of Service
Privacy Policy
Contact: info@tinkutara.com