Question Number 162490 by amin96 last updated on 29/Dec/21
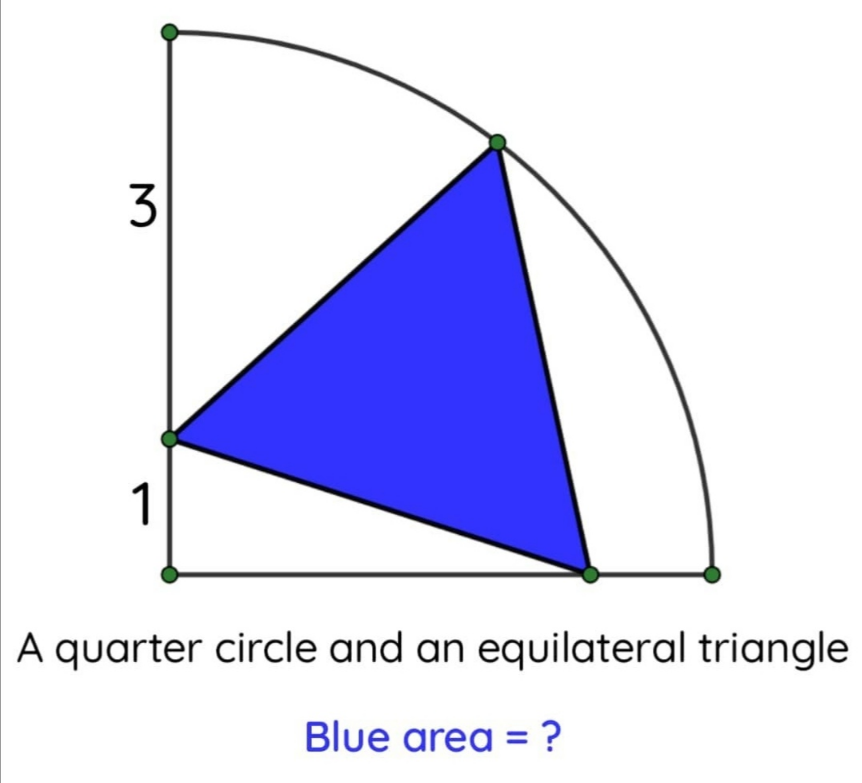
Answered by mr W last updated on 30/Dec/21
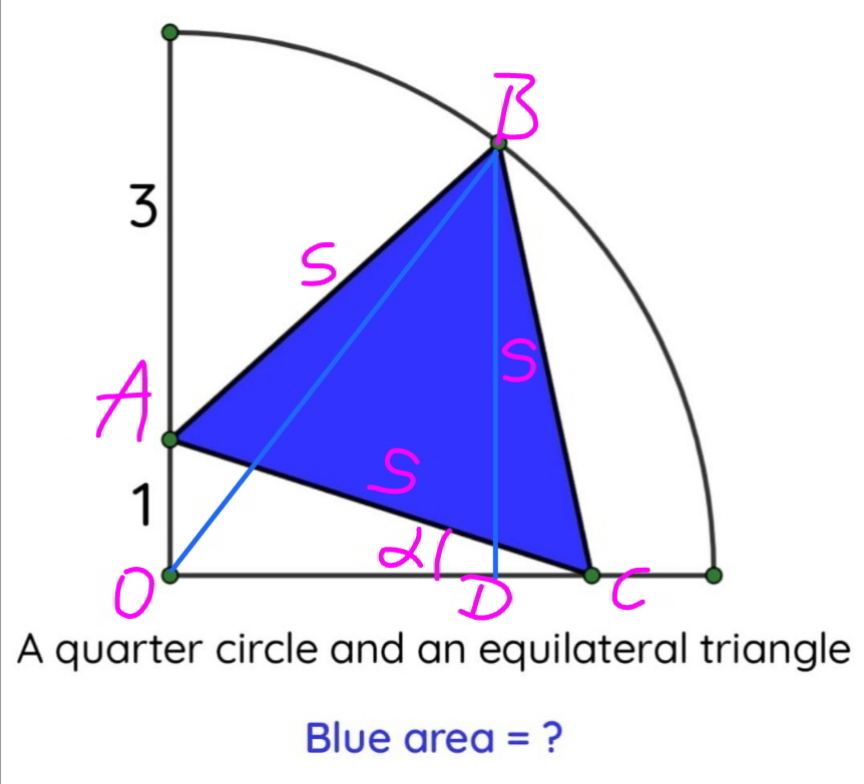
Commented by mr W last updated on 30/Dec/21

$${R}=\mathrm{4}={radius} \\ $$$${OA}={a}=\mathrm{1} \\ $$$${s}={side}\:{length}\:{of}\:{equilateral} \\ $$$${OC}=\sqrt{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} } \\ $$$${DC}={s}\:\mathrm{cos}\:\left(\frac{\pi}{\mathrm{3}}+\alpha\right)=\frac{\mathrm{1}}{\mathrm{2}}\left({s}\:\mathrm{cos}\:\alpha−\sqrt{\mathrm{3}}\:{s}\:\mathrm{sin}\:\alpha\right) \\ $$$$\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\left(\sqrt{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} }−\sqrt{\mathrm{3}}\:{a}\right) \\ $$$${DB}={s}\:\mathrm{sin}\:\left(\frac{\pi}{\mathrm{3}}+\alpha\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\:{s}\:\mathrm{cos}\:\alpha+{s}\:\mathrm{sin}\:\alpha\right) \\ $$$$\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\left(\sqrt{\mathrm{3}\left({s}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)}+{a}\right) \\ $$$${OD}=\sqrt{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} }−\:\frac{\mathrm{1}}{\mathrm{2}}\left(\sqrt{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} }−\sqrt{\mathrm{3}}\:{a}\right) \\ $$$$\:\:\:\:\:\:\:=\:\frac{\mathrm{1}}{\mathrm{2}}\left(\sqrt{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} }+\sqrt{\mathrm{3}}\:{a}\right) \\ $$$${OD}^{\mathrm{2}} +{DB}^{\mathrm{2}} ={OB}^{\mathrm{2}} \\ $$$$\:\frac{\mathrm{1}}{\mathrm{4}}\left(\sqrt{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} }+\sqrt{\mathrm{3}}\:{a}\right)^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{4}}\left(\sqrt{\mathrm{3}\left({s}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)}+{a}\right)^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$$\:{s}^{\mathrm{2}} −{a}^{\mathrm{2}} +\mathrm{3}{a}^{\mathrm{2}} +\mathrm{2}{a}\sqrt{\mathrm{3}\left({s}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)}+\mathrm{3}\left({s}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)+{a}^{\mathrm{2}} +\mathrm{2}{a}\sqrt{\mathrm{3}\left({s}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)}=\mathrm{4}{R}^{\mathrm{2}} \\ $$$$\:{a}\sqrt{\mathrm{3}\left({s}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)}={R}^{\mathrm{2}} −{s}^{\mathrm{2}} \\ $$$$\:\mathrm{3}{a}^{\mathrm{2}} \left({s}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)={s}^{\mathrm{4}} +{R}^{\mathrm{4}} −\mathrm{2}{R}^{\mathrm{2}} {s}^{\mathrm{2}} \\ $$$$\:{s}^{\mathrm{4}} −\left(\mathrm{2}{R}^{\mathrm{2}} +\mathrm{3}{a}^{\mathrm{2}} \right){s}^{\mathrm{2}} +{R}^{\mathrm{4}} +\mathrm{3}{a}^{\mathrm{4}} =\mathrm{0} \\ $$$${s}^{\mathrm{2}} =\frac{\mathrm{2}{R}^{\mathrm{2}} +\mathrm{3}{a}^{\mathrm{2}} −\sqrt{\left(\mathrm{2}{R}^{\mathrm{2}} +\mathrm{3}{a}^{\mathrm{2}} \right)^{\mathrm{2}} −\mathrm{4}\left({R}^{\mathrm{4}} +\mathrm{3}{a}^{\mathrm{4}} \right)}}{\mathrm{2}} \\ $$$$\:\:\:\:=\frac{\mathrm{2}{R}^{\mathrm{2}} +\mathrm{3}{a}^{\mathrm{2}} −{a}\sqrt{\mathrm{3}\left(\mathrm{4}{R}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)}}{\mathrm{2}} \\ $$$${with}\:{R}=\mathrm{4},\:{a}=\mathrm{1}: \\ $$$${s}^{\mathrm{2}} =\frac{\mathrm{2}×\mathrm{4}^{\mathrm{2}} +\mathrm{3}×\mathrm{1}^{\mathrm{2}} −\mathrm{1}\sqrt{\mathrm{3}\left(\mathrm{4}×\mathrm{4}^{\mathrm{2}} −\mathrm{1}^{\mathrm{2}} \right)}}{\mathrm{2}}=\frac{\mathrm{35}−\mathrm{3}\sqrt{\mathrm{21}}}{\mathrm{2}} \\ $$$${area}\:{of}\:{blue}\:{equilateral} \\ $$$${A}_{{blue}} =\frac{\sqrt{\mathrm{3}}{s}^{\mathrm{2}} }{\mathrm{4}}=\frac{\sqrt{\mathrm{3}}\left(\mathrm{35}−\mathrm{3}\sqrt{\mathrm{21}}\right)}{\mathrm{8}}=\frac{\mathrm{35}\sqrt{\mathrm{3}}−\mathrm{9}\sqrt{\mathrm{7}}}{\mathrm{8}}\approx\mathrm{4}.\mathrm{601} \\ $$$$ \\ $$$$=================== \\ $$$${let}\:\xi=\frac{{a}}{{R}} \\ $$$$\left(\frac{{s}}{{R}}\right)^{\mathrm{2}} =\frac{\mathrm{2}+\mathrm{3}\xi^{\mathrm{2}} −\xi\sqrt{\mathrm{3}\left(\mathrm{4}−\xi^{\mathrm{2}} \right)}}{\mathrm{2}} \\ $$$$\left(\frac{{s}}{{R}}\right)_{{max}} =\mathrm{1}\:{at}\:\xi=\mathrm{0} \\ $$$$\left(\frac{{s}}{{R}}\right)_{{min}} =\sqrt{\mathrm{3}}−\mathrm{1}\:{at}\:\xi=\frac{\sqrt{\mathrm{3}}−\mathrm{1}}{\:\sqrt{\mathrm{2}}} \\ $$
Commented by mr W last updated on 30/Dec/21
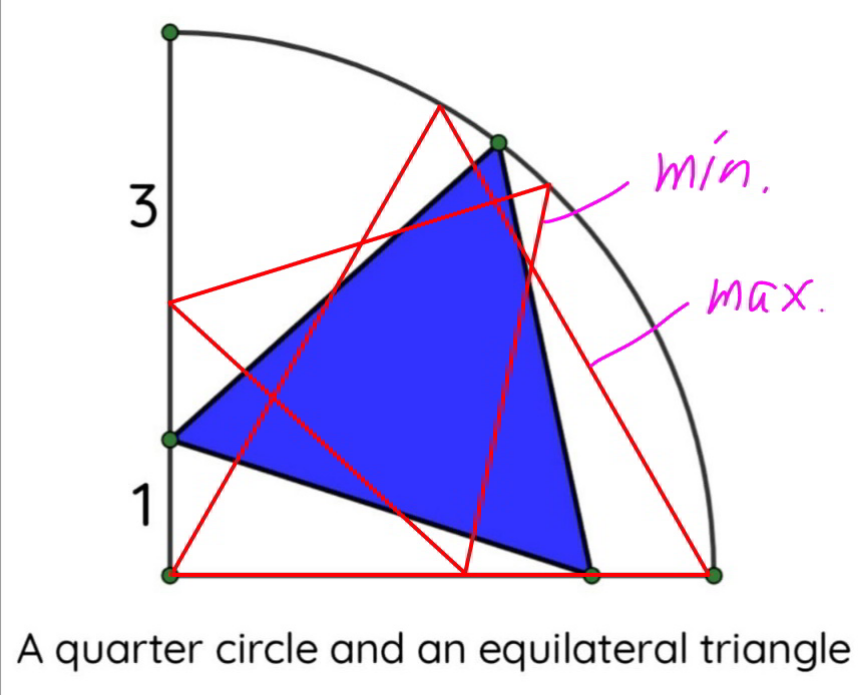
Commented by amin96 last updated on 30/Dec/21

$$\boldsymbol{{greaat}}\:\boldsymbol{{sir}} \\ $$
Commented by Tawa11 last updated on 30/Dec/21

$$\mathrm{Great}\:\mathrm{sir} \\ $$
