Question-163048

Question Number 163048 by mnjuly1970 last updated on 03/Jan/22

Answered by mahdipoor last updated on 03/Jan/22

{s i n}^{2} x = m {c o s}^{2} x = n

\Rightarrow m^{^{'}} = - n^{^{'}} = 2 c o s x . s i n x

f^{'} = ({n m}^{3} + {m n}^{3})^{'} = m^{'} (3 {n m}^{2} + n^{3}) + n^{'} (3 {m n}^{2} + m^{3}) =

2 c o s x . s i n x (n^{3} - 3 {m n}^{2} + 3 m^{2} n - m^{3}) =

s i n 2 x {(n - m)}^{3} =

s i n 2 x {({c o s}^{2} x - {s i n}^{2} x)}^{3} =

s i n 2 x . {c o s}^{3} 2 x = 0

\Rightarrow x = \frac{k π}{4} + \frac{π}{2}

{\begin{cases} s i n (\frac{k π}{4} + \frac{π}{2}) = c o s (\frac{k π}{4}) \\ c o s (\frac{k π}{4} + \frac{π}{2}) = - s i n (\frac{k π}{2}) \end{cases}

f (\frac{k π}{4} + \frac{π}{2}) = \frac{1}{4} {s i n}^{2} (\frac{k π}{2}) ({s i n}^{4} (\frac{k π}{4}) + {c o s}^{4} (\frac{k π}{4}))

= {\begin{cases} 2 ∣ k f = 0 \\ 2 ∤ k f = + \frac{1}{8} \end{cases}

R_{f} = [0, 0.125]

Commented by mnjuly1970 last updated on 03/Jan/22

m e r c e y s i r m a h d i p o o r

Answered by tounghoungko last updated on 03/Jan/22

f (x) = \sin^{2} x \cos^{2} x (\sin^{4} x + \cos^{4} x)

f (x) = \frac{1}{4} \sin^{2} 2 x (1 - 2 \sin^{2} x \cos^{2} x)

f (x) = \frac{1}{4} \sin^{2} 2 x (1 - \frac{1}{2} \sin^{2} 2 x)

f (x) = \frac{1}{4} \sin^{2} 2 x - \frac{1}{8} \sin^{4} 2 x

f (x) = - \frac{1}{8} (\sin^{4} 2 x - 2 \sin^{2} 2 x + 1) + \frac{1}{8}

f (x) = - \frac{1}{8} {(\sin^{2} 2 x - 1)}^{2} + \frac{1}{8}

w h e r e 0 ⩽ \sin^{2} 2 x ⩽ 1

w h e n {\begin{cases} \sin^{2} 2 x = 0 \Rightarrow f = 0 \\ \sin^{2} 2 x = 1 \Rightarrow f = \frac{1}{8} \end{cases}

R a n g e f \Rightarrow 0 ⩽ f ⩽ \frac{1}{8}

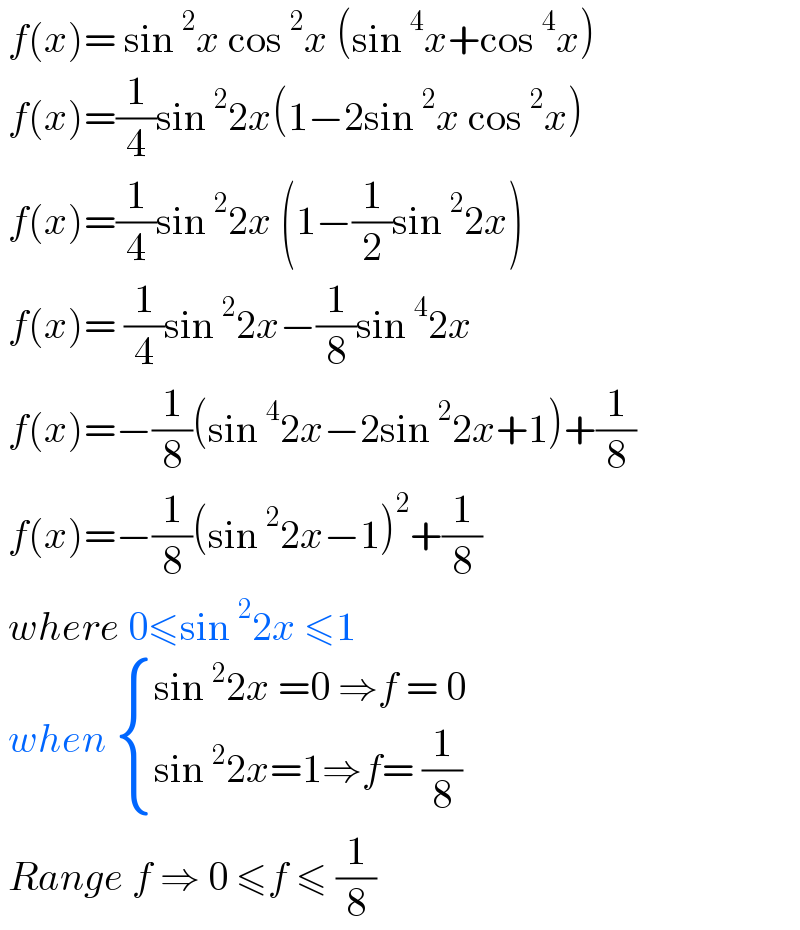
Commented by mnjuly1970 last updated on 03/Jan/22

v e r y n i c e s o l u t i o n s i r

Answered by abdullahhhhh last updated on 03/Jan/22

Commented by mnjuly1970 last updated on 03/Jan/22

t h a n k s a l o t m a s t e r