Menu Close

Question-16310

Tinku Tara 0 Comments
Pin




Question Number 16310 by tawa tawa last updated on 20/Jun/17
Commented by tawa tawa last updated on 20/Jun/17
please i need the solutions urgent sirs. i really appreciate youreffort. God bless you sirs.
$$\mathrm{please}\:\mathrm{i}\:\mathrm{need}\:\mathrm{the}\:\mathrm{solutions}\:\mathrm{urgent}\:\mathrm{sirs}.\:\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{youreffort}. \\ $$$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sirs}. \\ $$
Commented by tawa tawa last updated on 20/Jun/17
a) The acceleration of a package sliding at point A is 3m/s^2 . Assuming that the coefficient of kinectic friction is the same for each section, determine the acceleration of the package at point B. b) The two blocks shown are originally at rest. Neglecting the masses of the pulleys and the effect of friction in the pulleys and assuming that the coefficients of friction between block A and the horizontal surface are μ_s = 0.25 and μ_k = 0.20, determine (a) the acceleration of each block (b) the tension in the cable. mass of A = 30 kg mass of B = 25 kg
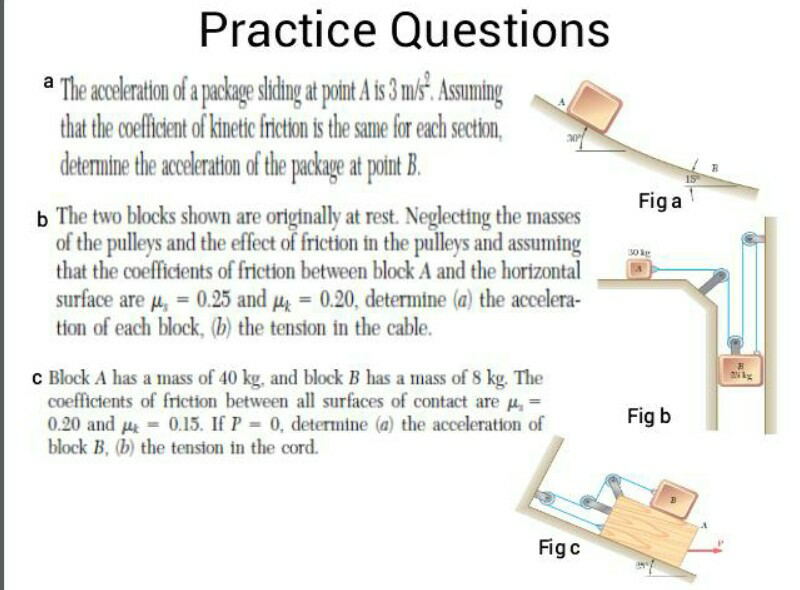
$$\left.\mathrm{a}\right)\:\mathrm{The}\:\mathrm{acceleration}\:\mathrm{of}\:\mathrm{a}\:\mathrm{package}\:\mathrm{sliding}\:\mathrm{at}\:\mathrm{point}\:\mathrm{A}\:\mathrm{is}\:\mathrm{3m}/\mathrm{s}^{\mathrm{2}} .\:\mathrm{Assuming}\:\mathrm{that} \\ $$$$\mathrm{the}\:\mathrm{coefficient}\:\mathrm{of}\:\mathrm{kinectic}\:\mathrm{friction}\:\mathrm{is}\:\mathrm{the}\:\mathrm{same}\:\mathrm{for}\:\mathrm{each}\:\mathrm{section},\:\mathrm{determine} \\ $$$$\mathrm{the}\:\mathrm{acceleration}\:\mathrm{of}\:\mathrm{the}\:\mathrm{package}\:\mathrm{at}\:\mathrm{point}\:\mathrm{B}. \\ $$$$ \\ $$$$\left.\mathrm{b}\right) \\ $$$$\mathrm{The}\:\mathrm{two}\:\mathrm{blocks}\:\mathrm{shown}\:\mathrm{are}\:\mathrm{originally}\:\mathrm{at}\:\mathrm{rest}.\:\mathrm{Neglecting}\:\mathrm{the}\:\mathrm{masses}\:\mathrm{of}\:\mathrm{the}\: \\ $$$$\mathrm{pulleys}\:\mathrm{and}\:\mathrm{the}\:\mathrm{effect}\:\mathrm{of}\:\mathrm{friction}\:\mathrm{in}\:\mathrm{the}\:\mathrm{pulleys}\:\mathrm{and}\:\mathrm{assuming}\:\mathrm{that}\:\mathrm{the}\: \\ $$$$\mathrm{coefficients}\:\mathrm{of}\:\mathrm{friction}\:\mathrm{between}\:\mathrm{block}\:\mathrm{A}\:\mathrm{and}\:\mathrm{the}\:\mathrm{horizontal}\:\mathrm{surface}\:\mathrm{are} \\ $$$$\mu_{\mathrm{s}} \:=\:\mathrm{0}.\mathrm{25}\:\mathrm{and}\:\mu_{\mathrm{k}} \:=\:\mathrm{0}.\mathrm{20},\:\mathrm{determine} \\ $$$$\left(\mathrm{a}\right)\:\mathrm{the}\:\mathrm{acceleration}\:\mathrm{of}\:\mathrm{each}\:\mathrm{block}\: \\ $$$$\left(\mathrm{b}\right)\:\mathrm{the}\:\mathrm{tension}\:\mathrm{in}\:\mathrm{the}\:\mathrm{cable}. \\ $$$$ \\ $$$$\mathrm{mass}\:\mathrm{of}\:\mathrm{A}\:=\:\mathrm{30}\:\mathrm{kg} \\ $$$$\mathrm{mass}\:\mathrm{of}\:\mathrm{B}\:=\:\mathrm{25}\:\mathrm{kg} \\ $$
Commented by tawa tawa last updated on 20/Jun/17
c) Block A has a mass of 40 kg. and block B has a mass of 8kg. The coeficients of friction between all surfaces of contact are μ_s = 0.20 and μ_k = 0.15. If P = 0, Determine a) The acceleration of block B b) The tension in the cord. the angle below the diagram is 25°.
$$\left.\mathrm{c}\right) \\ $$$$\mathrm{Block}\:\mathrm{A}\:\mathrm{has}\:\mathrm{a}\:\mathrm{mass}\:\mathrm{of}\:\mathrm{40}\:\mathrm{kg}.\:\mathrm{and}\:\mathrm{block}\:\mathrm{B}\:\mathrm{has}\:\mathrm{a}\:\mathrm{mass}\:\mathrm{of}\:\mathrm{8kg}.\:\mathrm{The}\:\mathrm{coeficients} \\ $$$$\mathrm{of}\:\mathrm{friction}\:\mathrm{between}\:\mathrm{all}\:\mathrm{surfaces}\:\mathrm{of}\:\mathrm{contact}\:\mathrm{are}\:\mu_{\mathrm{s}} \:=\:\mathrm{0}.\mathrm{20}\:\mathrm{and}\:\mu_{\mathrm{k}} \:=\:\mathrm{0}.\mathrm{15}.\:\mathrm{If}\:\mathrm{P}\:=\:\mathrm{0}, \\ $$$$\mathrm{Determine}\: \\ $$$$\left.\mathrm{a}\right)\:\mathrm{The}\:\mathrm{acceleration}\:\mathrm{of}\:\mathrm{block}\:\mathrm{B} \\ $$$$\left.\mathrm{b}\right)\:\mathrm{The}\:\mathrm{tension}\:\mathrm{in}\:\mathrm{the}\:\mathrm{cord}. \\ $$$$ \\ $$$$\mathrm{the}\:\mathrm{angle}\:\mathrm{below}\:\mathrm{the}\:\mathrm{diagram}\:\mathrm{is}\:\:\:\mathrm{25}°. \\ $$
Answered by mrW1 last updated on 20/Jun/17
a) ma=mg×sin α−μ×mg×cos α a=g(sin α−μcos α) at point A: a_A =g(sin 30−μcos 30) μ=((sin 30−(a_A /g))/(cos 30))=(((1/2)−(3/(10)))/((√3)/2)) =(2/(5(√3)))=0.23 at point B: a_B =g(sin 15−μcos 15) =10(sin 15−0.23×cos 15)=0.37 m/s^2 b) (b) tension in rope T=((m_B g)/3)=((25×10)/3)=83.3 N (a) T>μ_s m_A g=0.25×30×10=75 N ⇒A will move. m_A a=T−μ_k m_A g a=((83.3−0.2×30×10)/(30))=0.78 c) let T=tension in cord Block B: T−m_B g×sin α+μ_k m_B g×cos α=m_B a_B T=m_B g(sin α−μ_k ×cos α+(a_B /g)) T=m_B g×sin α−μ_k m_B gcos α+m_B ga′ with a′=(a_B /g)=(a_A /g) Block A: m_A g×sin α−2T+T−μ_k m_B g×cos α−μ_k (m_A +m_B )g×cos α=m_A a_A m_A g×sin α−m_B g(sin α−μ_k cos α+a′)−μ_k (m_A +2m_B )g×cos α=m_A a_A m_A g×sin α−m_B g×sin α−μ_k m_B gcos α+m_B ga′−μ_k (m_A +2m_B )g×cos α=m_A a_A (m_A −m_B )×sin α−μ_k (m_A +3m_B )×cos α=(m_A −m_B )a′ a′=(((m_A −m_B )×sin α−μ_k (m_A +3m_B )×cos α)/((m_A −m_B ))) =sin α−μ_k ((m_A +3m_B )/(m_A −m_B )) cos α =sin 25−0.15×((40+3×8)/(40−8))×cos 25 =sin 25−0.15×2×cos 25 =0.15 ⇒a_B =a′×g=0.15×10=1.5 m/s^2 T=m_B g(sin α−μ_k ×cos α+(a_B /g)) =8×10(sin 25−0.15×cos 25+0.15) =34.9 N
$$\left.\mathrm{a}\right) \\ $$$$\mathrm{ma}=\mathrm{mg}×\mathrm{sin}\:\alpha−\mu×\mathrm{mg}×\mathrm{cos}\:\alpha \\ $$$$\mathrm{a}=\mathrm{g}\left(\mathrm{sin}\:\alpha−\mu\mathrm{cos}\:\alpha\right) \\ $$$$\mathrm{at}\:\mathrm{point}\:\mathrm{A}: \\ $$$$\mathrm{a}_{\mathrm{A}} =\mathrm{g}\left(\mathrm{sin}\:\mathrm{30}−\mu\mathrm{cos}\:\mathrm{30}\right) \\ $$$$\mu=\frac{\mathrm{sin}\:\mathrm{30}−\frac{\mathrm{a}_{\mathrm{A}} }{\mathrm{g}}}{\mathrm{cos}\:\mathrm{30}}=\frac{\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{3}}{\mathrm{10}}}{\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}\:=\frac{\mathrm{2}}{\mathrm{5}\sqrt{\mathrm{3}}}=\mathrm{0}.\mathrm{23} \\ $$$$\mathrm{at}\:\mathrm{point}\:\mathrm{B}: \\ $$$$\mathrm{a}_{\mathrm{B}} =\mathrm{g}\left(\mathrm{sin}\:\mathrm{15}−\mu\mathrm{cos}\:\mathrm{15}\right) \\ $$$$=\mathrm{10}\left(\mathrm{sin}\:\mathrm{15}−\mathrm{0}.\mathrm{23}×\mathrm{cos}\:\mathrm{15}\right)=\mathrm{0}.\mathrm{37}\:\mathrm{m}/\mathrm{s}^{\mathrm{2}} \\ $$$$ \\ $$$$\left.\mathrm{b}\right) \\ $$$$\left(\mathrm{b}\right)\:\mathrm{tension}\:\mathrm{in}\:\mathrm{rope}\:\mathrm{T}=\frac{\mathrm{m}_{\mathrm{B}} \mathrm{g}}{\mathrm{3}}=\frac{\mathrm{25}×\mathrm{10}}{\mathrm{3}}=\mathrm{83}.\mathrm{3}\:\mathrm{N} \\ $$$$\left(\mathrm{a}\right)\:\mathrm{T}>\mu_{\mathrm{s}} \mathrm{m}_{\mathrm{A}} \mathrm{g}=\mathrm{0}.\mathrm{25}×\mathrm{30}×\mathrm{10}=\mathrm{75}\:\mathrm{N} \\ $$$$\Rightarrow\mathrm{A}\:\mathrm{will}\:\mathrm{move}. \\ $$$$\:\mathrm{m}_{\mathrm{A}} \mathrm{a}=\mathrm{T}−\mu_{\mathrm{k}} \mathrm{m}_{\mathrm{A}} \mathrm{g} \\ $$$$\mathrm{a}=\frac{\mathrm{83}.\mathrm{3}−\mathrm{0}.\mathrm{2}×\mathrm{30}×\mathrm{10}}{\mathrm{30}}=\mathrm{0}.\mathrm{78} \\ $$$$ \\ $$$$\left.\mathrm{c}\right) \\ $$$$\mathrm{let}\:\mathrm{T}=\mathrm{tension}\:\mathrm{in}\:\mathrm{cord} \\ $$$$\mathrm{Block}\:\mathrm{B}: \\ $$$$\mathrm{T}−\mathrm{m}_{\mathrm{B}} \mathrm{g}×\mathrm{sin}\:\alpha+\mu_{\mathrm{k}} \mathrm{m}_{\mathrm{B}} \mathrm{g}×\mathrm{cos}\:\alpha=\mathrm{m}_{\mathrm{B}} \mathrm{a}_{\mathrm{B}} \\ $$$$\mathrm{T}=\mathrm{m}_{\mathrm{B}} \mathrm{g}\left(\mathrm{sin}\:\alpha−\mu_{\mathrm{k}} ×\mathrm{cos}\:\alpha+\frac{\mathrm{a}_{\mathrm{B}} }{\mathrm{g}}\right) \\ $$$$\mathrm{T}=\mathrm{m}_{\mathrm{B}} \mathrm{g}×\mathrm{sin}\:\alpha−\mu_{\mathrm{k}} \mathrm{m}_{\mathrm{B}} \mathrm{gcos}\:\alpha+\mathrm{m}_{\mathrm{B}} \mathrm{ga}' \\ $$$$\mathrm{with}\:\mathrm{a}'=\frac{\mathrm{a}_{\mathrm{B}} }{\mathrm{g}}=\frac{\mathrm{a}_{\mathrm{A}} }{\mathrm{g}} \\ $$$$ \\ $$$$\mathrm{Block}\:\mathrm{A}: \\ $$$$\mathrm{m}_{\mathrm{A}} \mathrm{g}×\mathrm{sin}\:\alpha−\mathrm{2T}+\mathrm{T}−\mu_{\mathrm{k}} \mathrm{m}_{\mathrm{B}} \mathrm{g}×\mathrm{cos}\:\alpha−\mu_{\mathrm{k}} \left(\mathrm{m}_{\mathrm{A}} +\mathrm{m}_{\mathrm{B}} \right)\mathrm{g}×\mathrm{cos}\:\alpha=\mathrm{m}_{\mathrm{A}} \mathrm{a}_{\mathrm{A}} \\ $$$$\mathrm{m}_{\mathrm{A}} \mathrm{g}×\mathrm{sin}\:\alpha−\mathrm{m}_{\mathrm{B}} \mathrm{g}\left(\mathrm{sin}\:\alpha−\mu_{\mathrm{k}} \mathrm{cos}\:\alpha+\mathrm{a}'\right)−\mu_{\mathrm{k}} \left(\mathrm{m}_{\mathrm{A}} +\mathrm{2m}_{\mathrm{B}} \right)\mathrm{g}×\mathrm{cos}\:\alpha=\mathrm{m}_{\mathrm{A}} \mathrm{a}_{\mathrm{A}} \\ $$$$\mathrm{m}_{\mathrm{A}} \mathrm{g}×\mathrm{sin}\:\alpha−\mathrm{m}_{\mathrm{B}} \mathrm{g}×\mathrm{sin}\:\alpha−\mu_{\mathrm{k}} \mathrm{m}_{\mathrm{B}} \mathrm{gcos}\:\alpha+\mathrm{m}_{\mathrm{B}} \mathrm{ga}'−\mu_{\mathrm{k}} \left(\mathrm{m}_{\mathrm{A}} +\mathrm{2m}_{\mathrm{B}} \right)\mathrm{g}×\mathrm{cos}\:\alpha=\mathrm{m}_{\mathrm{A}} \mathrm{a}_{\mathrm{A}} \\ $$$$\left(\mathrm{m}_{\mathrm{A}} −\mathrm{m}_{\mathrm{B}} \right)×\mathrm{sin}\:\alpha−\mu_{\mathrm{k}} \left(\mathrm{m}_{\mathrm{A}} +\mathrm{3m}_{\mathrm{B}} \right)×\mathrm{cos}\:\alpha=\left(\mathrm{m}_{\mathrm{A}} −\mathrm{m}_{\mathrm{B}} \right)\mathrm{a}' \\ $$$$\mathrm{a}'=\frac{\left(\mathrm{m}_{\mathrm{A}} −\mathrm{m}_{\mathrm{B}} \right)×\mathrm{sin}\:\alpha−\mu_{\mathrm{k}} \left(\mathrm{m}_{\mathrm{A}} +\mathrm{3m}_{\mathrm{B}} \right)×\mathrm{cos}\:\alpha}{\left(\mathrm{m}_{\mathrm{A}} −\mathrm{m}_{\mathrm{B}} \right)} \\ $$$$=\mathrm{sin}\:\alpha−\mu_{\mathrm{k}} \:\frac{\mathrm{m}_{\mathrm{A}} +\mathrm{3m}_{\mathrm{B}} }{\mathrm{m}_{\mathrm{A}} −\mathrm{m}_{\mathrm{B}} }\:\mathrm{cos}\:\alpha \\ $$$$=\mathrm{sin}\:\mathrm{25}−\mathrm{0}.\mathrm{15}×\frac{\mathrm{40}+\mathrm{3}×\mathrm{8}}{\mathrm{40}−\mathrm{8}}×\mathrm{cos}\:\mathrm{25} \\ $$$$=\mathrm{sin}\:\mathrm{25}−\mathrm{0}.\mathrm{15}×\mathrm{2}×\mathrm{cos}\:\mathrm{25} \\ $$$$=\mathrm{0}.\mathrm{15} \\ $$$$\Rightarrow\mathrm{a}_{\mathrm{B}} =\mathrm{a}'×\mathrm{g}=\mathrm{0}.\mathrm{15}×\mathrm{10}=\mathrm{1}.\mathrm{5}\:\mathrm{m}/\mathrm{s}^{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{T}=\mathrm{m}_{\mathrm{B}} \mathrm{g}\left(\mathrm{sin}\:\alpha−\mu_{\mathrm{k}} ×\mathrm{cos}\:\alpha+\frac{\mathrm{a}_{\mathrm{B}} }{\mathrm{g}}\right) \\ $$$$=\mathrm{8}×\mathrm{10}\left(\mathrm{sin}\:\mathrm{25}−\mathrm{0}.\mathrm{15}×\mathrm{cos}\:\mathrm{25}+\mathrm{0}.\mathrm{15}\right) \\ $$$$=\mathrm{34}.\mathrm{9}\:\mathrm{N} \\ $$
Commented by tawa tawa last updated on 20/Jun/17
wow, i really appreciate sir. i will type it now sir.
$$\mathrm{wow},\:\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{sir}.\:\mathrm{i}\:\mathrm{will}\:\mathrm{type}\:\mathrm{it}\:\mathrm{now}\:\mathrm{sir}. \\ $$
Commented by tawa tawa last updated on 20/Jun/17
God bless you sir.
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
Commented by tawa tawa last updated on 20/Jun/17
God bless you sir. thanks for your time.
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{thanks}\:\mathrm{for}\:\mathrm{your}\:\mathrm{time}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *