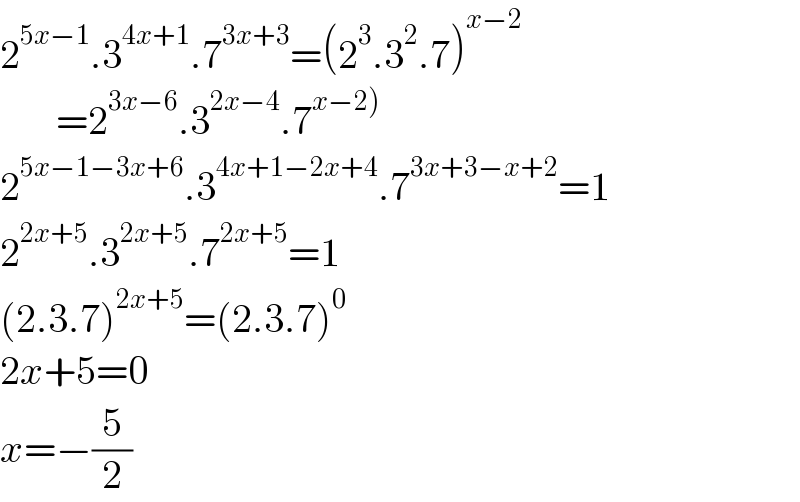Question-163226

Question Number 163226 by nurtani last updated on 05/Jan/22

Commented by Rasheed.Sindhi last updated on 06/Jan/22

Sir mr W, w h y h a v e y o u d e l e t e d y o u r

a n s w e r ?

Commented by mr W last updated on 06/Jan/22

s o m e t i m e s i d e l e t e m y a n s w e r w h e n

i s e e t h a t t h e r e e x i s t s a l r e a d y t h e

s a m e o r s i m i l a r a n s w e r f r o m o t h e r

p e o p l e .

Commented by Rasheed.Sindhi last updated on 06/Jan/22

U n d e r s t o o d s i r!

Answered by cortano1 last updated on 05/Jan/22

2^{5 x - 1} . 3^{4 x + 1} . 7^{3 x + 3} = 2^{3 x - 6} . 3^{2 x - 4} . 7^{x - 2}

\Rightarrow x = - \frac{5}{2}

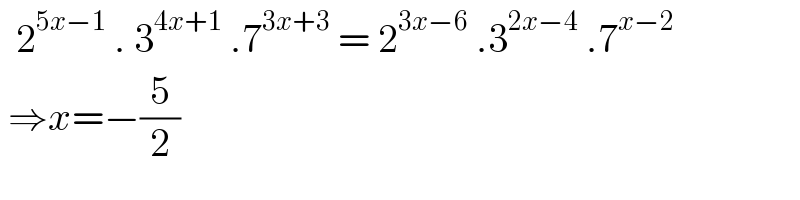
Answered by Rasheed.Sindhi last updated on 05/Jan/22

2^{5 x - 1} . 3^{4 x + 1} . 7^{3 x + 3} = {(2^{3} . 3^{2} . 7)}^{x - 2}

= 2^{3 x - 6} . 3^{2 x - 4} . 7^{x - 2)}

2^{5 x - 1 - 3 x + 6} . 3^{4 x + 1 - 2 x + 4} . 7^{3 x + 3 - x + 2} = 1

2^{2 x + 5} . 3^{2 x + 5} . 7^{2 x + 5} = 1

{(2.3.7)}^{2 x + 5} = {(2.3.7)}^{0}

2 x + 5 = 0

x = - \frac{5}{2}