Question-164842

Question Number 164842 by ajfour last updated on 22/Jan/22

Commented by MJS_new last updated on 22/Jan/22

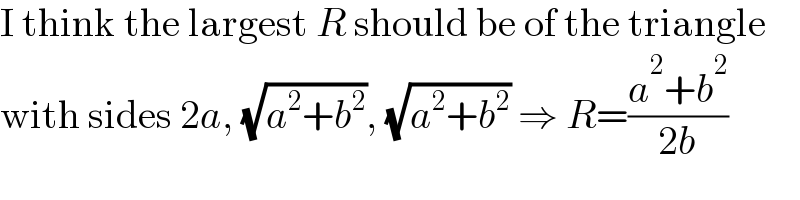
I think the largest R should be of the triangle with sides 2a, (√(a^2 +b^2 )), (√(a^2 +b^2 )) ⇒ R=((a^2 +b^2 )/(2b))

$$\mathrm{I}\:\mathrm{think}\:\mathrm{the}\:\mathrm{largest}\:{R}\:\mathrm{should}\:\mathrm{be}\:\mathrm{of}\:\mathrm{the}\:\mathrm{triangle} \\ $$$$\mathrm{with}\:\mathrm{sides}\:\mathrm{2}{a},\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} },\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:\Rightarrow\:{R}=\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{\mathrm{2}{b}} \\ $$

Commented by ajfour last updated on 22/Jan/22

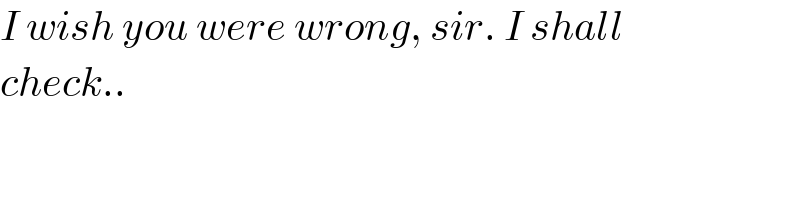
$${I}\:{wish}\:{you}\:{were}\:{wrong},\:{sir}.\:{I}\:{shall} \\ $$$${check}.. \\ $$

Commented by mr W last updated on 22/Jan/22

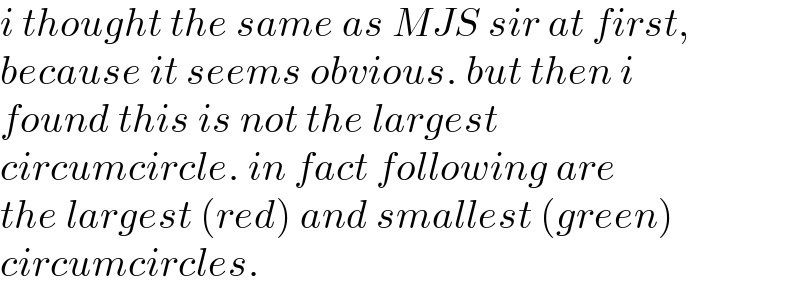
$${i}\:{thought}\:{the}\:{same}\:{as}\:{MJS}\:{sir}\:{at}\:{first}, \\ $$$${because}\:{it}\:{seems}\:{obvious}.\:{but}\:{then}\:{i} \\ $$$${found}\:{this}\:{is}\:{not}\:{the}\:{largest}\: \\ $$$${circumcircle}.\:{in}\:{fact}\:{following}\:{are} \\ $$$${the}\:{largest}\:\left({red}\right)\:{and}\:{smallest}\:\left({green}\right) \\ $$$${circumcircles}. \\ $$

Commented by mr W last updated on 22/Jan/22

Commented by mr W last updated on 22/Jan/22

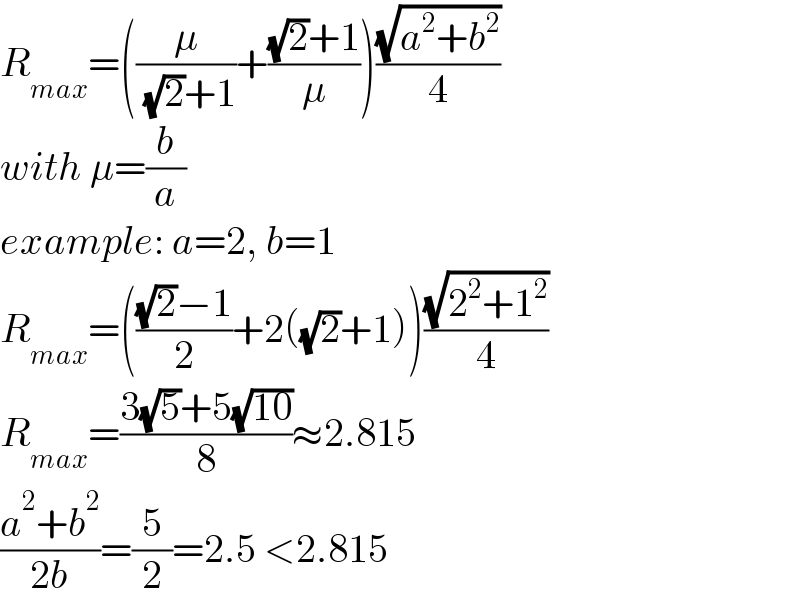
R_(max) =((μ/( (√2)+1))+(((√2)+1)/μ))((√(a^2 +b^2 ))/4) with μ=(b/a) example: a=2, b=1 R_(max) =((((√2)−1)/2)+2((√2)+1))((√(2^2 +1^2 ))/4) R_(max) =((3(√5)+5(√(10)))/8)≈2.815 ((a^2 +b^2 )/(2b))=(5/2)=2.5 <2.815

$${R}_{{max}} =\left(\frac{\mu}{\:\sqrt{\mathrm{2}}+\mathrm{1}}+\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\mu}\right)\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{4}}\: \\ $$$${with}\:\mu=\frac{{b}}{{a}} \\ $$$${example}:\:{a}=\mathrm{2},\:{b}=\mathrm{1} \\ $$$${R}_{{max}} =\left(\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\mathrm{2}}+\mathrm{2}\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)\right)\frac{\sqrt{\mathrm{2}^{\mathrm{2}} +\mathrm{1}^{\mathrm{2}} }}{\mathrm{4}} \\ $$$${R}_{{max}} =\frac{\mathrm{3}\sqrt{\mathrm{5}}+\mathrm{5}\sqrt{\mathrm{10}}}{\mathrm{8}}\approx\mathrm{2}.\mathrm{815} \\ $$$$\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{\mathrm{2}{b}}=\frac{\mathrm{5}}{\mathrm{2}}=\mathrm{2}.\mathrm{5}\:<\mathrm{2}.\mathrm{815} \\ $$

Commented by MJS_new last updated on 23/Jan/22

you′re right. I put a=1 and use (((−1)),(0) ) and ((0),(b) ) as vertices of the fixed side if the Δ ⇒ the maximum R always is with P= ((((√2)/2)),((((√2)/2)b)) ) and the minimum R with Q= (((−((√2)/2))),((−((√2)/2)b)) ) R_(max) =((√(b^2 +1))/(4b))(((√2)−1)b^2 +(√2)+1) R_(min) =((√(b^2 +1))/(4b))(((√2)+1)b^2 +(√2)−1) which represent the same values as you get

$$\mathrm{you}'\mathrm{re}\:\mathrm{right}.\:\mathrm{I}\:\mathrm{put}\:{a}=\mathrm{1}\:\mathrm{and}\:\mathrm{use}\:\begin{pmatrix}{−\mathrm{1}}\\{\mathrm{0}}\end{pmatrix}\:\mathrm{and} \\ $$$$\begin{pmatrix}{\mathrm{0}}\\{{b}}\end{pmatrix}\:\mathrm{as}\:\mathrm{vertices}\:\mathrm{of}\:\mathrm{the}\:\mathrm{fixed}\:\mathrm{side}\:\mathrm{if}\:\mathrm{the}\:\Delta \\ $$$$\Rightarrow\:\mathrm{the}\:\mathrm{maximum}\:{R}\:\mathrm{always}\:\mathrm{is}\:\mathrm{with}\:{P}=\begin{pmatrix}{\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}}\\{\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}{b}}\end{pmatrix} \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{minimum}\:{R}\:\mathrm{with}\:{Q}=\begin{pmatrix}{−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}}\\{−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}{b}}\end{pmatrix} \\ $$$${R}_{{max}} =\frac{\sqrt{{b}^{\mathrm{2}} +\mathrm{1}}}{\mathrm{4}{b}}\left(\left(\sqrt{\mathrm{2}}−\mathrm{1}\right){b}^{\mathrm{2}} +\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$${R}_{{min}} =\frac{\sqrt{{b}^{\mathrm{2}} +\mathrm{1}}}{\mathrm{4}{b}}\left(\left(\sqrt{\mathrm{2}}+\mathrm{1}\right){b}^{\mathrm{2}} +\sqrt{\mathrm{2}}−\mathrm{1}\right) \\ $$$$\mathrm{which}\:\mathrm{represent}\:\mathrm{the}\:\mathrm{same}\:\mathrm{values}\:\mathrm{as}\:\mathrm{you}\:\mathrm{get} \\ $$

Commented by MJS_new last updated on 23/Jan/22

the above R_(min) is wrong for b below a certain border I′ve not exactly found yet

$$\mathrm{the}\:\mathrm{above}\:{R}_{{min}} \:\mathrm{is}\:\mathrm{wrong}\:\mathrm{for}\:{b}\:\mathrm{below}\:\mathrm{a}\:\mathrm{certain} \\ $$$$\mathrm{border}\:\mathrm{I}'\mathrm{ve}\:\mathrm{not}\:\mathrm{exactly}\:\mathrm{found}\:\mathrm{yet} \\ $$

Commented by MJS_new last updated on 23/Jan/22

for b<(√2)−1 we get 2 points Q_1 , Q_2 with x=((b^2 +1±(√(b^4 −6b^2 +1)))/(2(b^2 −1))) and R_(min) is below above value

$$\mathrm{for}\:{b}<\sqrt{\mathrm{2}}−\mathrm{1} \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{2}\:\mathrm{points}\:{Q}_{\mathrm{1}} ,\:{Q}_{\mathrm{2}} \:\mathrm{with}\:{x}=\frac{{b}^{\mathrm{2}} +\mathrm{1}\pm\sqrt{{b}^{\mathrm{4}} −\mathrm{6}{b}^{\mathrm{2}} +\mathrm{1}}}{\mathrm{2}\left({b}^{\mathrm{2}} −\mathrm{1}\right)} \\ $$$$\mathrm{and}\:{R}_{{min}} \:\mathrm{is}\:\mathrm{below}\:\mathrm{above}\:\mathrm{value} \\ $$

Commented by MJS_new last updated on 23/Jan/22

R_(min) =(√(−(((b^2 +1)((b^2 −1)x−b^2 −1)((b^2 −1)(√(1−x^2 ))+b^2 +1))/(8b^2 )))) with above x

$${R}_{{min}} =\sqrt{−\frac{\left({b}^{\mathrm{2}} +\mathrm{1}\right)\left(\left({b}^{\mathrm{2}} −\mathrm{1}\right){x}−{b}^{\mathrm{2}} −\mathrm{1}\right)\left(\left({b}^{\mathrm{2}} −\mathrm{1}\right)\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }+{b}^{\mathrm{2}} +\mathrm{1}\right)}{\mathrm{8}{b}^{\mathrm{2}} }}\:\mathrm{with}\:\mathrm{above}\:{x} \\ $$

Commented by mr W last updated on 23/Jan/22

you are right MJS sir! R_(max) is always at θ=−(π/4). but R_(min) is at θ=((3π)/4) only for (b/a)≥(√2)−1. for (b/a)<(√2)−1 there are two positions for R_(min) , one at θ<((3π)/4) and one at θ>((3π)/4). and in this case R_(min) =((√(a^2 +b^2 ))/2). can you please check if this matches also with your result?

$${you}\:{are}\:{right}\:{MJS}\:{sir}!\:{R}_{{max}} \:{is}\:{always} \\ $$$${at}\:\theta=−\frac{\pi}{\mathrm{4}}.\:{but}\:{R}_{{min}} \:{is}\:{at}\:\theta=\frac{\mathrm{3}\pi}{\mathrm{4}}\:{only} \\ $$$${for}\:\frac{{b}}{{a}}\geqslant\sqrt{\mathrm{2}}−\mathrm{1}.\:{for}\:\frac{{b}}{{a}}<\sqrt{\mathrm{2}}−\mathrm{1}\:{there}\:{are} \\ $$$${two}\:{positions}\:{for}\:{R}_{{min}} ,\:{one}\:{at}\:\theta<\frac{\mathrm{3}\pi}{\mathrm{4}} \\ $$$${and}\:{one}\:{at}\:\theta>\frac{\mathrm{3}\pi}{\mathrm{4}}.\:{and}\:{in}\:{this}\:{case} \\ $$$${R}_{{min}} =\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}}. \\ $$$${can}\:{you}\:{please}\:{check}\:{if}\:{this}\:{matches} \\ $$$${also}\:{with}\:{your}\:{result}? \\ $$

Commented by mr W last updated on 23/Jan/22

Commented by mr W last updated on 23/Jan/22

Commented by mr W last updated on 23/Jan/22

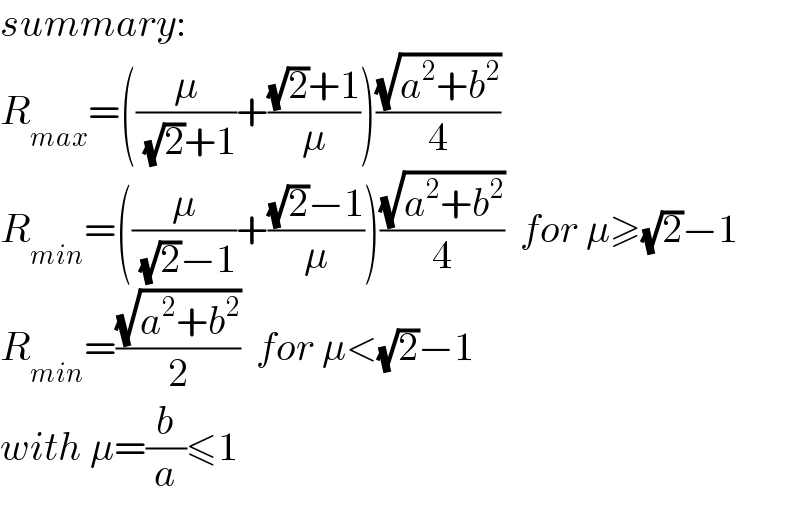
summary: R_(max) =((μ/( (√2)+1))+(((√2)+1)/μ))((√(a^2 +b^2 ))/4) R_(min) =((μ/( (√2)−1))+(((√2)−1)/μ))((√(a^2 +b^2 ))/4) for μ≥(√2)−1 R_(min) =((√(a^2 +b^2 ))/2) for μ<(√2)−1 with μ=(b/a)≤1

$${summary}: \\ $$$${R}_{{max}} =\left(\frac{\mu}{\:\sqrt{\mathrm{2}}+\mathrm{1}}+\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\mu}\right)\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{4}}\: \\ $$$${R}_{{min}} =\left(\frac{\mu}{\:\sqrt{\mathrm{2}}−\mathrm{1}}+\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\mu}\right)\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{4}}\:\:{for}\:\mu\geqslant\sqrt{\mathrm{2}}−\mathrm{1} \\ $$$${R}_{{min}} =\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}}\:\:{for}\:\mu<\sqrt{\mathrm{2}}−\mathrm{1} \\ $$$${with}\:\mu=\frac{{b}}{{a}}\leqslant\mathrm{1} \\ $$

Answered by ajfour last updated on 22/Jan/22

P(acos θ,bsin θ) condider line y−((asin θ)/2)=−(((acos θ+a)/(asin θ)))(x−((a−acos θ)/2)) And line y+(b/2)=(a/b)(x+(a/2)) subteacting (b/2)+((asin θ)/2)=((a/b)+((1+cos θ)/(sin θ)))x +(a^2 /(2b))−((asin θ)/2) ⇒ x=((((a^2 +b^2 )/b)+asin θ)/(2((a/b)+((1+cos θ)/(sin θ))))) y+b=((a^2 +b^2 )/(2b))+(a/b){((((a^2 +b^2 )/b)+asin θ)/(2((a/b)+((1+cos θ)/(sin θ)))))} x^2 +(y+b)^2 =R^2 R^2 ={((((a^2 +b^2 )/b)+asin θ)/(2((a/b)+((1+cos θ)/(sin θ)))))}^2 + +[((a^2 +b^2 )/(2b))+(a/b){((((a^2 +b^2 )/b)+asin θ)/(2((a/b)+((1+cos θ)/(sin θ)))))}]^2 R^2 =(((((a^2 +b^2 )/b)+asin θ)^2 +[4(((a^2 +b^2 )/(2b)))((a/b)+((1+cos θ)/(sin θ)))^2 +(a/b)(((a^2 +b^2 )/b)+asin θ)^2 ]^2 )/(4((a/b)+((1+cos θ)/(sin θ)))^2 )) let for example a=2, b=1 R^2 =(((5+2sin θ)^2 +[10(2+((1+cos θ)/(sin θ)))^2 +2(5+2sin θ)^2 ]^2 )/(4(2+((1+cos θ)/(sin θ)))^2 )) .....

$${P}\left({a}\mathrm{cos}\:\theta,{b}\mathrm{sin}\:\theta\right) \\ $$$${condider}\:\:{line}\:\: \\ $$$${y}−\frac{{a}\mathrm{sin}\:\theta}{\mathrm{2}}=−\left(\frac{{a}\mathrm{cos}\:\theta+{a}}{{a}\mathrm{sin}\:\theta}\right)\left({x}−\frac{{a}−{a}\mathrm{cos}\:\theta}{\mathrm{2}}\right) \\ $$$${And}\:{line} \\ $$$${y}+\frac{{b}}{\mathrm{2}}=\frac{{a}}{{b}}\left({x}+\frac{{a}}{\mathrm{2}}\right) \\ $$$${subteacting} \\ $$$$\frac{{b}}{\mathrm{2}}+\frac{{a}\mathrm{sin}\:\theta}{\mathrm{2}}=\left(\frac{{a}}{{b}}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right){x} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\frac{{a}^{\mathrm{2}} }{\mathrm{2}{b}}−\frac{{a}\mathrm{sin}\:\theta}{\mathrm{2}} \\ $$$$\Rightarrow\:\:{x}=\frac{\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{{b}}+{a}\mathrm{sin}\:\theta}{\mathrm{2}\left(\frac{{a}}{{b}}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right)} \\ $$$${y}+{b}=\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{\mathrm{2}{b}}+\frac{{a}}{{b}}\left\{\frac{\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{{b}}+{a}\mathrm{sin}\:\theta}{\mathrm{2}\left(\frac{{a}}{{b}}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right)}\right\} \\ $$$${x}^{\mathrm{2}} +\left({y}+{b}\right)^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$${R}^{\mathrm{2}} =\left\{\frac{\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{{b}}+{a}\mathrm{sin}\:\theta}{\mathrm{2}\left(\frac{{a}}{{b}}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right)}\right\}^{\mathrm{2}} + \\ $$$$\:\:\:\:\:\:+\left[\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{\mathrm{2}{b}}+\frac{{a}}{{b}}\left\{\frac{\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{{b}}+{a}\mathrm{sin}\:\theta}{\mathrm{2}\left(\frac{{a}}{{b}}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right)}\right\}\right]^{\mathrm{2}} \\ $$$${R}^{\mathrm{2}} =\frac{\left(\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{{b}}+{a}\mathrm{sin}\:\theta\right)^{\mathrm{2}} +\left[\mathrm{4}\left(\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{\mathrm{2}{b}}\right)\left(\frac{{a}}{{b}}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right)^{\mathrm{2}} +\frac{{a}}{{b}}\left(\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{{b}}+{a}\mathrm{sin}\:\theta\right)^{\mathrm{2}} \right]^{\mathrm{2}} }{\mathrm{4}\left(\frac{{a}}{{b}}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right)^{\mathrm{2}} } \\ $$$${let}\:\:{for}\:{example}\:\:{a}=\mathrm{2},\:{b}=\mathrm{1} \\ $$$${R}^{\mathrm{2}} =\frac{\left(\mathrm{5}+\mathrm{2sin}\:\theta\right)^{\mathrm{2}} +\left[\mathrm{10}\left(\mathrm{2}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right)^{\mathrm{2}} +\mathrm{2}\left(\mathrm{5}+\mathrm{2sin}\:\theta\right)^{\mathrm{2}} \right]^{\mathrm{2}} }{\mathrm{4}\left(\mathrm{2}+\frac{\mathrm{1}+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\right)^{\mathrm{2}} } \\ $$$$….. \\ $$$$ \\ $$

Commented by Tawa11 last updated on 22/Jan/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$

Answered by ajfour last updated on 23/Jan/22

Let left end be origin O. slope p=((bsin θ)/(a+acos θ)) p^2 +1=((a^2 (1+cos θ)^2 +b^2 sin^2 θ)/(a^2 (1+cos θ)^2 )) slope q=((b+bsin θ)/(acos θ)) tan φ=tan (tan^(−1) q−tan^(−1) p) R is maximum if φ=φ_(max) . tan φ=((q−p)/(1+pq)) ((d(tan φ))/dθ)=0 ⇒ (((1+pq)((dq/dθ)−(dp/dθ))−(q−p)[q((dp/dθ))+p((dq/dθ))])/((1+pq)^2 )) =0 (1+p^2 )((dq/dθ))=(1+q^2 )((dp/dθ)) (1+p^2 )[((abcos^2 θ+asin θ(b+bsin θ))/(a^2 cos^2 θ))] =(1+q^2 )[(((1+q^2 )[abcos θ(1+cos θ)+absin^2 θ])/((a+acos θ)^2 )) ⇒ (1+p^2 )(((1+sin θ)/(cos^2 θ)))=(1+q^2 )((1/(1+cos θ))) ....

$${Let}\:{left}\:{end}\:{be}\:{origin}\:{O}. \\ $$$${slope}\:\:{p}=\frac{{b}\mathrm{sin}\:\theta}{{a}+{a}\mathrm{cos}\:\theta} \\ $$$${p}^{\mathrm{2}} +\mathrm{1}=\frac{{a}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{cos}\:\theta\right)^{\mathrm{2}} +{b}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \theta}{{a}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{cos}\:\theta\right)^{\mathrm{2}} } \\ $$$$\:\:{slope}\:\:{q}=\frac{{b}+{b}\mathrm{sin}\:\theta}{{a}\mathrm{cos}\:\theta} \\ $$$$\mathrm{tan}\:\phi=\mathrm{tan}\:\left(\mathrm{tan}^{−\mathrm{1}} {q}−\mathrm{tan}^{−\mathrm{1}} {p}\right) \\ $$$${R}\:{is}\:{maximum}\:{if}\:\phi=\phi_{{max}} . \\ $$$$\:\:\mathrm{tan}\:\phi=\frac{{q}−{p}}{\mathrm{1}+{pq}} \\ $$$$\:\:\frac{{d}\left(\mathrm{tan}\:\phi\right)}{{d}\theta}=\mathrm{0} \\ $$$$\Rightarrow\:\:\:\frac{\left(\mathrm{1}+{pq}\right)\left(\frac{{dq}}{{d}\theta}−\frac{{dp}}{{d}\theta}\right)−\left({q}−{p}\right)\left[{q}\left(\frac{{dp}}{{d}\theta}\right)+{p}\left(\frac{{dq}}{{d}\theta}\right)\right]}{\left(\mathrm{1}+{pq}\right)^{\mathrm{2}} } \\ $$$$\:\:=\mathrm{0} \\ $$$$\left(\mathrm{1}+{p}^{\mathrm{2}} \right)\left(\frac{{dq}}{{d}\theta}\right)=\left(\mathrm{1}+{q}^{\mathrm{2}} \right)\left(\frac{{dp}}{{d}\theta}\right) \\ $$$$\left(\mathrm{1}+{p}^{\mathrm{2}} \right)\left[\frac{{ab}\mathrm{cos}\:^{\mathrm{2}} \theta+{a}\mathrm{sin}\:\theta\left({b}+{b}\mathrm{sin}\:\theta\right)}{{a}^{\mathrm{2}} \mathrm{cos}\:^{\mathrm{2}} \theta}\right] \\ $$$$\:\:\:\:=\left(\mathrm{1}+{q}^{\mathrm{2}} \right)\left[\frac{\left(\mathrm{1}+{q}^{\mathrm{2}} \right)\left[{ab}\mathrm{cos}\:\theta\left(\mathrm{1}+\mathrm{cos}\:\theta\right)+{ab}\mathrm{sin}\:^{\mathrm{2}} \theta\right]}{\left({a}+{a}\mathrm{cos}\:\theta\right)^{\mathrm{2}} }\right. \\ $$$$\Rightarrow\:\:\left(\mathrm{1}+{p}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}+\mathrm{sin}\:\theta}{\mathrm{cos}\:^{\mathrm{2}} \theta}\right)=\left(\mathrm{1}+{q}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}}{\mathrm{1}+\mathrm{cos}\:\theta}\right) \\ $$$$…. \\ $$

Answered by mr W last updated on 23/Jan/22

Commented by mr W last updated on 24/Jan/22

let μ=(b/a)≤1 A(−a,0) B(0,−b) say P(a cos θ, b sin θ) AP=(√(a^2 (1+cos θ)^2 +b^2 sin^2 θ)) ⇒AP=a(√((1+cos θ)^2 +μ^2 sin^2 θ)) BP=(√(a^2 cos^2 θ+b^2 (1+sin θ)^2 )) ⇒BP=a(√(cos^2 θ+μ^2 (1+sin θ)^2 )) AB=(√(a^2 +b^2 ))=a(√(1+μ^2 )) eqn. of AB: (x/a)+(y/b)+1=0 distance from P to AB: d=((∣cos θ+sin θ+1∣)/( (√((1/a^2 )+(1/b^2 )))))=((a^2 μ∣cos θ+sin θ+1∣)/( (√(a^2 +b^2 )))) area of ΔABP: Δ=(d(√(a^2 +b^2 ))/2)=((a^2 μ(cos θ+sin θ+1))/2) Δ=((AP×BP sin φ)/2) Δ=((a^2 sin φ(√([(1+cos θ)^2 +μ^2 sin^2 θ][cos^2 θ+μ^2 (1+sin θ)^2 ])))/2) ((a^2 μ(cos θ+sin θ+1))/2)=((a^2 sin φ(√([(1+cos θ)^2 +μ^2 sin^2 θ][cos^2 θ+μ^2 (1+sin θ)^2 ])))/2) sin φ=((μ(cos θ+sin θ+1))/( (√([(1+cos θ)^2 +μ^2 sin^2 θ][cos^2 θ+μ^2 (1+sin θ)^2 ])))) R=radius of circumcircle of ΔABP AB=2R sin φ R=((AB)/(2 sin φ)) R=((√(a^2 +b^2 ))/(2μ))×((√([(1+cos θ)^2 +μ^2 sin^2 θ][cos^2 θ+μ^2 (1+sin θ)^2 ]))/(cos θ+sin θ+1)) R=(((1−μ^2 )(√(a^2 +b^2 )))/(2μ))×((√((1+cos θ)(1+sin θ)(((1+μ^2 )/(1−μ^2 ))+cos θ)(((1+μ^2 )/(1−μ^2 ))−sin θ)))/(cos θ+sin θ+1)) let λ=((1+μ^2 )/(1−μ^2 )) R=(((1−μ^2 )(√(a^2 +b^2 )))/(2μ))×((√((1+cos θ)(1+sin θ)(λ+cos θ)(λ−sin θ)))/(cos θ+sin θ+1)) R=(((1−μ^2 )(√(a^2 +b^2 )))/(2μ))×(√(Φ(θ))) Φ(θ)=(((1+cos θ)(1+sin θ)(λ+cos θ)(λ−sin θ))/((cos θ+sin θ+1)^2 )) ln Φ(θ)=ln (1+cos θ)+ln (1+sin θ)+ln (λ+cos θ)+ln (λ−sin θ)−2 ln (cos θ+sin θ+1) ((Φ′(θ))/(Φ(θ)))=((−sin θ)/(1+cos θ))+((cos θ)/(1+sin θ))+((−sin θ)/(λ+cos θ))+((−cos θ)/(λ−sin θ))−((2(−sin θ+cos θ))/(cos θ+sin θ+1)) ((Φ′(θ))/(Φ(θ)))=(cos θ−sin θ)[(((1+cos θ+sin θ)^2 −2(1+cos θ)(1+sin θ))/((1+cos θ)(1+sin θ)))]−(((sin θ+cos θ)(λ+cos θ−sin θ))/((λ+cos θ)(λ−sin θ))) ((Φ′(θ))/(Φ(θ)))=−(((sin θ+cos θ)(λ+cos θ−sin θ))/((λ+cos θ)(λ−sin θ))) Φ′(θ)=0 for R_(max) or R_(min) . (sin θ+cos θ)(λ+cos θ−sin θ)=0 sin θ+cos θ=0 tan θ=−1 ⇒θ=−(π/4) or ((3π)/4) λ+cos θ−sin θ=0 sin θ−cos θ=λ (√2) sin (θ−(π/4))=λ sin (θ−(π/4))=(λ/( (√2))) valid only when (λ/( (√2)))≤1, i.e. λ=((1+μ^2 )/(1−μ^2 ))≤(√2), μ^2 ≤(((√2)−1)/( (√2)+1))=((√2)−1)^2 ⇒μ≤(√2)−1 θ−(π/4)=sin^(−1) (λ/( (√2))) or π−sin^(−1) (λ/( (√2))) ⇒θ=sin^(−1) (λ/( (√2)))+(π/4) or ((5π)/4)−sin^(−1) (λ/( (√2))) R_(max) is at θ=−(π/4): R_(max) =(1/( (√2)))(λ+(1/( (√2))))(((1−μ^2 )(√(a^2 +b^2 )))/(2μ)) R_(max) =((((√2)+1)/( μ))+(μ/( (√2)+1)))((√(a^2 +b^2 ))/4) R_(min) is at θ=((3π)/4), if μ≥(√2)−1: R_(min) =((((√2)−1)/( μ))+(μ/( (√2)−1)))((√(a^2 +b^2 ))/4) R_(min) is at θ=sin^(−1) (λ/( (√2)))+(π/4) or ((5π)/4)−sin^(−1) (λ/( (√2))), if μ≤(√2)−1: R_(min) =((√(a^2 +b^2 ))/2) how to find the circumcenter: eqn. of bisector of AP: y=((b sin θ)/2)−((cos θ+1)/(μ sin θ)) (x−((a(cos θ−1))/2)) eqn. of bisector of BP: y=((b(sin θ−1))/2)−((cos θ)/(μ(1+sin θ)))(x−((a cos θ)/2)) circumcenter C(x_C , y_C ) ((μ(sin θ−1))/2)−((cos θ)/(μ(1+sin θ)))((x_C /a)−((cos θ)/2))=((μ sin θ)/2)−((cos θ+1)/(μ sin θ)) ((x_C /a)−((cos θ−1)/2)) −(μ^2 /2)=((cos θ)/((1+sin θ)))((x_C /a)−((cos θ)/2))−((cos θ+1)/( sin θ)) ((x_C /a)−((cos θ−1)/2)) ((1−μ^2 )/2)=(((cos θ)/(1+sin θ))−((cos θ+1)/( sin θ)))(x_C /a) ⇒(x_C /a)=−(((1−μ^2 )(1+sin θ) sin θ)/(2(1+sin θ+cos θ))) (y_C /a)=((μ(sin θ−1))/2)−((cos θ)/(μ(1+sin θ)))((x_C /a)−((cos θ)/2)) (y_C /a)=(1/(2μ)){((−μ^2 (1−sin θ)(1+sin θ+cos θ)+cos θ−μ^2 sin θ cos θ+cos^2 θ)/((1+sin θ+cos θ)))} ⇒(y_C /a)=(((1−μ^2 )(1+cos θ) cos θ)/(2μ(1+sin θ+cos θ)))

$${let}\:\mu=\frac{{b}}{{a}}\leqslant\mathrm{1} \\ $$$${A}\left(−{a},\mathrm{0}\right) \\ $$$${B}\left(\mathrm{0},−{b}\right) \\ $$$${say}\:{P}\left({a}\:\mathrm{cos}\:\theta,\:{b}\:\mathrm{sin}\:\theta\right) \\ $$$${AP}=\sqrt{{a}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{cos}\:\theta\right)^{\mathrm{2}} +{b}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$$\Rightarrow{AP}={a}\sqrt{\left(\mathrm{1}+\mathrm{cos}\:\theta\right)^{\mathrm{2}} +\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$${BP}=\sqrt{{a}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\theta+{b}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{sin}\:\theta\right)^{\mathrm{2}} } \\ $$$$\Rightarrow{BP}={a}\sqrt{\mathrm{cos}^{\mathrm{2}} \:\theta+\mu^{\mathrm{2}} \left(\mathrm{1}+\mathrm{sin}\:\theta\right)^{\mathrm{2}} } \\ $$$$ \\ $$$${AB}=\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }={a}\sqrt{\mathrm{1}+\mu^{\mathrm{2}} } \\ $$$${eqn}.\:{of}\:{AB}: \\ $$$$\frac{{x}}{{a}}+\frac{{y}}{{b}}+\mathrm{1}=\mathrm{0} \\ $$$${distance}\:{from}\:{P}\:{to}\:{AB}: \\ $$$${d}=\frac{\mid\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}\mid}{\:\sqrt{\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }}}=\frac{{a}^{\mathrm{2}} \mu\mid\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}\mid}{\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }} \\ $$$${area}\:{of}\:\Delta{ABP}: \\ $$$$\Delta=\frac{{d}\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}}=\frac{{a}^{\mathrm{2}} \mu\left(\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}\right)}{\mathrm{2}} \\ $$$$\Delta=\frac{{AP}×{BP}\:\mathrm{sin}\:\phi}{\mathrm{2}} \\ $$$$\Delta=\frac{{a}^{\mathrm{2}} \:\mathrm{sin}\:\phi\sqrt{\left[\left(\mathrm{1}+\mathrm{cos}\:\theta\right)^{\mathrm{2}} +\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta\right]\left[\mathrm{cos}^{\mathrm{2}} \:\theta+\mu^{\mathrm{2}} \left(\mathrm{1}+\mathrm{sin}\:\theta\right)^{\mathrm{2}} \right]}}{\mathrm{2}} \\ $$$$\frac{{a}^{\mathrm{2}} \mu\left(\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}\right)}{\mathrm{2}}=\frac{{a}^{\mathrm{2}} \:\mathrm{sin}\:\phi\sqrt{\left[\left(\mathrm{1}+\mathrm{cos}\:\theta\right)^{\mathrm{2}} +\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta\right]\left[\mathrm{cos}^{\mathrm{2}} \:\theta+\mu^{\mathrm{2}} \left(\mathrm{1}+\mathrm{sin}\:\theta\right)^{\mathrm{2}} \right]}}{\mathrm{2}} \\ $$$$\mathrm{sin}\:\phi=\frac{\mu\left(\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}\right)}{\:\sqrt{\left[\left(\mathrm{1}+\mathrm{cos}\:\theta\right)^{\mathrm{2}} +\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta\right]\left[\mathrm{cos}^{\mathrm{2}} \:\theta+\mu^{\mathrm{2}} \left(\mathrm{1}+\mathrm{sin}\:\theta\right)^{\mathrm{2}} \right]}} \\ $$$$ \\ $$$${R}={radius}\:{of}\:{circumcircle}\:{of}\:\Delta{ABP} \\ $$$${AB}=\mathrm{2}{R}\:\mathrm{sin}\:\phi \\ $$$${R}=\frac{{AB}}{\mathrm{2}\:\mathrm{sin}\:\phi} \\ $$$${R}=\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}\mu}×\frac{\sqrt{\left[\left(\mathrm{1}+\mathrm{cos}\:\theta\right)^{\mathrm{2}} +\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta\right]\left[\mathrm{cos}^{\mathrm{2}} \:\theta+\mu^{\mathrm{2}} \left(\mathrm{1}+\mathrm{sin}\:\theta\right)^{\mathrm{2}} \right]}}{\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}} \\ $$$${R}=\frac{\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}\mu}×\frac{\sqrt{\left(\mathrm{1}+\mathrm{cos}\:\theta\right)\left(\mathrm{1}+\mathrm{sin}\:\theta\right)\left(\frac{\mathrm{1}+\mu^{\mathrm{2}} }{\mathrm{1}−\mu^{\mathrm{2}} }+\mathrm{cos}\:\theta\right)\left(\frac{\mathrm{1}+\mu^{\mathrm{2}} }{\mathrm{1}−\mu^{\mathrm{2}} }−\mathrm{sin}\:\theta\right)}}{\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}} \\ $$$${let}\:\lambda=\frac{\mathrm{1}+\mu^{\mathrm{2}} }{\mathrm{1}−\mu^{\mathrm{2}} } \\ $$$${R}=\frac{\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}\mu}×\frac{\sqrt{\left(\mathrm{1}+\mathrm{cos}\:\theta\right)\left(\mathrm{1}+\mathrm{sin}\:\theta\right)\left(\lambda+\mathrm{cos}\:\theta\right)\left(\lambda−\mathrm{sin}\:\theta\right)}}{\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}} \\ $$$${R}=\frac{\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}\mu}×\sqrt{\Phi\left(\theta\right)} \\ $$$$\Phi\left(\theta\right)=\frac{\left(\mathrm{1}+\mathrm{cos}\:\theta\right)\left(\mathrm{1}+\mathrm{sin}\:\theta\right)\left(\lambda+\mathrm{cos}\:\theta\right)\left(\lambda−\mathrm{sin}\:\theta\right)}{\left(\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\mathrm{ln}\:\Phi\left(\theta\right)=\mathrm{ln}\:\left(\mathrm{1}+\mathrm{cos}\:\theta\right)+\mathrm{ln}\:\left(\mathrm{1}+\mathrm{sin}\:\theta\right)+\mathrm{ln}\:\left(\lambda+\mathrm{cos}\:\theta\right)+\mathrm{ln}\:\left(\lambda−\mathrm{sin}\:\theta\right)−\mathrm{2}\:\mathrm{ln}\:\left(\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}\right) \\ $$$$\frac{\Phi'\left(\theta\right)}{\Phi\left(\theta\right)}=\frac{−\mathrm{sin}\:\theta}{\mathrm{1}+\mathrm{cos}\:\theta}+\frac{\mathrm{cos}\:\theta}{\mathrm{1}+\mathrm{sin}\:\theta}+\frac{−\mathrm{sin}\:\theta}{\lambda+\mathrm{cos}\:\theta}+\frac{−\mathrm{cos}\:\theta}{\lambda−\mathrm{sin}\:\theta}−\frac{\mathrm{2}\left(−\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)}{\mathrm{cos}\:\theta+\mathrm{sin}\:\theta+\mathrm{1}} \\ $$$$\frac{\Phi'\left(\theta\right)}{\Phi\left(\theta\right)}=\left(\mathrm{cos}\:\theta−\mathrm{sin}\:\theta\right)\left[\frac{\left(\mathrm{1}+\mathrm{cos}\:\theta+\mathrm{sin}\:\theta\right)^{\mathrm{2}} −\mathrm{2}\left(\mathrm{1}+\mathrm{cos}\:\theta\right)\left(\mathrm{1}+\mathrm{sin}\:\theta\right)}{\left(\mathrm{1}+\mathrm{cos}\:\theta\right)\left(\mathrm{1}+\mathrm{sin}\:\theta\right)}\right]−\frac{\left(\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)\left(\lambda+\mathrm{cos}\:\theta−\mathrm{sin}\:\theta\right)}{\left(\lambda+\mathrm{cos}\:\theta\right)\left(\lambda−\mathrm{sin}\:\theta\right)} \\ $$$$\frac{\Phi'\left(\theta\right)}{\Phi\left(\theta\right)}=−\frac{\left(\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)\left(\lambda+\mathrm{cos}\:\theta−\mathrm{sin}\:\theta\right)}{\left(\lambda+\mathrm{cos}\:\theta\right)\left(\lambda−\mathrm{sin}\:\theta\right)} \\ $$$$\Phi'\left(\theta\right)=\mathrm{0}\:{for}\:{R}_{{max}} \:{or}\:{R}_{{min}} . \\ $$$$\left(\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)\left(\lambda+\mathrm{cos}\:\theta−\mathrm{sin}\:\theta\right)=\mathrm{0} \\ $$$$\mathrm{sin}\:\theta+\mathrm{cos}\:\theta=\mathrm{0} \\ $$$$\mathrm{tan}\:\theta=−\mathrm{1} \\ $$$$\Rightarrow\theta=−\frac{\pi}{\mathrm{4}}\:{or}\:\frac{\mathrm{3}\pi}{\mathrm{4}} \\ $$$$ \\ $$$$\lambda+\mathrm{cos}\:\theta−\mathrm{sin}\:\theta=\mathrm{0} \\ $$$$\mathrm{sin}\:\theta−\mathrm{cos}\:\theta=\lambda \\ $$$$\sqrt{\mathrm{2}}\:\mathrm{sin}\:\left(\theta−\frac{\pi}{\mathrm{4}}\right)=\lambda \\ $$$$\mathrm{sin}\:\left(\theta−\frac{\pi}{\mathrm{4}}\right)=\frac{\lambda}{\:\sqrt{\mathrm{2}}} \\ $$$${valid}\:{only}\:{when}\:\frac{\lambda}{\:\sqrt{\mathrm{2}}}\leqslant\mathrm{1},\: \\ $$$${i}.{e}.\:\lambda=\frac{\mathrm{1}+\mu^{\mathrm{2}} }{\mathrm{1}−\mu^{\mathrm{2}} }\leqslant\sqrt{\mathrm{2}},\: \\ $$$$\mu^{\mathrm{2}} \leqslant\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\:\sqrt{\mathrm{2}}+\mathrm{1}}=\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} \:\Rightarrow\mu\leqslant\sqrt{\mathrm{2}}−\mathrm{1} \\ $$$$\theta−\frac{\pi}{\mathrm{4}}=\mathrm{sin}^{−\mathrm{1}} \frac{\lambda}{\:\sqrt{\mathrm{2}}}\:{or}\:\pi−\mathrm{sin}^{−\mathrm{1}} \frac{\lambda}{\:\sqrt{\mathrm{2}}} \\ $$$$\Rightarrow\theta=\mathrm{sin}^{−\mathrm{1}} \frac{\lambda}{\:\sqrt{\mathrm{2}}}+\frac{\pi}{\mathrm{4}}\:{or}\:\frac{\mathrm{5}\pi}{\mathrm{4}}−\mathrm{sin}^{−\mathrm{1}} \frac{\lambda}{\:\sqrt{\mathrm{2}}} \\ $$$$ \\ $$$${R}_{{max}} \:{is}\:{at}\:\theta=−\frac{\pi}{\mathrm{4}}: \\ $$$${R}_{{max}} =\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\left(\lambda+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right)\frac{\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}\mu} \\ $$$${R}_{{max}} =\left(\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\:\mu}+\frac{\mu}{\:\sqrt{\mathrm{2}}+\mathrm{1}}\right)\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{4}} \\ $$$$ \\ $$$${R}_{{min}} \:{is}\:{at}\:\theta=\frac{\mathrm{3}\pi}{\mathrm{4}},\:{if}\:\mu\geqslant\sqrt{\mathrm{2}}−\mathrm{1}: \\ $$$${R}_{{min}} =\left(\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\:\mu}+\frac{\mu}{\:\sqrt{\mathrm{2}}−\mathrm{1}}\right)\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{4}} \\ $$$${R}_{{min}} \:{is}\:{at}\:\theta=\mathrm{sin}^{−\mathrm{1}} \frac{\lambda}{\:\sqrt{\mathrm{2}}}+\frac{\pi}{\mathrm{4}}\:{or}\:\frac{\mathrm{5}\pi}{\mathrm{4}}−\mathrm{sin}^{−\mathrm{1}} \frac{\lambda}{\:\sqrt{\mathrm{2}}},\:{if}\:\mu\leqslant\sqrt{\mathrm{2}}−\mathrm{1}: \\ $$$${R}_{{min}} =\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{\mathrm{2}} \\ $$$$ \\ $$$${how}\:{to}\:{find}\:{the}\:{circumcenter}: \\ $$$${eqn}.\:{of}\:\:{bisector}\:{of}\:{AP}: \\ $$$${y}=\frac{{b}\:\mathrm{sin}\:\theta}{\mathrm{2}}−\frac{\mathrm{cos}\:\theta+\mathrm{1}}{\mu\:\mathrm{sin}\:\theta}\:\left({x}−\frac{{a}\left(\mathrm{cos}\:\theta−\mathrm{1}\right)}{\mathrm{2}}\right) \\ $$$${eqn}.\:{of}\:{bisector}\:{of}\:{BP}: \\ $$$${y}=\frac{{b}\left(\mathrm{sin}\:\theta−\mathrm{1}\right)}{\mathrm{2}}−\frac{\mathrm{cos}\:\theta}{\mu\left(\mathrm{1}+\mathrm{sin}\:\theta\right)}\left({x}−\frac{{a}\:\mathrm{cos}\:\theta}{\mathrm{2}}\right) \\ $$$${circumcenter}\:{C}\left({x}_{{C}} ,\:{y}_{{C}} \right) \\ $$$$\frac{\mu\left(\mathrm{sin}\:\theta−\mathrm{1}\right)}{\mathrm{2}}−\frac{\mathrm{cos}\:\theta}{\mu\left(\mathrm{1}+\mathrm{sin}\:\theta\right)}\left(\frac{{x}_{{C}} }{{a}}−\frac{\mathrm{cos}\:\theta}{\mathrm{2}}\right)=\frac{\mu\:\mathrm{sin}\:\theta}{\mathrm{2}}−\frac{\mathrm{cos}\:\theta+\mathrm{1}}{\mu\:\mathrm{sin}\:\theta}\:\left(\frac{{x}_{{C}} }{{a}}−\frac{\mathrm{cos}\:\theta−\mathrm{1}}{\mathrm{2}}\right) \\ $$$$−\frac{\mu^{\mathrm{2}} }{\mathrm{2}}=\frac{\mathrm{cos}\:\theta}{\left(\mathrm{1}+\mathrm{sin}\:\theta\right)}\left(\frac{{x}_{{C}} }{{a}}−\frac{\mathrm{cos}\:\theta}{\mathrm{2}}\right)−\frac{\mathrm{cos}\:\theta+\mathrm{1}}{\:\mathrm{sin}\:\theta}\:\left(\frac{{x}_{{C}} }{{a}}−\frac{\mathrm{cos}\:\theta−\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\frac{\mathrm{1}−\mu^{\mathrm{2}} }{\mathrm{2}}=\left(\frac{\mathrm{cos}\:\theta}{\mathrm{1}+\mathrm{sin}\:\theta}−\frac{\mathrm{cos}\:\theta+\mathrm{1}}{\:\mathrm{sin}\:\theta}\right)\frac{{x}_{{C}} }{{a}} \\ $$$$\Rightarrow\frac{{x}_{{C}} }{{a}}=−\frac{\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{sin}\:\theta\right)\:\mathrm{sin}\:\theta}{\mathrm{2}\left(\mathrm{1}+\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)} \\ $$$$\frac{{y}_{{C}} }{{a}}=\frac{\mu\left(\mathrm{sin}\:\theta−\mathrm{1}\right)}{\mathrm{2}}−\frac{\mathrm{cos}\:\theta}{\mu\left(\mathrm{1}+\mathrm{sin}\:\theta\right)}\left(\frac{{x}_{{C}} }{{a}}−\frac{\mathrm{cos}\:\theta}{\mathrm{2}}\right) \\ $$$$\frac{{y}_{{C}} }{{a}}=\frac{\mathrm{1}}{\mathrm{2}\mu}\left\{\frac{−\mu^{\mathrm{2}} \left(\mathrm{1}−\mathrm{sin}\:\theta\right)\left(\mathrm{1}+\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)+\mathrm{cos}\:\theta−\mu^{\mathrm{2}} \:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta+\mathrm{cos}^{\mathrm{2}} \:\theta}{\left(\mathrm{1}+\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)}\right\} \\ $$$$\Rightarrow\frac{{y}_{{C}} }{{a}}=\frac{\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{cos}\:\theta\right)\:\mathrm{cos}\:\theta}{\mathrm{2}\mu\left(\mathrm{1}+\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)} \\ $$

Commented by mr W last updated on 23/Jan/22