Question Number 165296 by ajfour last updated on 28/Jan/22

Commented by ajfour last updated on 28/Jan/22

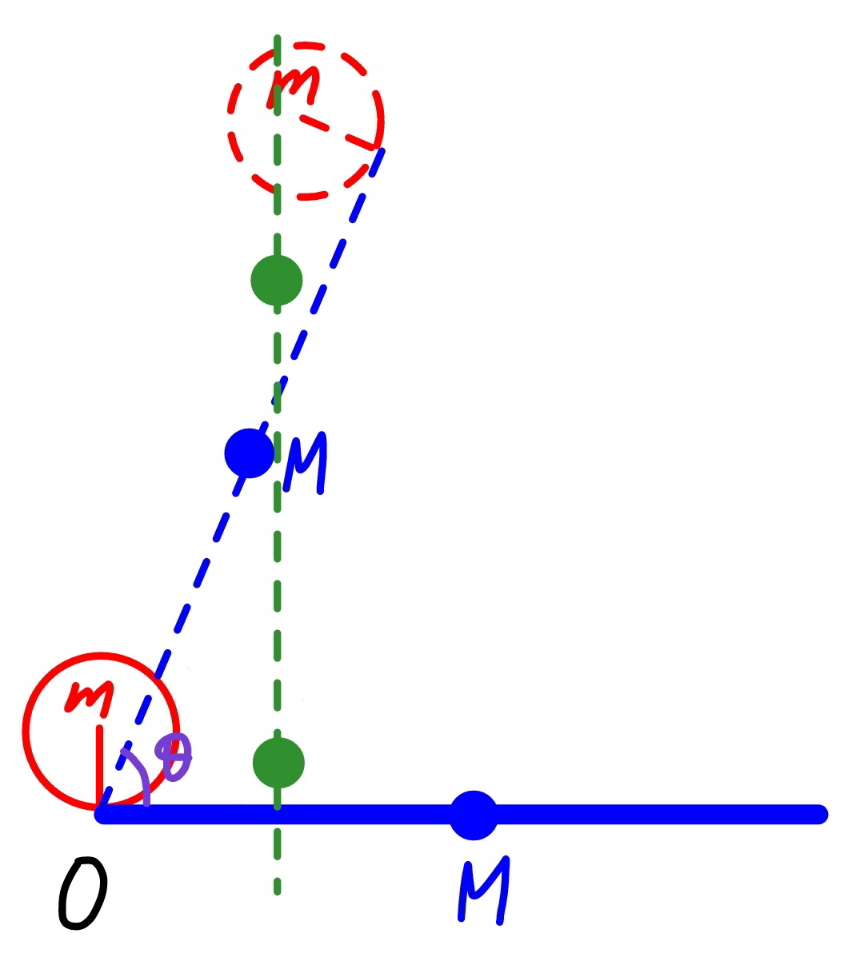
$${If}\:{when}\:{the}\:{disc}\:{rolls}\:{down}\:{and}\:{is} \\ $$$$\:{about}\:{to}\:{to}\:{hit}\:{the}\:{pivot},\:{the}\:{rod} \\ $$$${gets}\:{horizontal},\:{find}\:{initial}\:{angle} \\ $$$$\theta\:{at}\:{which}\:{system}\:{is}\:{released}. \\ $$$$\left({assume}\:{pure}\:{rolling}\:{throughout}\right) \\ $$
Answered by TheSupreme last updated on 29/Jan/22
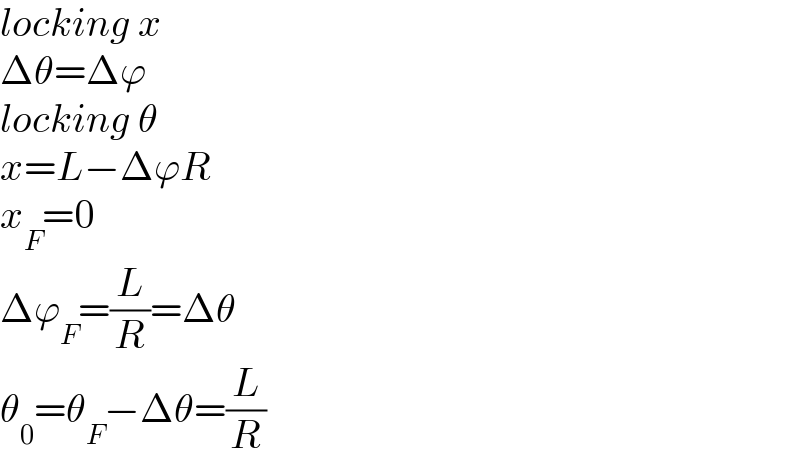
$${locking}\:{x} \\ $$$$\Delta\theta=\Delta\varphi \\ $$$${locking}\:\theta \\ $$$${x}={L}−\Delta\varphi{R} \\ $$$${x}_{{F}} =\mathrm{0} \\ $$$$\Delta\varphi_{{F}} =\frac{{L}}{{R}}=\Delta\theta \\ $$$$\theta_{\mathrm{0}} =\theta_{{F}} −\Delta\theta=\frac{{L}}{{R}} \\ $$
Answered by mr W last updated on 30/Jan/22
Commented by mr W last updated on 29/Jan/22
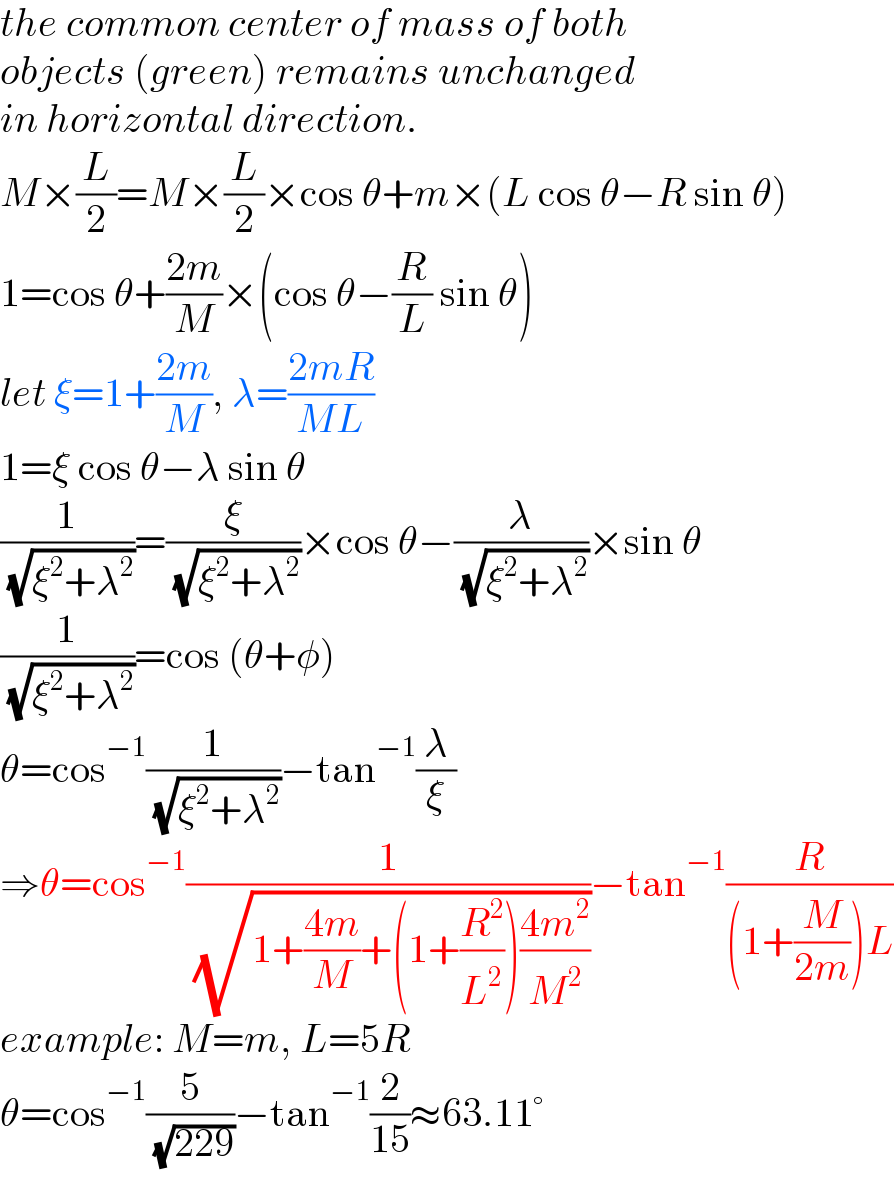
$${the}\:{common}\:{center}\:{of}\:{mass}\:{of}\:{both} \\ $$$${objects}\:\left({green}\right)\:{remains}\:{unchanged} \\ $$$${in}\:{horizontal}\:{direction}. \\ $$$${M}×\frac{{L}}{\mathrm{2}}={M}×\frac{{L}}{\mathrm{2}}×\mathrm{cos}\:\theta+{m}×\left({L}\:\mathrm{cos}\:\theta−{R}\:\mathrm{sin}\:\theta\right) \\ $$$$\mathrm{1}=\mathrm{cos}\:\theta+\frac{\mathrm{2}{m}}{{M}}×\left(\mathrm{cos}\:\theta−\frac{{R}}{{L}}\:\mathrm{sin}\:\theta\right) \\ $$$${let}\:\xi=\mathrm{1}+\frac{\mathrm{2}{m}}{{M}},\:\lambda=\frac{\mathrm{2}{mR}}{{ML}} \\ $$$$\mathrm{1}=\xi\:\mathrm{cos}\:\theta−\lambda\:\mathrm{sin}\:\theta \\ $$$$\frac{\mathrm{1}}{\:\sqrt{\xi^{\mathrm{2}} +\lambda^{\mathrm{2}} }}=\frac{\xi}{\:\sqrt{\xi^{\mathrm{2}} +\lambda^{\mathrm{2}} }}×\mathrm{cos}\:\theta−\frac{\lambda}{\:\sqrt{\xi^{\mathrm{2}} +\lambda^{\mathrm{2}} }}×\mathrm{sin}\:\theta \\ $$$$\frac{\mathrm{1}}{\:\sqrt{\xi^{\mathrm{2}} +\lambda^{\mathrm{2}} }}=\mathrm{cos}\:\left(\theta+\phi\right) \\ $$$$\theta=\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\:\sqrt{\xi^{\mathrm{2}} +\lambda^{\mathrm{2}} }}−\mathrm{tan}^{−\mathrm{1}} \frac{\lambda}{\xi} \\ $$$$\Rightarrow\theta=\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\frac{\mathrm{4}{m}}{{M}}+\left(\mathrm{1}+\frac{{R}^{\mathrm{2}} }{{L}^{\mathrm{2}} }\right)\frac{\mathrm{4}{m}^{\mathrm{2}} }{{M}^{\mathrm{2}} }}}−\mathrm{tan}^{−\mathrm{1}} \frac{{R}}{\left(\mathrm{1}+\frac{{M}}{\mathrm{2}{m}}\right){L}} \\ $$$${example}:\:{M}={m},\:{L}=\mathrm{5}{R} \\ $$$$\theta=\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{5}}{\:\sqrt{\mathrm{229}}}−\mathrm{tan}^{−\mathrm{1}} \frac{\mathrm{2}}{\mathrm{15}}\approx\mathrm{63}.\mathrm{11}° \\ $$
Commented by Tawa11 last updated on 29/Jan/22

$$\mathrm{Weldone}\:\mathrm{sir}. \\ $$
Commented by ajfour last updated on 29/Jan/22
$${Really}\:{greatly}\:{done},\:{fine}\:{notice} \\ $$$${Sir}.\:{Thanks}\:{immensely}! \\ $$
