Question Number 165428 by mathlove last updated on 01/Feb/22

Answered by som(math1967) last updated on 01/Feb/22
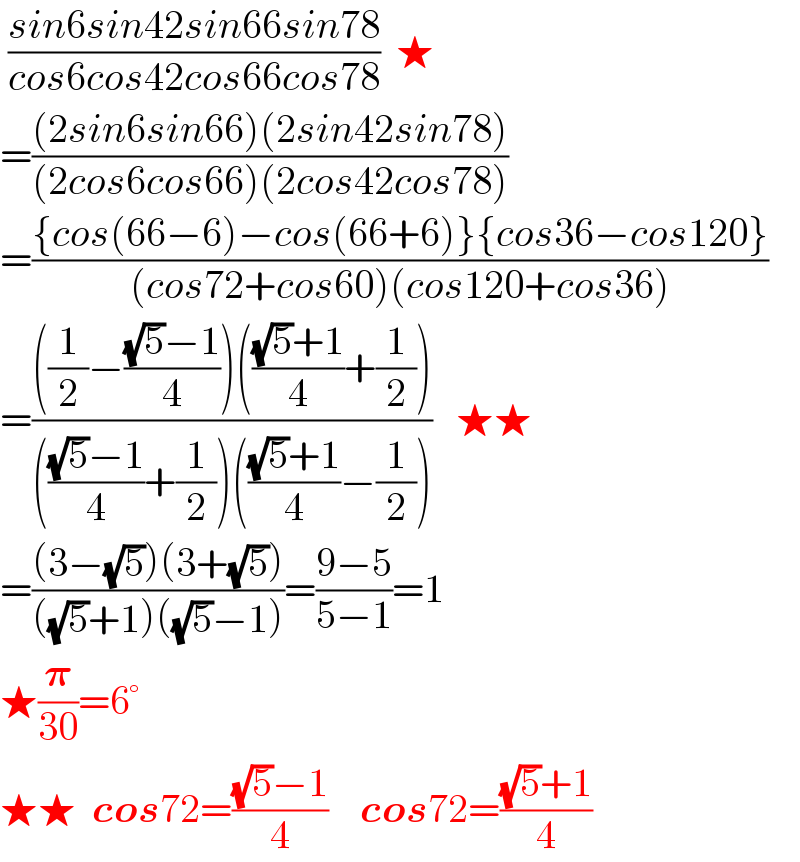
$$\:\frac{{sin}\mathrm{6}{sin}\mathrm{42}{sin}\mathrm{66}{sin}\mathrm{78}}{{cos}\mathrm{6}{cos}\mathrm{42}{cos}\mathrm{66}{cos}\mathrm{78}}\:\:\bigstar \\ $$$$=\frac{\left(\mathrm{2}{sin}\mathrm{6}{sin}\mathrm{66}\right)\left(\mathrm{2}{sin}\mathrm{42}{sin}\mathrm{78}\right)}{\left(\mathrm{2}{cos}\mathrm{6}{cos}\mathrm{66}\right)\left(\mathrm{2}{cos}\mathrm{42}{cos}\mathrm{78}\right)} \\ $$$$=\frac{\left\{{cos}\left(\mathrm{66}−\mathrm{6}\right)−{cos}\left(\mathrm{66}+\mathrm{6}\right)\right\}\left\{{cos}\mathrm{36}−{cos}\mathrm{120}\right\}}{\left({cos}\mathrm{72}+{cos}\mathrm{60}\right)\left({cos}\mathrm{120}+{cos}\mathrm{36}\right)} \\ $$$$=\frac{\left(\frac{\mathrm{1}}{\mathrm{2}}−\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\right)\left(\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}\right)}{\left(\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}\right)\left(\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}}\right)}\:\:\:\bigstar\bigstar \\ $$$$=\frac{\left(\mathrm{3}−\sqrt{\mathrm{5}}\right)\left(\mathrm{3}+\sqrt{\mathrm{5}}\right)}{\left(\sqrt{\mathrm{5}}+\mathrm{1}\right)\left(\sqrt{\mathrm{5}}−\mathrm{1}\right)}=\frac{\mathrm{9}−\mathrm{5}}{\mathrm{5}−\mathrm{1}}=\mathrm{1} \\ $$$$\bigstar\frac{\boldsymbol{\pi}}{\mathrm{30}}=\mathrm{6}°\:\:\: \\ $$$$\bigstar\bigstar\:\:\boldsymbol{{cos}}\mathrm{72}=\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\:\:\:\:\boldsymbol{{cos}}\mathrm{72}=\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{4}} \\ $$
